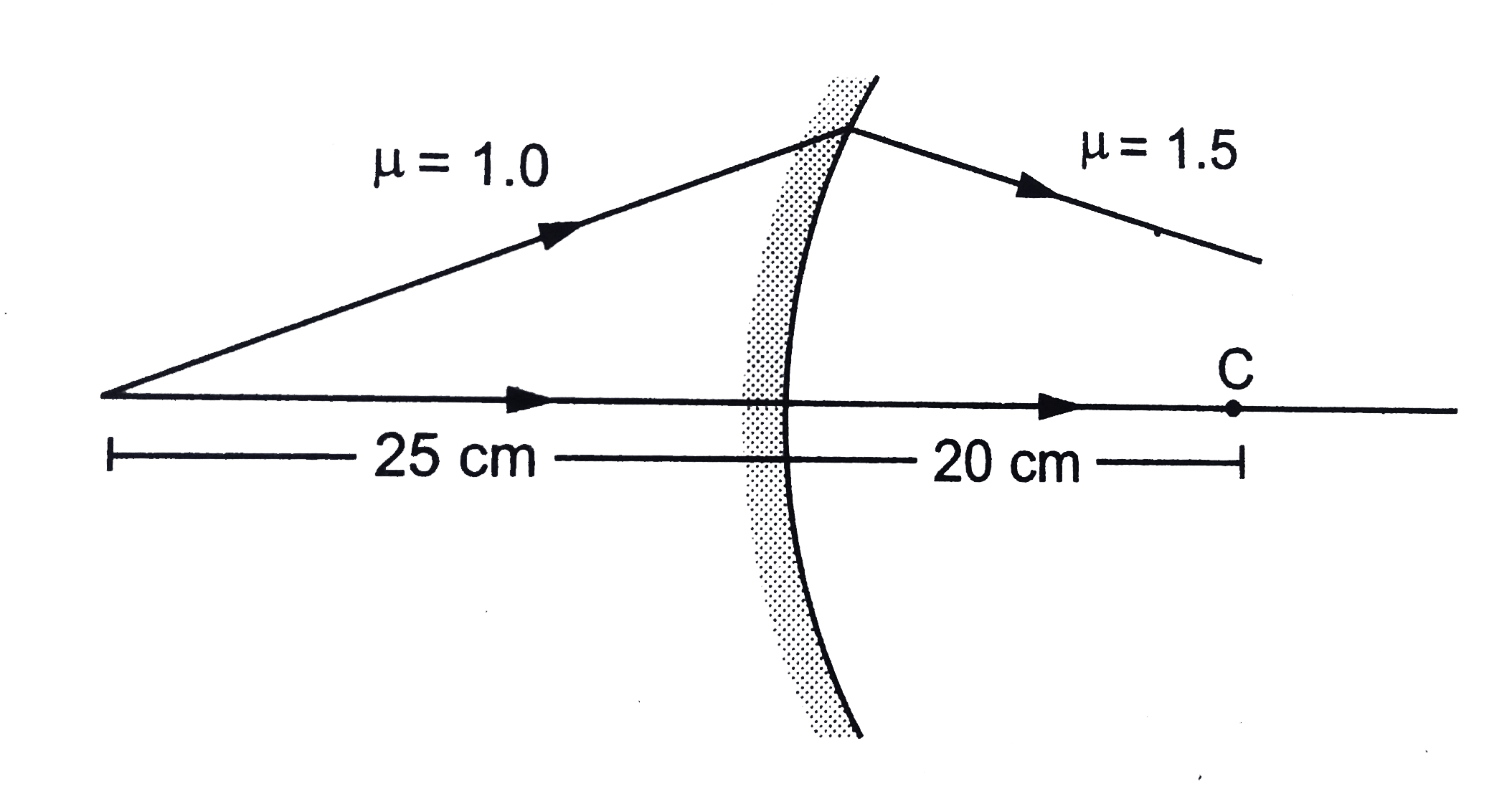
Locate the image of the point object O in the situation shown. The point C denotes the centre of curvature of the separating surface.
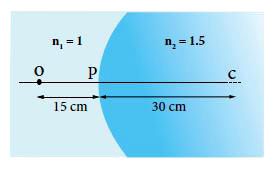
Given, cm, R=30 cm and
Equation for single spherical surface is,
Substituting the values,
cm
The image is a virtual image formed 30 cm to the left of the spherical surface.
Topper's Solved these Questions
- OPTICSBOOK - NCERT TAMILCHAPTER - OPTICSEXERCISE - EVALUATION (Multiple choice questions)
20 Videos
- OPTICSBOOK - NCERT TAMILCHAPTER - OPTICSEXERCISE - EVALUATION (Numerical Problems)
10 Videos
- NUCLEIBOOK - NCERT TAMILCHAPTER - NUCLEIEXERCISE - ADDITIONAL EXERCISES
9 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - NCERT TAMILCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - EXERCISES
40 Videos
Similar Questions
Knowledge Check
In the figure shown , a point object O is placed in air on the principal axis. The radius of curvature of the spherical surfaces is 60cm. I is the final image formed after all reflections and refractions.
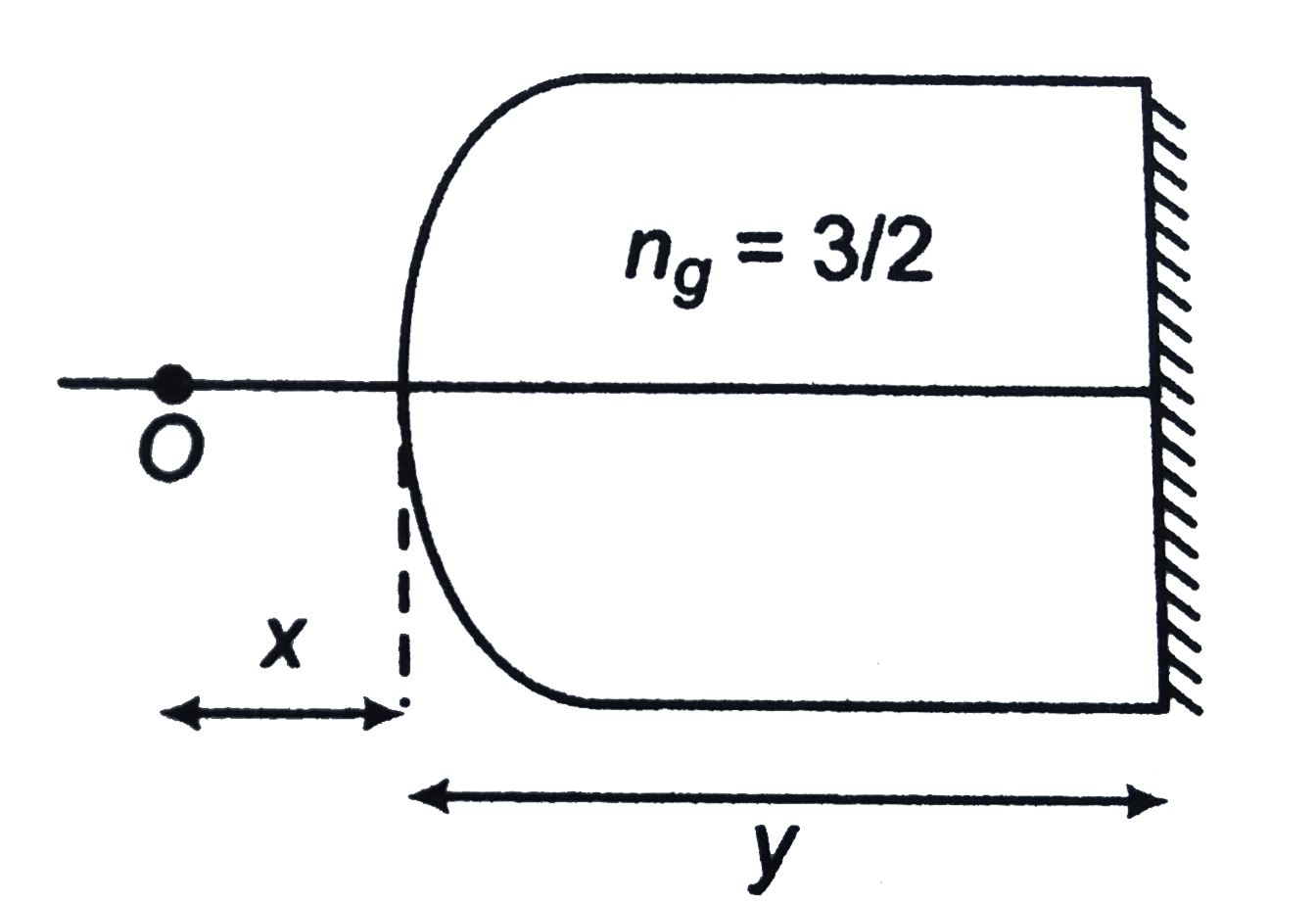 AIf x=120cm, then I is formed on O for any value of yBIf x=240cm, then I is formed on O for any value of y=360cmCIf x=240cm, then I is formed on O for any value of yDNone of these
AIf x=120cm, then I is formed on O for any value of yBIf x=240cm, then I is formed on O for any value of y=360cmCIf x=240cm, then I is formed on O for any value of yDNone of these
Locate the image formed by refraction in the situation shown in figure
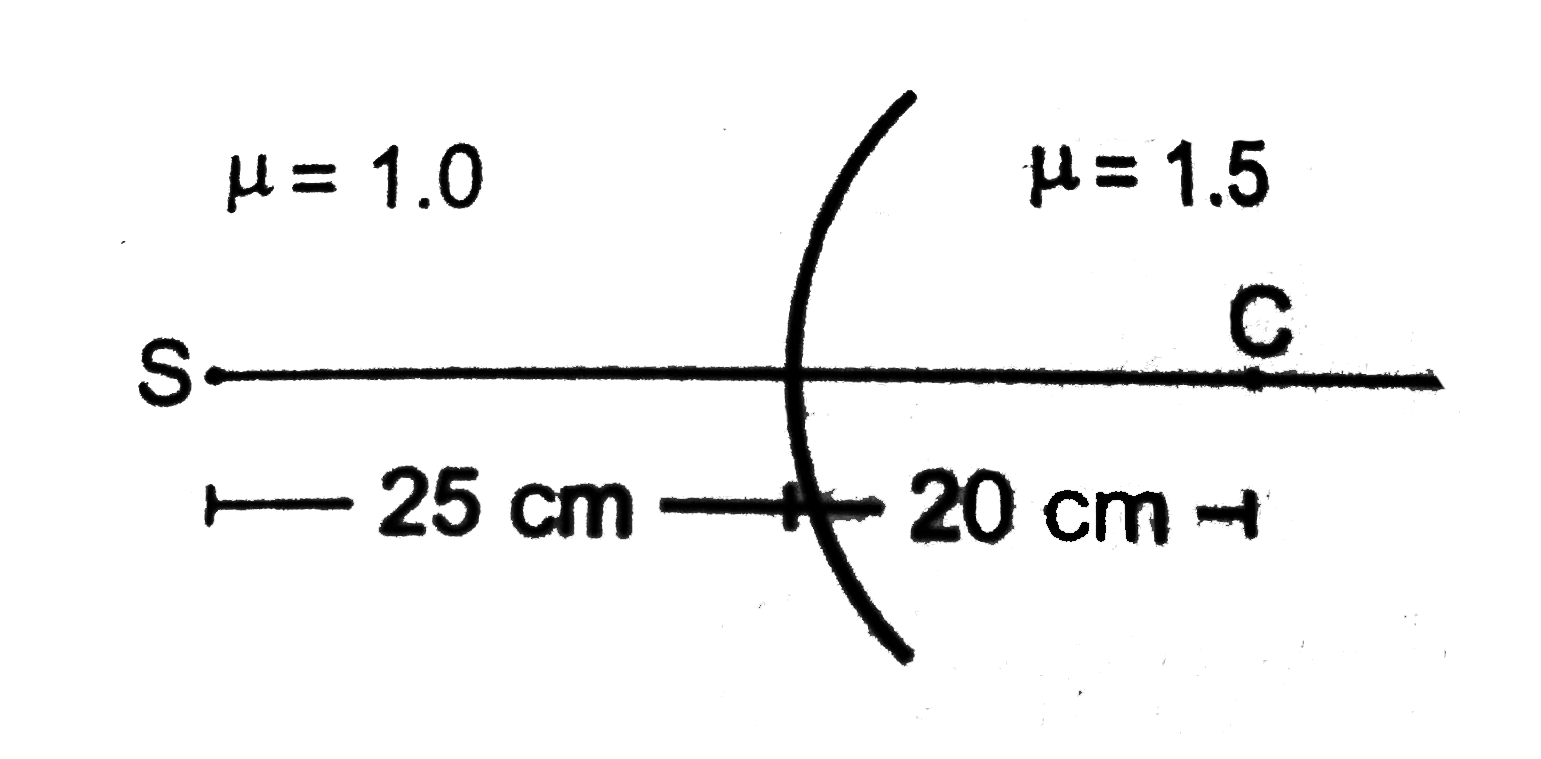
View SolutionLocate the image formed by refraction in the situation shown in figure

View Solution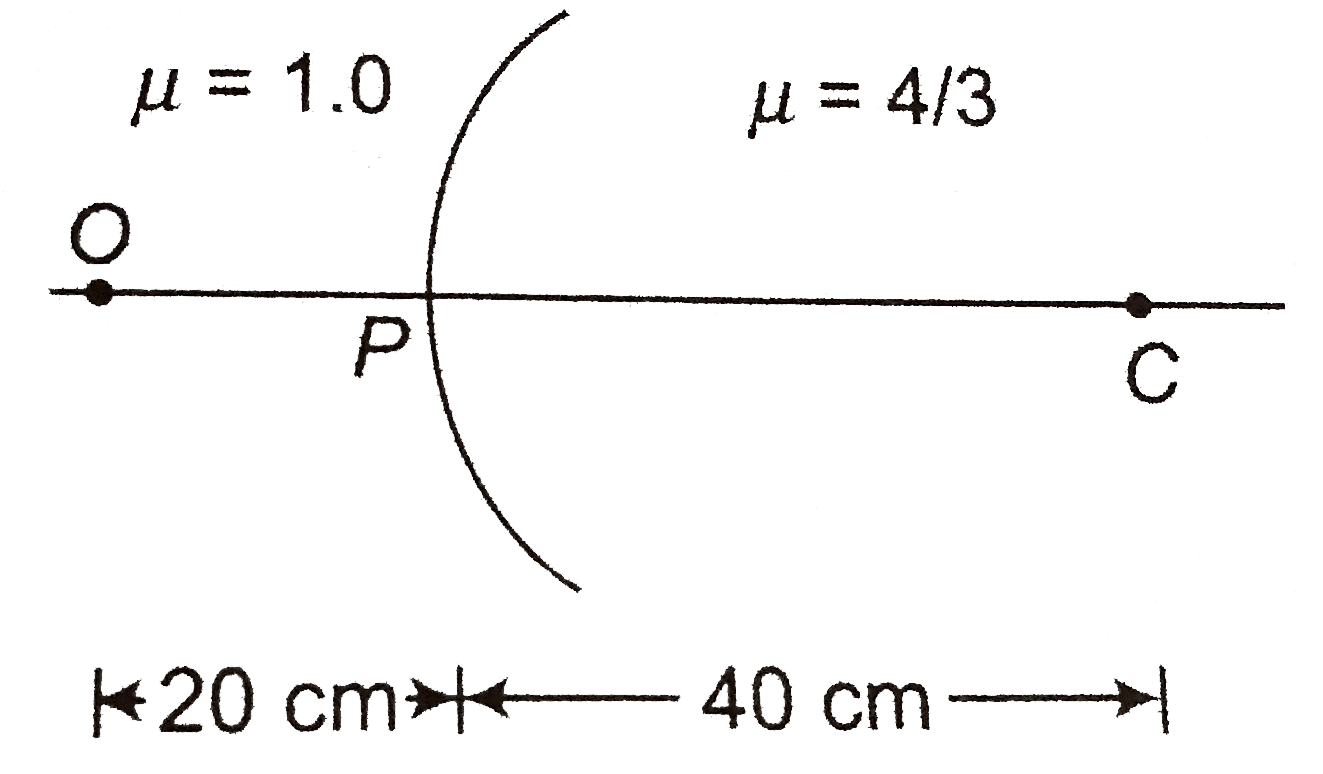
Locate the image of the point object O. The point C is centre of curvature of the spherical surface.
View Solution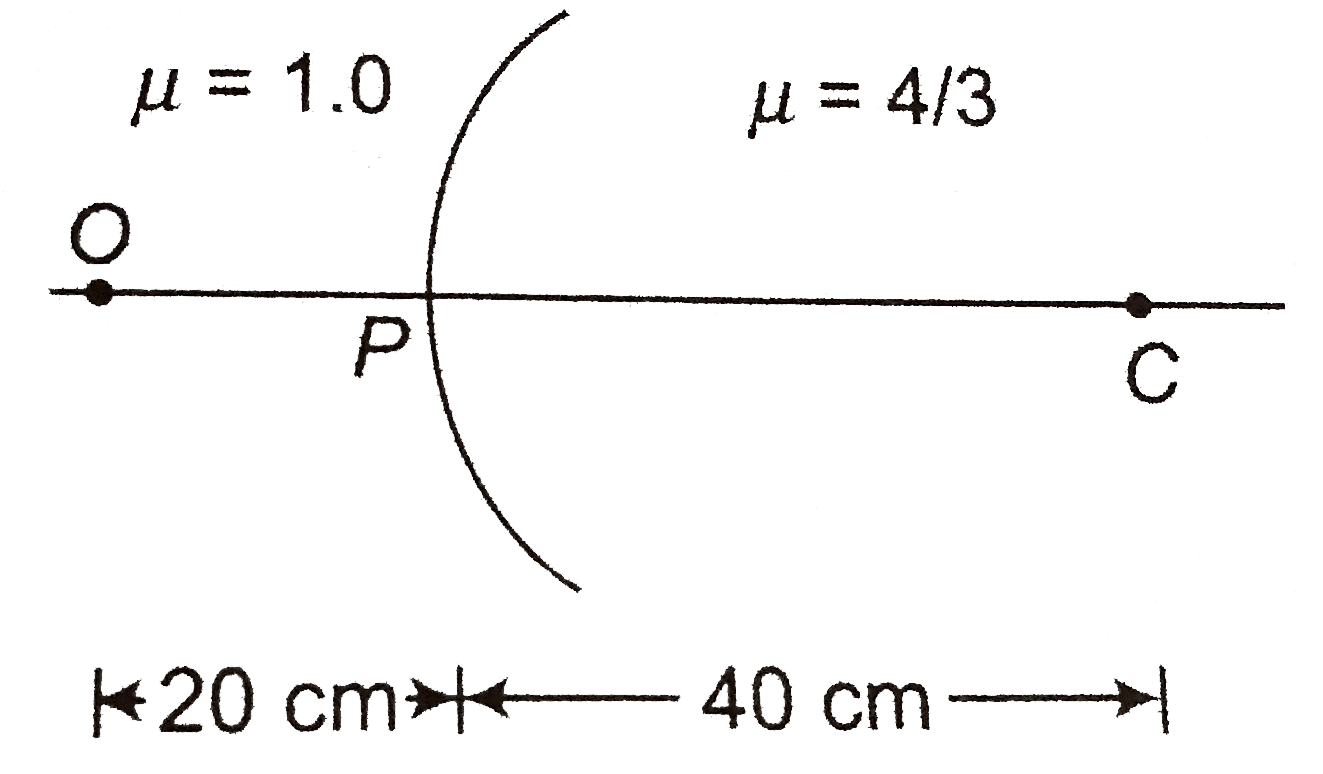
Locate the image of the point object O. The point C is centre of curvature of the spherical surface.
View SolutionIn the figure shown , a point object O is placed in air on the principal axis. The radius of curvature of the spherical surfaces is 60cm. I is the final image formed after all reflections and refractions.

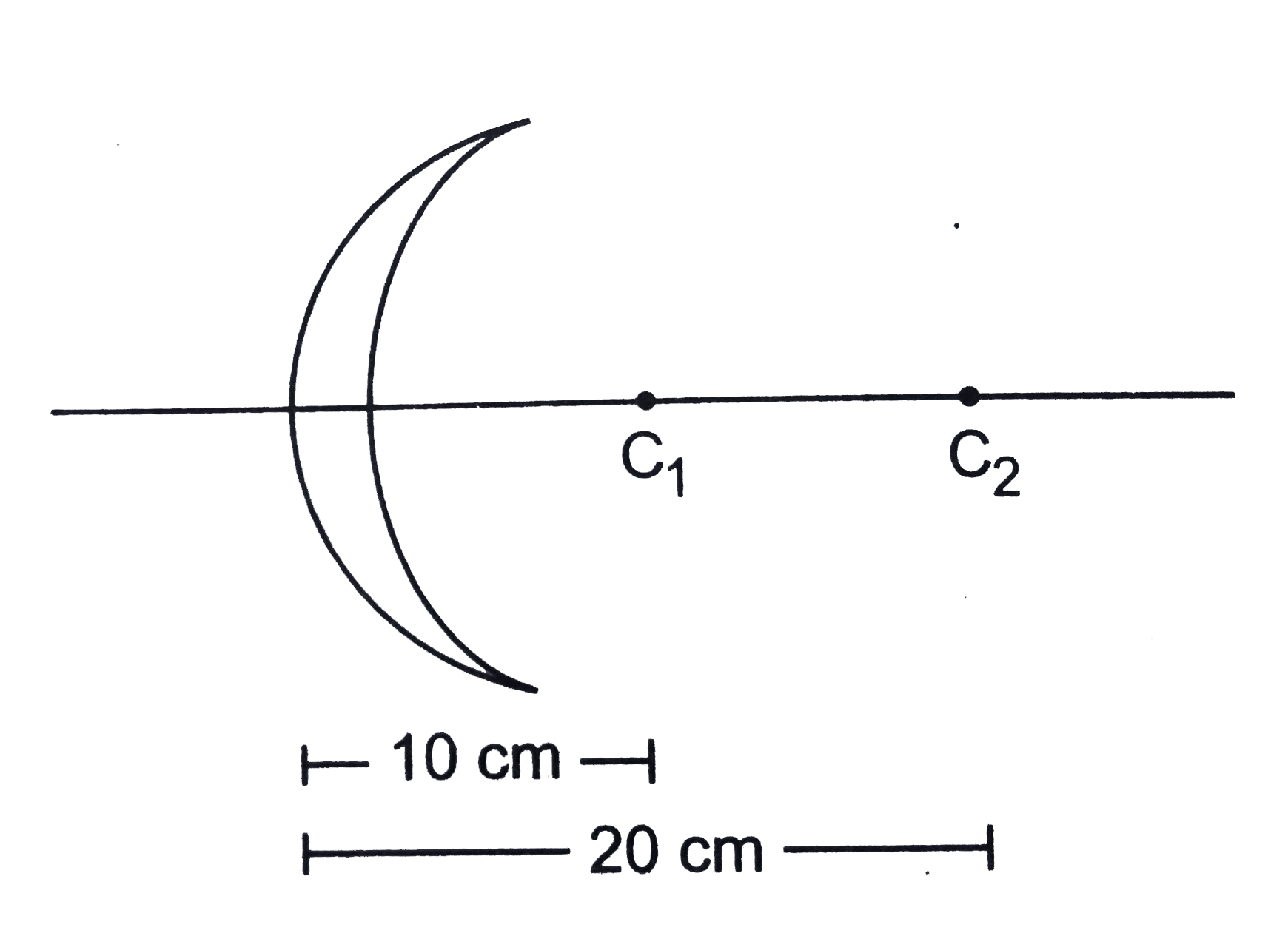
View SolutionCalculate the focal length of the thin lens shown infigure. The pionts C1andC2 denote the centres of curvature.
View Solution(a) A point object, O is on the principle axis of a spherical surface having a radius of curvature, R. Draw a diagram to obtain the relation between the object and image distances, the refractive indicess of the media and the radius of curvature of the spherical surface.
View Solution