The wavelength of a light is 450 nm. How much phase it will differ for a path of 3 mm?
The wavelength is λ=450 mm=450×10−9m
Path difference is, δ=3 mm=3×10−3m
Relation between phase difference and path difference is, ϕ=2πλ×δ
Substituting,
ϕ=2π450×10−9×3×10−3=π75×106
ϕ=π75×106 rad
Topper's Solved these Questions
- OPTICSBOOK - NCERT TAMILCHAPTER - OPTICSEXERCISE - EVALUATION (Multiple choice questions)
20 Videos
- OPTICSBOOK - NCERT TAMILCHAPTER - OPTICSEXERCISE - EVALUATION (Numerical Problems)
10 Videos
- NUCLEIBOOK - NCERT TAMILCHAPTER - NUCLEIEXERCISE - ADDITIONAL EXERCISES
9 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - NCERT TAMILCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - EXERCISES
40 Videos
Similar Questions
In a photoelectric effect experiment the threshold wavelength of light is 380 nm. If the wavelength of incident light is 260 nm, the maximum kinetic energy of emitted electrons will be ________eV
Given E (in eV) = 1237λ(in mm)
View SolutionIn a standard YDSE slit width is 1mm and distance of screen from the slit is 1m. If wavelength of light is 632nm and bright fringe formed at y = 1.270 mm. Find path difference for the point
View Solution
Knowledge Check
In standard Young's double-slit experiment, 9 fringes are found to be formed in a portion of screen. Wavelength of light used is 600 nm. How many fringes will be formed in the same portion of screen if wavelength of light is changed to 450 nm?
A12B18C14D20In a standard YDSE slit width is 1mm and distance of screen from the slit is 1m. If wavelength of light is 632nm and bright fringe formed at y = 1.270 mm. Find path difference for the point
A1.27 micromB2.45 micromC0.27 micromD2.27 micromIn YDSE wavelength of light used is 500 nm and slit width is 0.05mm. then the angular fringe width will be.
A1.8∘B3.2∘C0.57∘D0.48∘
In YDSE wavelength of light used is 600nm. At a point intensity is 50% of maximum value. What is path difference (in nm ) 'at that point.
View SolutionIn YDSE wavelength of light used is 500 nm and slit width is 0.05mm. then the angular fringe width will be.
View SolutionThe width of an aperture is 4 mm and wavelength of light illuminating it is 600 nm. Find the distance up to which ray optics is valid.
View SolutionA ray of light of frequency 500 terahertz is passed through a liquid. If the wavelength of light measured inside the liquid is 450 nm, calculate the refractive index of the liquid.
View SolutionFigure shows two light rays that are initially exactly in phase and that reflect from several glass surfaces. Neglect the slight slant in the path of the light in the second arrangement. The path length difference in terms of wavelength λ is :
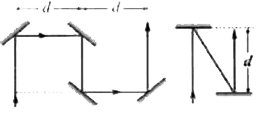
View Solution
