From a point Q, 13 cm away from the centre of a circle, the length of tangent PQ to the circle is 12 cm. What will be it the radius of the circle (in cm)?
The correct Answer is:5cm
To find the radius of the circle given the distance from point Q to the center O of the circle and the length of the tangent PQ, we can use the Pythagorean theorem. Here’s the step-by-step solution:
Step 1: Identify the Given Information
- Distance from point Q to the center O of the circle (QO) = 13 cm
- Length of the tangent PQ = 12 cm
Step 2: Understand the Geometry
In the right triangle formed by points P, Q, and O:
- OQ is the hypotenuse.
- PQ is one leg (the tangent).
- PO is the other leg (the radius of the circle).
Step 3: Apply the Pythagorean Theorem
According to the Pythagorean theorem:
QO2=PQ2+PO2
Substituting the known values:
132=122+PO2
Step 4: Calculate the Squares
Calculate 132 and 122:
169=144+PO2
Step 5: Solve for PO^2
Rearranging the equation to find PO2:
PO2=169−144
PO2=25
Step 6: Find PO (the Radius)
Taking the square root of both sides to find PO:
PO=√25=5 cm
Conclusion
The radius of the circle is 5 cm.
---
- Distance from point Q to the center O of the circle (QO) = 13 cm
- Length of the tangent PQ = 12 cm
In the right triangle formed by points P, Q, and O:
- OQ is the hypotenuse.
- PQ is one leg (the tangent).
- PO is the other leg (the radius of the circle).
According to the Pythagorean theorem:
QO2=PQ2+PO2
Substituting the known values:
132=122+PO2
Calculate 132 and 122:
169=144+PO2
Rearranging the equation to find PO2:
PO2=169−144
PO2=25
Taking the square root of both sides to find PO:
PO=√25=5 cm
The radius of the circle is 5 cm.
Topper's Solved these Questions
- SAMPLE PAPER 5BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE PAPER 5EXERCISE - Section- B
3 Videos
- SAMPLE PAPER 5BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE PAPER 5EXERCISE - Section -C
2 Videos
- SAMPLE PAPER 5BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE PAPER 5EXERCISE - Section -C
2 Videos
- SAMPLE PAPER 4BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE PAPER 4EXERCISE - Section -C
8 Videos
- SAMPLE PAPER 6BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE PAPER 6EXERCISE - Section -C
7 Videos
Similar Questions
From a point Q, 13 cm away from the centre of a circle, the length of tangent PQ to the circle is 12 cm. The radius of the circle (in cm) is
View SolutionA point P is 13cm from the centre of the circle. The length of the tangent drawn from P to the circle is 12 cm. Find the radius of the circle.
View Solution
Knowledge Check
A point P is 25 cm from the centre of the circle. The length of the tangent drawn from P to the circle is 24 cm. Find the radius of the circle.
A5 cmB7 cmC6 cmD8 cmPoint A is situated at a distance of 6.5 cm from the centre of a circle. The length of tangent drawn from point A to the circle is 6 cm. What is the radius of the circle?
AA)5 cmBB)4 cmCC)3.5 cmDD)2.5 cmA point Q is 13 cm from the centre of a circle . The lenght of the tangent drawn from Q to a circle is 12 cm. The distance of Q from the nearest point of the circle is
A7 cmB8 cmC5 cmD12 cm
A point is 26 cm away from the centre O of a circle and the length PT of the tangent drawn from P to the circle is 10 cm. Find the radius of the circle.
View SolutionA point P is 25cm away from the centre of a circle and the lens of tangent drawn from P to the circle is 24cm. Find the radius.
View SolutionType I: A point P is 13cm from the center of the circle. The length of the tangent drawn from P to the circle is 12 cm . Find the radius of the circle ?
View SolutionFrom a point P, 10cm away from the centre of a circle, a tangent PT of length 8cm is drawn. Find the radius of the circle.
View Solution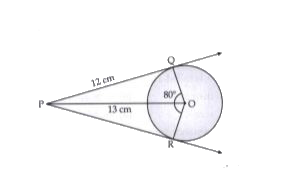
A point P is 13 cm from the centre of the circle. The two tangent PQ and PR are drawn from the point P, The length of the tangent drawn from P to the circle is 12 cm.
Find the radius of the circle.
View Solution
