The five sides of a regular pentagon are represented by vectors ${\vec{A}}_{1}, {\vec{A}}_{2}, {\vec{A}}_{3}, {\vec{A}}_{4}$ and ${\vec{A}}_{5}$ in cyclic order as shown. Corresponding vertices are represented by ${\vec{B}}_{1}, {\vec{B}}_{2}, {\vec{B}}_{3}, {\vec{B}}_{4} and {\vec{B}}_{5}$ drawn from the centre of the pentagon. Then, ${\vec{B}}_{2} + {\vec{B}}_{3} + {\vec{B}}_{4} + {\vec{B}}_{5}$ =

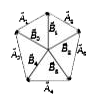
A

${\vec{A}}_{1}$

B

$- {\vec{A}}_{1}$

C

${\vec{B}}_{1}$

D

$- {\vec{B}}_{1}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Since, ${\vec{B}}_{1} + {\vec{B}}_{2} + {\vec{B}}_{3} + {\vec{B}}_{4} + {\vec{B}}_{5} = 0$
This is because of regular pentagon Hence, ${\vec{B}}_{2} + {\vec{B}}_{3} + {\vec{B}}_{4} + {\vec{B}}_{5} = - {\vec{B}}_{1}$

|

Updated on:21/07/2023

Class 11 PHYSICS BASIC MATHEMATICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If $| \to a | = 3, ∣ ∣ \to b ∣ ∣ = 4 and ∣ ∣ \to a - \to b ∣ ∣ = 5$ , then what is the value of $∣ ∣ \to a + \to b ∣ ∣ = ?$
A8
B6
C $5 \sqrt{2}$
D5
Question 2 - Select One
If $| \to a | = 3, ∣ ∣ \to b ∣ ∣ = 4 and ∣ ∣ \to a - \to b ∣ ∣ = 5$ , then what si the value of $∣ ∣ \to a + \to b ∣ ∣$ =?
A8
B6
C $5 \sqrt{2}$
D5
Question 3 - Select One
Given that $\to A + \to B = \to C$ . If $∣ ∣ \to A ∣ ∣ = 4, ∣ ∣ \to B ∣ ∣ = 5$ and $∣ ∣ \to C ∣ ∣ = \sqrt{61}$ , the angle between $\to A$ and $\to B$ is
A $30^{\circ}$
B $60^{\circ}$
C $90^{\circ}$
D $120^{\circ}$

ERRORLESS-BASIC MATHEMATICS AND VECTORS-NCERT Based Questions (Addition and Subtraction of Vectors )

Home
Ask Doubt
Profile