Assertion: In y=Asin(ωt−kx),(ωt−kx) is dimensionless.
Reason: Because dimension of ω=[M0L0T].
If both assertion and reason are true and the reason is the correct explanation of the assertion.
If both assertion and reason are true but reason is not the correct explanation of the assertion
If assertion is true but reason is false.
If the assertion and reason both are false
The correct Answer is:C
y=Asin(ωt−kx)
[ωt]=[kx]=[M0L0T0]→ dimensionless
[ω]=[T−1]
Topper's Solved these Questions
- BOOK - ERRORLESSCHAPTER - UNITS, DIMENSIONS AND MEASUREMENTEXERCISE - PAST YEARS QUESTIONS24videos
UNITS, DIMENSIONS AND MEASUREMENT
- BOOK - ERRORLESSCHAPTER - TRANSMISSION OF HEAT EXERCISE - Assertion & Reason22videos
TRANSMISSION OF HEAT
- BOOK - ERRORLESSCHAPTER - WAVES AND SOUND EXERCISE - ASSERTION & REASON25videos
WAVES AND SOUND
Similar Questions
Knowledge Check
- Question 1 - Select One
In the relation y=rsin(ωt−kx), the dimensions of ω/k are
A[M0L0T0]B[M0L1T−1]C[M0L0T1]D[M0L1T0] - Question 2 - Select One
if y=Asin(ωt−kx), then the value of dydx is
AAcos(ωt−kx)B−Aωcos(ωt−kx)CAKcos(ωt−kx)D−AKcos(ωt−kx)
(a) the displacement of a particle is given by s−asin(ωt=kx), where t is in second and x is in meter. Find the dimensions of ω and k.
(b) The velocity of a praticle is given by v=v0e−λt, where t is time. Find the dimensions of v0 and λ.View SolutionIn the expression y=asin(ωt+θ), y is the displacement and t is the time . Write the dimension ofa,ωand θ .
View SolutionIn y= A sin ωt + A sin (ωt+(2π)/3) match the following table.
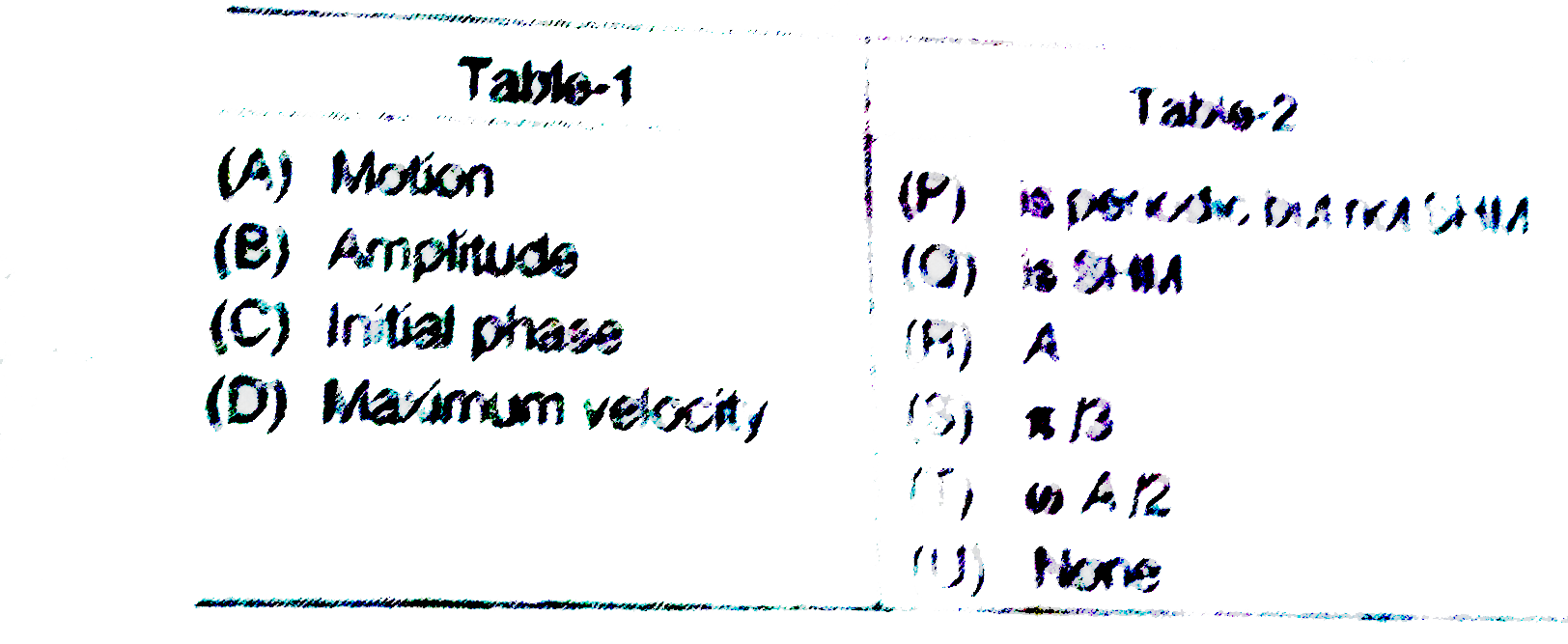 View Solution
View SolutionIn the equation y= a sin (ωt+kx) t and x stand for time and distance respectively. What are the dimensions of ω/k?
View SolutionIn the relation x=cos(ωt+kx), the dimension(s) of ω is/are
View SolutionIn the equation y=Asin(ωt−kx), obtain the dimensional formula of ωandk. Given x is distnace and t is time.
View Solution

