An electron and a proton are moving under the influence of mutual forces. In calculating the change in the kinetic energy of the system during motion, one ignores the magnetic force of one on another. This is because,
The two magnetic forces are equal and opposite, so they produce no net effect
The magnetic forces do not work on each particle
The magnetic forces do equal and opposite (but nonzero) work on each particle
The magnetic forces are necessarily negligible
The correct Answer is:B
When electron and proton are moving under influence of their mutual forces, the magnetic forces will be perpendicular to their motion hence no work is done by these forces.
Topper's Solved these Questions
- BOOK - ERRORLESSCHAPTER - WORK, ENERGY POWER AND COLLISIONEXERCISE - NCERT BASED QUESTIONS (Power)18videos
WORK, ENERGY POWER AND COLLISION
- BOOK - ERRORLESSCHAPTER - WORK, ENERGY POWER AND COLLISIONEXERCISE - NCERT BASED QUESTIONS (Elastic and Inelastic Collision)44videos
WORK, ENERGY POWER AND COLLISION
- BOOK - ERRORLESSCHAPTER - WORK, ENERGY POWER AND COLLISIONEXERCISE - NCERT BASED QUESTIONS (Work Done by Variable Force)26videos
WORK, ENERGY POWER AND COLLISION
- BOOK - ERRORLESSCHAPTER - WAVES AND SOUND EXERCISE - ASSERTION & REASON25videos
WAVES AND SOUND
Similar Questions
Knowledge Check
- Question 1 - Select One
Assertion: Internal forces cannot change linear momentum.
Reason: Internal forces can change the kinetic energy of a system.A(a) If both Assertion and Reason are true and the Reason is correct explanation of the Assertion.B(b) If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.C(c) If Assertion is true, but the Reason is false.D(d) If Assertion is false but the Reason is true. - Question 2 - Select One
A : Internal forces can change the kinetic energy but not the momentum of the system.
R : The net internal force on a system is always zero.AIf both Assertion & Reason are true and the reason is the correct explanation of the assertion, then mark (1).BIf both Assertion & Reason are true but the reason is not the correct explanation of the assertion, then mark (2).CIf Assertion is true statement but Reason is false, then mark (3).DIf both Assertion and Reason are falsė statements, then mark (4). - Question 3 - Select One
A particle is moving under the influence of a force which is fixed in magnitude and acting at an angle θ in the direction of motion. The path described by the particle is
Aa circleBan ellipseCa parabolaDa straight line
An electron is either released from rest or projected with some initial velocity in a uniform electric field. Neglect any other force on the electron apart from electrostatic force. Which of the graphs shown in fig could possibly represent the change in kinetic energy of the electron during its course of motion? Explain the situation in each case.
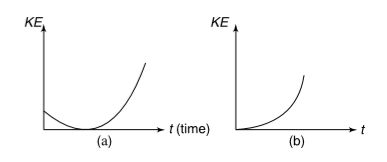
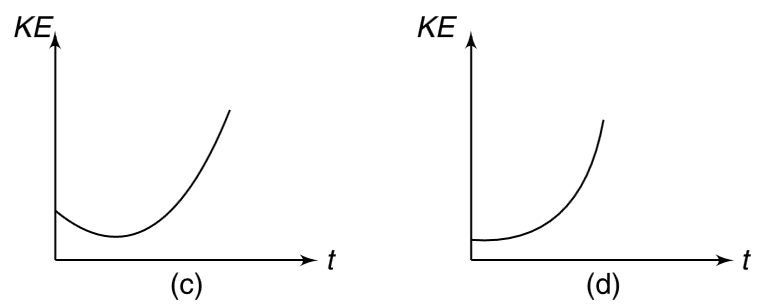 View Solution
View SolutionAn electron and a proton are moving with the same kinetic energy along the same direction, When they pass through a uniform magnetic field perpendicular to the direction of their motion, they describe circular path of the same radius. Is this statement true or false?
View SolutionAn electron and a proton are moving with the same kinetic energy along the same direction . When they pass through a uniform magnetic field perpendicular to the direction of their motion , they describe circular paths of the same radius.
View SolutionAssertion : Internal forces acting on the system cannot change linear momentum of the system .
Reason : Internal forces can change kinetic energy of the system.View SolutionConsider the following statements
I. The rate of change of the total linear momentum of a system consisting of many particles is proportional to the vector sum of all the internal forces due to inter-particle interaction.
The internal forces can change the kinetic energy of the system of particles are not the linear momentum of the system. Choose the correct option.View Solution

