A train accelerates from rest at a constant rate a for distance $x_{1}$ and time $t_{91})$ . After that is retards at constant rate $β$ for distance $x_{2}$ and time $t_{2}$ and comes to the rest. Which of the following relation is correct:-

A

$\frac{x_{1}}{x_{2}} = \frac{α}{β} = \frac{t_{1}}{t_{2}}$

B

$\frac{x_{1}}{x_{2}} = \frac{β}{α} = \frac{t_{1}}{t_{2}}$

C

$\frac{x_{1}}{x_{2}} = \frac{α}{β} = \frac{t_{2}}{t_{1}}$

D

$\frac{x_{1}}{x_{2}} = \frac{β}{α} = \frac{t_{2}}{t_{1}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

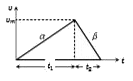
$t_{1} = \frac{v_{m}}{α},$ t_2 = (v_m)/(beta) $< b r >$ x_1 = 1/2 v_m t_1 $< b r >$ x_2 = 1/2 v_m t_2 $< b r >$ x_1/x_2 = (t_1)/(t_2) = beta/alpha

|

Updated on:21/07/2023

Class 11 PHYSICS RECTILINEAR MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A train accelerates from rest at a constant rate for distance $\X1$ and time . $\t1$ After that retards to rst at constant rate for distance $X_{2}$ and time $t_{2}$ . Then, it is found that
A $\frac{X_{1}}{X_{2}} = \frac{α}{β} = \frac{t_{1}}{t_{2}}$
B $\frac{X_{1}}{X_{2}} = \frac{α}{β} = \frac{t_{2}}{t_{1}}$
C $\frac{X_{1}}{X_{2}} = \frac{β}{α} = \frac{t_{1}}{t_{2}}$
D $\frac{X_{1}}{X_{2}} = \frac{β}{α} = \frac{t_{2}}{t_{1}}$
Question 2 - Select One or More
A train moves from rest with acceleration $α$ and in time $t_{1}$ covers a distance x. It then decelerates rest at constant retardation $β$ for distance y in time $t_{2}$ . Then
A $\frac{x}{y} = \frac{β}{α}$
B $\frac{β}{α} = \frac{t_{1}}{t_{2}}$
Cx=y
D $\frac{x}{y} = \frac{β t_{1}}{α t_{2}}$
Question 3 - Select One
A scooter accelerates from rest for time $t_{1}$ at constant rate $a_{1}$ and then retards at constant rate $a_{2}$ for time $t_{2}$ and comes to rest. The correct value of $\frac{t_{1}}{t_{2}}$ will be :-
A $\frac{a_{1} + a_{2}}{a_{2}}$
B $\frac{a_{2}}{a_{1}}$
C $\frac{a_{1}}{a_{2}}$
D $\frac{a_{1} + a_{2}}{a_{1}}$

ERRORLESS-MOTION IN ONE DIMENSION-NCERT BASED QUESTIONS (NON-UNIFORM MOTION )

Home
Ask Doubt
Profile