A mass m is suspended from the two coupled springs connected in series. The force constant for springs are k1andk2. The time period of the suspended mass will be
T=2π√(mK1+K2)
T=2π√(2mK1+K2)
T=2π ⎷(m(K1+K2)K1K2)
T=2π√(mK1K2K1+K2)
The correct Answer is:C
keq=K1K2K1+K2 [as spring connected in series]
T=2π√m(K1+K2)K1K2.
Topper's Solved these Questions
- BOOK - ERRORLESSCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - NCERT BASED QUESTIONS (Superposition of S.H.M.s and Resonance)7videos
SIMPLE HARMONIC MOTION
- BOOK - ERRORLESSCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - PAST YEARS QUESTIONS28videos
SIMPLE HARMONIC MOTION
- BOOK - ERRORLESSCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - NCERT BASED QUESTIONS (Simple Pendulum)27videos
SIMPLE HARMONIC MOTION
- BOOK - ERRORLESSCHAPTER - ROTATIONAL MOTIONEXERCISE - Assertion & Reason25videos
ROTATIONAL MOTION
- BOOK - ERRORLESSCHAPTER - SURFACE TENSIONEXERCISE - ASSERTION & REASON11videos
SURFACE TENSION
Similar Questions
Two springs are joined and attached to a mass of 16 kg. The system is then suspended vertically from a rigid support. The spring constant of the two springs are K1 and K2 respectively. The period of vertical oscillations of the system will be:
View SolutionWhen a body is suspended from two light springs separately, the periods of vertical oscillations are T1 and T2. When the same body is suspended from the two spring connected in series, the period will be
View Solution
Knowledge Check
- Question 1 - Select One
A mass m is suspended from the two coupled springs connected in series. The force constant for spring are k1 and k2. The time period of the suspended mass will be:
AT=2π√mk1−k2BT=2π√mk1k2k1+k2CT=2π√mk1+k2DT=2π√m(k1+k2)k1k2 - Question 2 - Select One
A mass m is suspended by means of two coiled springs which have the same length in unstretched condition as shown in figure. Their force constants are k1andk2 respectively. When set into vertical vibrations, the period will be
 A2π√(mk1k2)B2π√m(k1k2)C2π√(mk1−k2)D2π√(mk1+k2)
A2π√(mk1k2)B2π√m(k1k2)C2π√(mk1−k2)D2π√(mk1+k2) - Question 3 - Select One
A mass m is suspended separately by two different springs of spring constant k1 and k2 given the time period t1 and t2 respectively. If the same mass m is shown in the figure then time period t is given by the relation
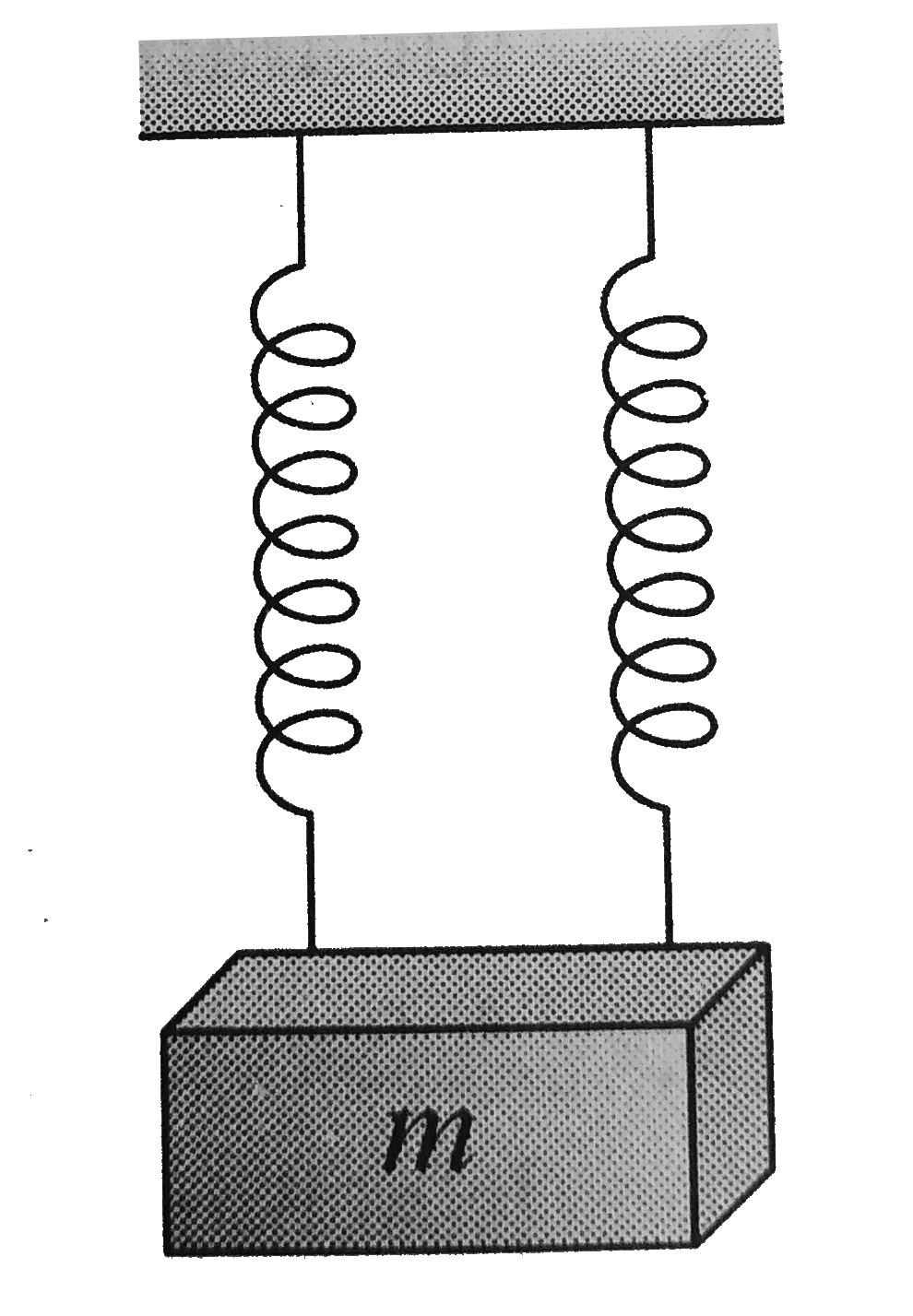 At=t1+t2Bt=t1t2t1+t2Ct2=t21+t22Dt−2=t−21+t−22
At=t1+t2Bt=t1t2t1+t2Ct2=t21+t22Dt−2=t−21+t−22
A mass m is suspended by means of two coiled spring which have the same length in unstretched condition as in figure. Their force constant are k 1 and k 2 respectively. When set into vertical vibrations, the period will be
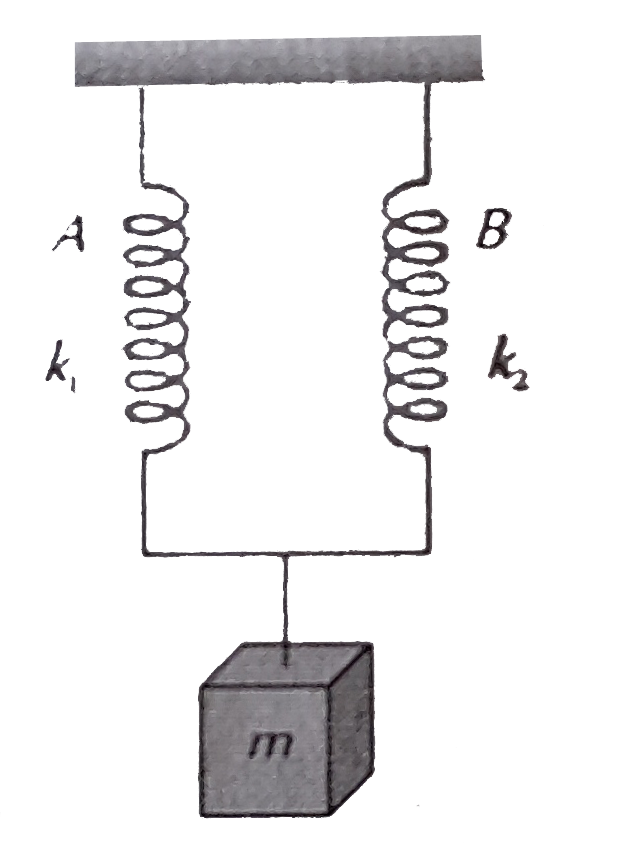 View Solution
View SolutionWhen a body is suspended from two light springs separately, the periods of vertical oscillations are T1 and T2. When the same body is suspended from the two spring connected in series, the period will be
View SolutionA mass is suspended separately by two springs of spring constants k1 and k2 in successive order. The time periods of oscillations in the two cases are T1 and T2 respectively. If the same mass be suspended by connecting the two springs in parallel, (as shown in figure) then the time period of oscillations is T. The correct relations is
 View Solution
View SolutionTwo springs are joined and attached to a mass of 16 kg. The system is then suspended vertically from a rigid support. The spring constant of the two spring are k1andk2 respectively. The period of vertical oscillations of the system will be
View SolutionLet T1 and T2 be the time periods of two springs A and B when a mass m is suspended from them separately. Now both the springs are connected in parallel and same mass m is suspended with them. Now let T be the time period in this position. Then
View Solution

