Two waves are propagating to the point P along a straight line produced by two sources A and B of simple harmonic and of equal frequency. The amplitude of every wave at P is $a$ and the phase of A is ahead by $π / 3$ than that of B and the distance AP is greater than BP by $50 c m$ . Then the resultant amplitude at the point P will be if the wavelength is $1$ meter

A

2a

B

$a \sqrt{3}$

C

$a \sqrt{2}$

D

a

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

$Δ x$ = path difference = 50 cm = $\frac{1}{2}$ m
$Δ ϕ = \frac{2 π}{λ} \times Δ ξ m p l i e s Δ ϕ = \frac{2 π}{1} \times \frac{1}{2} = π$
Total phase difference $= π - \frac{π}{3} = \frac{2 π}{3}$
Resultant amplitude $A = \sqrt{a^{2} + a^{2} + 2 a^{2} cos (\frac{2 π}{3})} = a$

|

Updated on:21/07/2023

Class 11 PHYSICS SOUND WAVES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two waves are propagating to the point P along a straight line produced by two sources A and B of simple harmonic and of equal frequency. The amplitude of every wave at P is $a$ and the phase of A is ahead by $π / 3$ than that of B and the distance AP is greater than BP by $50 c m$ . Then the resultant amplitude at the point P will be if the wavelength is $1$ meter
A $2 a$
B $a \sqrt{3}$
C $a \sqrt{2}$
D $a$
Question 2 - Select One
The resultant of two rectangular simple harmonic motion of the same frequency and unequal amplitude but differing in phase by $π / 2$ is
ASimple harmonic
BCircular
CElliptical
DParabolic
Question 3 - Select One
The mass M shown in figure ocillates in simple harmonic motion with amplitude A.
The amplitude of the point P is

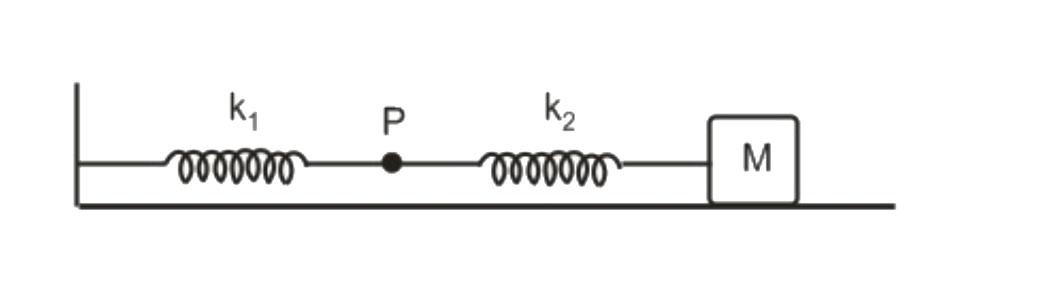A $\frac{k_{1} A}{k_{2}}$
B $\frac{k_{2} A}{k_{2} - k_{1}}$
C $\frac{k_{2} A}{k_{1} + k_{2}}$
D $\frac{k_{2} A}{k_{1}}$

ERRORLESS-WAVES AND SOUND -NCERT BASED QUESTIONS (INTERFERENCE AND SUPERPOSITION OF WAVES)

Home
Ask Doubt
Profile