Question

Statement I: In Young's double-slit experiment, the two slits are at distance d apart. Interference pattern is observed on a screen at distance D from the slits. At a point on the screen which is directly opposite to one of the slits, a dark fringe is observed. Then, the wavelength of wave is proportional to the squar of distance between two slits.
Statement II: For a dark fringe, intensity is zero

Options

A. If both assertion and reason are true and the reason is the correct explanation of the assertion
B. If both assertion and reason are true but reason is not the correct explanation of the assertion
C. If assertion is true but reason is false.
D. If the assertion and reason both are false

Answer

B

Explanation

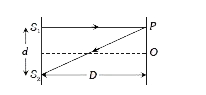
When dark fringe is obtained at the point opposite to one of the slits then
`S_(1)P= D` and `S_(2)P= sqrt(D^(2)+ d^(2))= D(1+(d^(2))/(D^(2)))^(1//2)= D(1+ (d^(2))/(2D^(2)))`
Path difference = `S_(2)P- S_(1)P`
`= D(1+ (d^(2))/(2D^(2)))-D=(d^(2))/(2D)= (lambda)/(2)`
`lambda= (d^(2))/(D) implies lambda prop d^(2)`
Now, intensity of a dark fringe is zero.