Find the magnitude of the resultant of shown forces :-

Video Solution

Text Solution

Verified by Experts

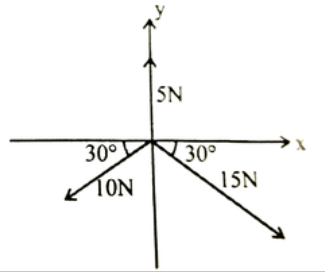
The correct Answer is:C

Answer

Step by step video, text & image solution for Find the magnitude of the resultant of shown forces :- by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 CHEMISTRY GENERAL PRINCIPLES AND PROCESSES OF ISOLATION OF ELEMENTS

Topper's Solved these Questions

BOOK - ERRORLESSCHAPTER - GENERAL PRINCIPLES & PROCESSES OF ISOLATION OF ELEMENTSEXERCISE - PAST YEARS QUESTIONS
20videos
GENERAL PRINCIPLES & PROCESSES OF ISOLATION OF ELEMENTS
BOOK - ERRORLESSCHAPTER - GENERAL PRINCIPLES & PROCESSES OF ISOLATION OF ELEMENTSEXERCISE - ASSERTION & REASON
8videos
GENERAL PRINCIPLES & PROCESSES OF ISOLATION OF ELEMENTS
BOOK - ERRORLESSCHAPTER - GENERAL PRINCIPLES & PROCESSES OF ISOLATION OF ELEMENTSEXERCISE - NCERT BASED QUESTIONS (Reduction of Free Metal)
43videos
GENERAL PRINCIPLES & PROCESSES OF ISOLATION OF ELEMENTS
BOOK - ERRORLESSCHAPTER - ELECTROCHEMISTRYEXERCISE - Assertion & Reason
11videos
ELECTROCHEMISTRY
BOOK - ERRORLESSCHAPTER - HELOALKANES AND HALOARENESEXERCISE - Assertion & Reason
8videos
HELOALKANES AND HALOARENES

Knowledge Check

Question 1 - Select One
The greatest & the least magnitude of the resultant of two forces of constant magnitudes are P & Q respectively. If the forces act at an angle $2 α$ , then prove that the magnitude of their resultant is given by $\sqrt{P^{2} {cos}^{2} α + Q^{2} {sin}^{2} α}$ .
A $\sqrt{} (F^{2} {cos}^{2} α + G^{2} {sin}^{2} α)$
B $\sqrt{} (F^{2} {sin}^{2} α + G^{2} {cos}^{2} α)$
C $\sqrt{} (F^{2} + G^{2})$
D $\sqrt{} (F^{2} - G^{2})$
Question 2 - Select One
Two forces with equal magnitudes F act on a body and the magnitude of the resultant force is F /3. The angle between the two forces is
A ${cos}^{- 1} (- \frac{17}{18})$
B ${cos}^{- 1} (- \frac{1}{3})$
C ${cos}^{- 1} (- \frac{2}{3})$
D ${cos}^{- 1} (- \frac{8}{9})$
Question 3 - Select One
Two forces with equal magnitudes F act on a body and the magnitude of the resultant force is F /3. The angle between the two forces is
A ${cos}^{- 1} (- \frac{17}{18})$
B ${cos}^{- 1} (- \frac{1}{3})$
C ${cos}^{- 1} (\frac{2}{3})$
D ${cos}^{- 1} (\frac{8}{9})$

ERRORLESS-GENERAL PRINCIPLES & PROCESSES OF ISOLATION OF ELEMENTS-NCERT BASED QUESTIONS (Refining of Crude Metal)

Home
Ask Doubt
Profile

Find the magnitude of the resultant of shown forces :-

The correct Answer is:C

Topper's Solved these Questions

Similar Questions

Knowledge Check

The greatest & the least magnitude of the resultant of two forces of constant magnitudes are P & Q respectively. If the forces act at an angle 2α, then prove that the magnitude of their resultant is given by √P2cos2α+Q2sin2α.

Two forces with equal magnitudes F act on a body and the magnitude of the resultant force is F /3. The angle between the two forces is

Two forces with equal magnitudes F act on a body and the magnitude of the resultant force is F /3. The angle between the two forces is

ERRORLESS-GENERAL PRINCIPLES & PROCESSES OF ISOLATION OF ELEMENTS-NCERT BASED QUESTIONS (Refining of Crude Metal)

The greatest & the least magnitude of the resultant of two forces of constant magnitudes are P & Q respectively. If the forces act at an angle $2 α$ , then prove that the magnitude of their resultant is given by $\sqrt{P^{2} {cos}^{2} α + Q^{2} {sin}^{2} α}$ .