A car starts moving along a line, first with an acceleration a=5ms−2 starting from rest, then uniformly and finally decelerating at the same rate, comes to rest in the total time of 25 seconds (t), then average velocity during the time is equal to v=72 kmph. how long does the particle move uniformly ?
25 seconds
2.5 hours
1.5 hours
15 seconds
The correct Answer is:D
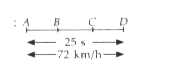
vavg=72km/h=20m/s
First part A to B: u=0,a=5m/s2,t=t1,s1=x1
s=ut+12at2⇒x1=0+125t21=52t21
v=u+at⇒v=0+5t1=5t10
For part B to C: u=5t1,t=t2,s2=x2
∴x2=5t1t2
For part C to D: u=5t1,t=t3,s2=x3,a=−5m/s2
x3=ut+12at2⇒x3=5t1t3−52t23
Average speed, vavg=s1+s2+s3t1+t2+t3
∴20=5t212+5t1t2+5t1t3−52t2325
As t1=t3andt1+t2+t3=25s
∴5t1t2+5t2125=20⇒t21+t1t2=100
(25−t22)2+(25−t22)t2=100 (∵t1=25−t22)
625−50t2+t224+25t2−t222=100
625−t22=400ort2=15s
The car will move uniformly for 15 s during whole journey.
Topper's Solved these Questions
- MOTIONBOOK - MTG IIT JEE FOUNDATIONCHAPTER - MOTIONEXERCISE - Exercise (Integer/Numerical Value Type)
5 Videos
- GRAVITATION BOOK - MTG IIT JEE FOUNDATIONCHAPTER - GRAVITATION EXERCISE - Olympiad/HOTS Corner
20 Videos
- SOUNDBOOK - MTG IIT JEE FOUNDATIONCHAPTER - SOUNDEXERCISE - Olympiad/HOTS Corner
15 Videos
Similar Questions
A car start moving along a line, fisrt with acceleration a=5ms−2 starting from rest then uniformly and finally decelerating at the same rate a, comes to rest. The total time of motion is π=25s. The average velocity during the time is equal to <v>=72kmhr. How long does the particle move uniformly ?
View SolutionA car starts moving along a line, first with acceleration a=5 ms−2 starting from rest then uniformly and finally decelerating at the same rate a, comes to rest.The total time of motion is τ=25s. The average velocity during the time is equal to lt v gt =72 km/hr.How long does the partial move uniformly ?
View Solution
Knowledge Check
A car accelerates from rest at a constant rate for some time after which it decelerates at a constant rate β to come to rest. If the total time elapsed is t, the maximum velocity acquired by the car is given by :
Aαβα+βtBα+βαβtCα2+β2βαDα+β2βα
A car starts moving along a line, first with acceleration a=5 ms−2 starting from rest then uniformly and finally decelerating at the same rate a, comes to rest.The total time of motion is τ=25s. The average velocity during the time is equal to lt v gt =72 km/hr.How long does the partial move uniformly ?
View SolutionA car starts moving along a line, first with acceleration a=5m/s2 starting from rest then uniformly and finally decelerating at the same rate till it comes to rest. The total time of motion is 25s. The average speed during the time is 20m/s. The particle moves uniformly for (2.5x) second. Find the value of x.
View SolutionA car starts moving along a line, first with acceleration a=5m/s2 starting from rest then uniformly and finally decelerating at the same rate till it comes to rest. The total of motion is 25s. The average speed during the time is 20m/s. The particle moves uniformly for (2.5x) second. Find the value of x.
View SolutionA car starts moving rectilinearly first with acceleration α=5ms−2 (the initial velocity is equal to zero), then uniformly, and finally, deceleration at the same rate α comea to a stop. The time of motion equals t=25s. The average velocity during this time is equal to <br>=72 km h^(-1) How long does the car move unitromly?
View SolutionA car starts moving rectilinearly first with acceleration α=5ms−2 (the initial velocity is equal to zero), then uniformly, and finally, deceleration at the same rate α comea to a stop. The time of motion equals t=25s. The average velocity during this time is equal to <br>=72 km h^(-1) How long does the car move unitromly?
View SolutionA train starting from rest moves with a uniform acceleration of 0.2m/s2 for 5 minutes . Calculate the speed acquired and the distance travelled in this time.
View SolutionA car,starting from rest, accelerates at the rate f through a distance s, then continuous at constant speed for time t and then decelerates at the rate f2 come to rest. If the total distance traversed is 5 s,then :
View Solution
