Three harmonic waves having equal frequency v and same intensity I0, have phase angles 0, and π4 and −π4 respectively. When they are superimposed the intensity of the resultant wave is close to
5.8I0
3I0
I0
0.2I0
The correct Answer is:A
Ares=(√2+1)A
Ires(√2+1)2I0
=(3+2√2)I0=5.8I0
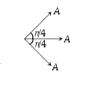
Similar Questions
Three waves of same intensity (I0) having initial phases 0,π4,−π4 rad respectively interfere at a point. Find the resultant Intensity
View SolutionThree waves of same intensity (I0) having initial phases 0,π4,−π4 rad respectively interfere at a point. Find the resultant Intensity
View SolutionTwo waves of same frequency and intensity superimpose on each other in opposite phases. After the superposition. The intensity and frequency of waves will
View SolutionEqual frequencies and intensity I_0 Angle of art of four periodic waves 0 , pi //3, 2pi //3 And pi is. When these waves are superposed, the resulting intensity nI_0 is. Then the value of n is:
View SolutionTwo waves have the same frequency . The first has intensity I0. The second has intensity 4I0 and lags behind the first in phase by π/2. When they meet , find the resultant intensity , and the phase relationship of the resultant wave with the first wave .
View SolutionIf two waves each of intensity l0 having the same frequency but differing by a constant phase angle of 60∘ superimposing at a certain point in space, then the intensity of the resultant wave is
View SolutionThree harmonic waves having equal frequency and same intensity I0 have phase angle−ϕ,0 & ϕ respectively.When they are superimposed internsity of the resultant wave becomes 4I0. Find ϕ
View SolutionFour harmonic waves of equal freuencies and equal intensity I_(0) have phase angles 0, (pi)/(3), (2pi)/(3) and pi . When they are superposed, the intensity of the resulting wave is nI_(0) . The value of n is
View Solution
