For the Equillibrium A(g)⇔B(g), ΔH = -42KJ/mol if the ratio of the activation energy of forward & backward reaction is 23 then find Efand Eb
To solve the problem, we need to find the activation energies of the forward and backward reactions given the enthalpy change (ΔH) and the ratio of the activation energies.
1. Identify the given values:
- ΔH = -42 kJ/mol (for the reaction A(g) ⇌ B(g))
- Ratio of activation energies: EfEb=23
2. Let the activation energies be defined:
- Let Eb be the activation energy of the backward reaction.
- Therefore, Ef=23Eb (from the ratio given).
3. Relate ΔH to the activation energies:
- The relationship between the activation energies and ΔH for a reversible reaction is given by:
ΔH=Ef−Eb
- Substituting the expression for Ef:
−42=23Eb−Eb
4. Simplify the equation:
- Rewrite the equation:
−42=23Eb−33Eb
- This simplifies to:
−42=−13Eb
5. Solve for Eb:
- Multiply both sides by -3 to isolate Eb:
Eb=126 kJ/mol
6. Find Ef:
- Now substitute Eb back into the equation for Ef:
Ef=23×126=84 kJ/mol
Final Answer:
- Activation energy of the forward reaction, Ef=84 kJ/mol
- Activation energy of the backward reaction, Eb=126 kJ/mol
- ΔH = -42 kJ/mol (for the reaction A(g) ⇌ B(g))
- Ratio of activation energies: EfEb=23
- Let Eb be the activation energy of the backward reaction.
- Therefore, Ef=23Eb (from the ratio given).
- The relationship between the activation energies and ΔH for a reversible reaction is given by:
ΔH=Ef−Eb
- Substituting the expression for Ef:
−42=23Eb−Eb
- Rewrite the equation:
−42=23Eb−33Eb
- This simplifies to:
−42=−13Eb
- Multiply both sides by -3 to isolate Eb:
Eb=126 kJ/mol
- Now substitute Eb back into the equation for Ef:
Ef=23×126=84 kJ/mol
- Activation energy of the forward reaction, Ef=84 kJ/mol
- Activation energy of the backward reaction, Eb=126 kJ/mol
Topper's Solved these Questions
Similar Questions
The energy change accompanying the equilibrium reaction A⇔B is −33.0kJmol−1. Calculate Energy of activation for forward and backward reaction (EfandEa) at 300 K. Given that EfandEb are in the ratio 20 : 31. Assume that pre-exponential factor is same for forward and backward reaction.
View SolutionIf Ef and Er are the activation energies of forward and backward reactions and the reaction is known to be exothermic, then
View Solution
Knowledge Check
For the equilibrium, A(g)⇔B(g),ΔH is −40kJ/mol, if the ratio of the activation energies of the forward (Ef) and reverse (Eb) reactions is 2/3, then
AEf=80kJ/mol,Eb=120kJ/molBEf=60kJ/mol,Eb=100kJ/molCEf=30kJ/mol,Eb=70kJ/molDEf=70kJ/mol,Eb=30kJ/molFor the equilibrium,
A(g)→B(g),ΔH is -40 KJ/mol.
If the ratio of the activation energies of the forward (Ef) and reverse (Eb) reactions is 23 then:AEf =60 KJ/mol, Eb=100 KJ/molBEf =30 KJ/mol, Eb=70 KJ/molCEf =80 KJ/mol, Eb=120 KJ/molDEf =70 KJ/mol, Eb=30 KJ/molA+B→C,ΔH=+60 kJ/mol
Eaf is 150 kJ. What is the activation energy for the backward reaction ?A210 kJB105 kJC90 kJD145 kJ
For a hypothetical reaction,
2A+B⇔C+ energy , the activeation energy of forward reaction (Eaf) and backward reaction (Eab) are related as
View SolutionFor a hypothetical reaction A→B then activation energy for forward and backward reactions are 19 kJ/mol and 9 kJ /mol respectively. The heat of reaction is
View SolutionFor A+B→C+D,ΔH=−20kJmol−1, the activation energy of the forward reaction is 85kJmol−1. The activation energy for backward reaction is …… kJmol−1.
View SolutionFor A+B→C+D,ΔH=−20kJmole−1. The activation energy for the forward reaction is 85 kJ. Then the activation energy for the backward reaction is ___
View SolutionThe energy change accompanying the equilibrium reaction A⇔B is −33.0kJmol−1.
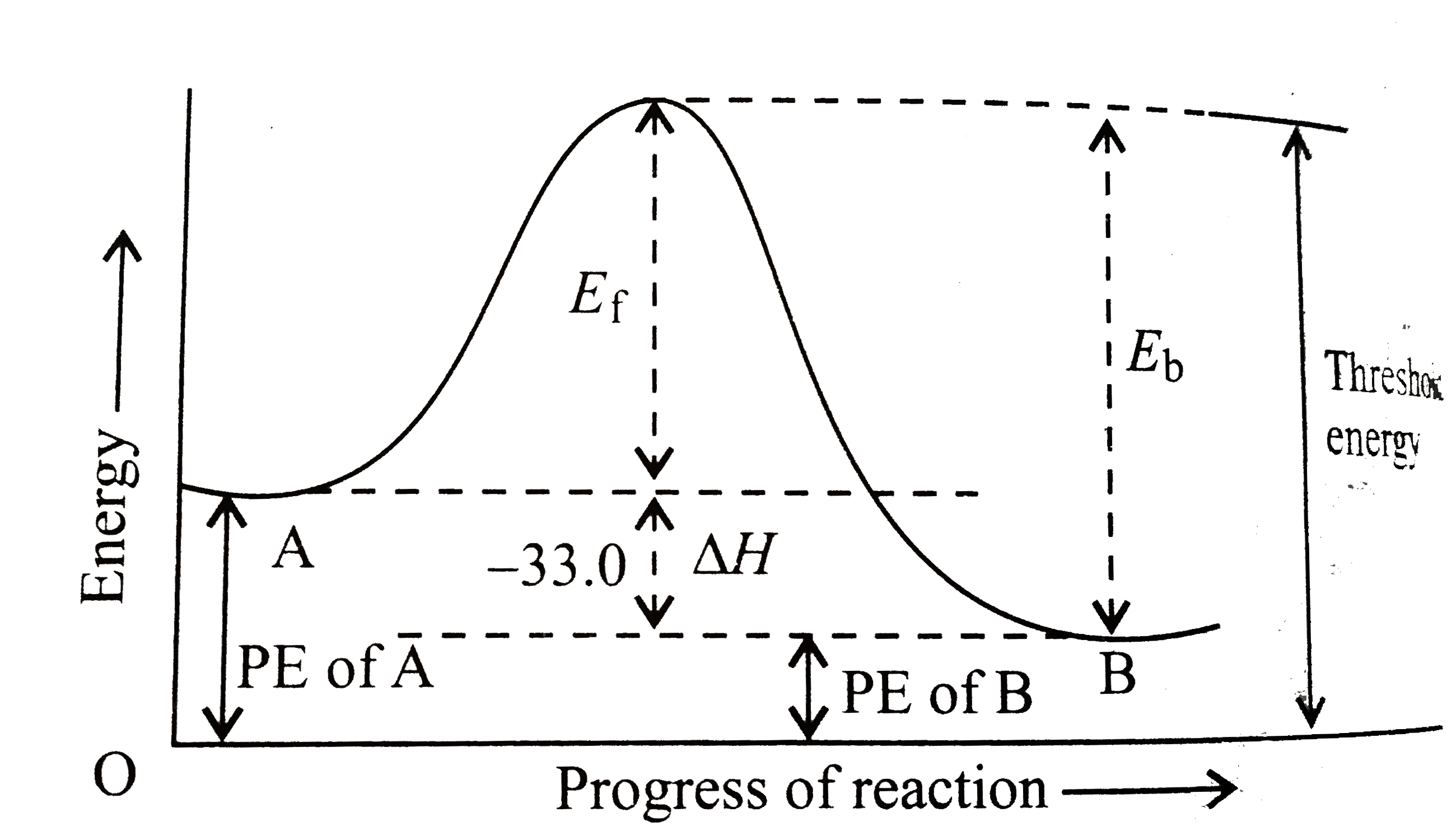
Assuming that pre-exponential factor is same for forward and backward reaction answer the following:
The energy of activation for forward and backward reactions (Ef and Eb) at 300K. Given that Ef and Eb are in the ratio 20:31,
View Solution
