A solid AxBy has ccp structure A forms ccp and B is present in all the octahedral voids if atoms A are removed from two opposite faces then x will be ?
To solve the problem, we need to analyze the structure of the solid AxBy and the implications of removing atoms from it.
1. Understanding the Structure:
- The solid AxBy has a cubic close-packed (CCP) structure where A forms the ccp lattice and B occupies all the octahedral voids.
- In a CCP structure, there are 4 atoms of A per unit cell (since it is a face-centered cubic structure).
2. Contribution of A atoms:
- In a CCP structure, there are 8 corner atoms (each contributes 18) and 6 face-centered atoms (each contributes 12).
- Therefore, the total contribution of A atoms in one unit cell is:
Total A=8×18+6×12=1+3=4
- Thus, x=4 initially.
3. Removing A from Two Opposite Faces:
- If we remove A atoms from two opposite faces, we need to consider how many A atoms are removed.
- Each face has 1 atom of A (from the face-centered positions), and since we are removing A from two opposite faces, we remove:
Removed A=2 atoms
- After removing these 2 atoms, the number of A atoms left in the structure becomes:
Remaining A=4−2=2
4. Determining the New Formula:
- After the removal, the new formula of the solid becomes A2By.
- We need to determine the value of y. Since B occupies all the octahedral voids in the original CCP structure, we know that there are 4 octahedral voids in a CCP unit cell (as there are 2 per unit cell).
- Therefore, the number of B atoms remains the same, which is y=4.
5. Final Formula:
- The new formula after removing A from the two opposite faces is A2B4.
- To find the value of x in the new formula AxBy, we have x=2.
Conclusion
Thus, the value of x after removing A from two opposite faces is:
2
- The solid AxBy has a cubic close-packed (CCP) structure where A forms the ccp lattice and B occupies all the octahedral voids.
- In a CCP structure, there are 4 atoms of A per unit cell (since it is a face-centered cubic structure).
- In a CCP structure, there are 8 corner atoms (each contributes 18) and 6 face-centered atoms (each contributes 12).
- Therefore, the total contribution of A atoms in one unit cell is:
Total A=8×18+6×12=1+3=4
- Thus, x=4 initially.
- If we remove A atoms from two opposite faces, we need to consider how many A atoms are removed.
- Each face has 1 atom of A (from the face-centered positions), and since we are removing A from two opposite faces, we remove:
Removed A=2 atoms
- After removing these 2 atoms, the number of A atoms left in the structure becomes:
Remaining A=4−2=2
- After the removal, the new formula of the solid becomes A2By.
- We need to determine the value of y. Since B occupies all the octahedral voids in the original CCP structure, we know that there are 4 octahedral voids in a CCP unit cell (as there are 2 per unit cell).
- Therefore, the number of B atoms remains the same, which is y=4.
- The new formula after removing A from the two opposite faces is A2B4.
- To find the value of x in the new formula AxBy, we have x=2.
Thus, the value of x after removing A from two opposite faces is:
2
Topper's Solved these Questions
Similar Questions
If a solid 'AB','A' atoms have ccp arrangement and B atoms occupy all the octahedral sites. If all the face centred atoms along one of the axes are removed, then what will be the resultant stoichiometry of the compound?
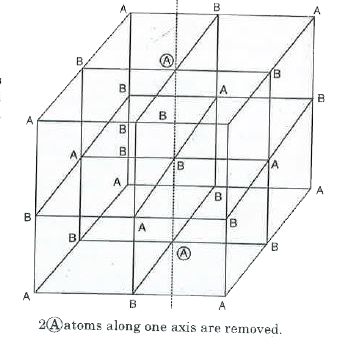 View Solution
View SolutionIn a crystalline solid, atom of A form fcc packing and atoms of B occupy all octahedral voids. If all the atoms along one body diagonal are removed then what will be the simplest formula of the crystalline solid ?
View Solution
Knowledge Check
- Question 1 - Select One
Oxygen atom forms FCC unit cell with 'A ' atoms occupying all tetrahedral voids and 'B ' atoms occupying all octahedral voids. If atoms are removed from two of the body diagonals then determine the formula of resultant compound formed .
AA4B4O7BA8B6O7CA8B8O7DA6B8O6 - Question 2 - Select One
Oxygen atom forms FCC unit cell with 'A' atoms occupying all tetrahedral voids and 'B' atoms occupying all octahedral voids. If atoms are removed from two of the body diagonals then determine the formula of resultant compound formed.
AA4B4O7BA8B6O7CA8B8O7DA6B8O6 - Question 3 - Select One
In a ccp structure of X atoms, Y atoms occupy all the octahedral holes. If 2X atom are removed from corners and replaced by Z, then the formula of the compound will be
AX15Y16ZBX7Y8ZCX7.5Y8ZDX8Y8Z3
In a solid AB, A atoms have a ccp arrangement and B atoms occupy all the octahedral sites. If all the face-centred atoms along one of the axes passing through body centres are removed, then what will be the resultant stoichiometry of compound ?
View SolutionIn a hypothetical solid C atoms form C C P lattice with A atoms occupying all the Tetrahedral Voids and B atoms occupying all the octahedral voids A and B atoms are of the appropriate size such that there is no distortions in the CCP lattice. Now if a plane is cut ( as shown ) then the section would like
 View Solution
View SolutionElement 'B' forms ccp structure and 'A' occupies half of the octahedral voids , while oxygen atoms occupy all the tetrahedral voids. The structure of bimetallic oxide is :
View SolutionElement 'B' forms ccp structure and 'A' occupies half of the octahedral voids, while oxygen atoms occupy all the tetrahedral voids. The structure of bimetallic oxide is:
View SolutionIn a hypothetical solid, C atoms are found to form cubical close-packed lattice. A atoms occupy all tetrahedral voids and B atoms occupy all octahedrals voids.
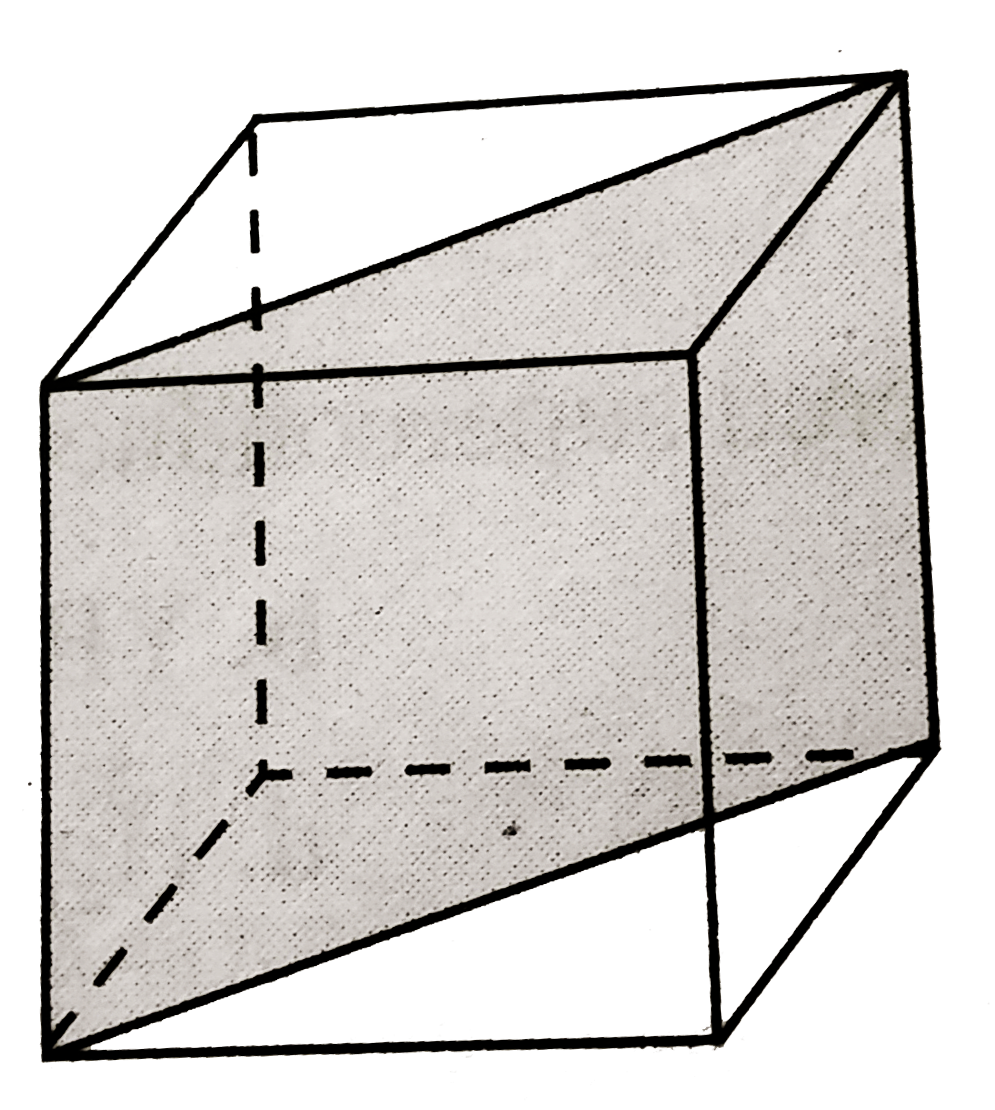
A and B atoms are of appropriate size, so that there is no distortion in the p lattice of C atoms. Now if a plane as shown in the following figure is cut, then the cross section of this plane will look likeView Solution

