What is the ratio of rate constants at 305 K and 300 K , if the activation energy of a reaction is 58.3 kJ/mol ?
To find the ratio of the rate constants at two different temperatures (305 K and 300 K) given the activation energy (Ea) of a reaction, we can use the Arrhenius equation. The formula for the ratio of rate constants (k2/k1) at two different temperatures is derived from the Arrhenius equation and is given by:
k2k1=eEaR(1T1−1T2)
However, we can also express this in logarithmic form:
logk2k1=Ea2.303R(1T1−1T2)
1. Identify the given values:
- Activation energy, Ea=58.3 kJ/mol=58.3×103 J/mol
- Gas constant, R=8.31 J/(K⋅mol)
- Temperatures: T1=300 K and T2=305 K
2. Calculate T2−T1 and T1⋅T2:
- T2−T1=305 K−300 K=5 K
- T1⋅T2=300 K⋅305 K=91500 K2
3. Substitute the values into the logarithmic equation:
logk2k1=58.3×1032.303×8.31⋅591500
4. Calculate the components:
- First, calculate 2.303×8.31:
2.303×8.31≈19.18993
- Now calculate 58.3×10319.18993:
58.3×10319.18993≈3031.82
- Finally, calculate logk2k1:
logk2k1=3031.82⋅591500≈0.165
5. Convert from logarithmic form to ratio:
- To find k2k1, we take the antilogarithm:
k2k1=100.165≈1.468
6. Final Result:
- The ratio of the rate constants at 305 K and 300 K is approximately:
k2k1≈1.5
- Activation energy, Ea=58.3 kJ/mol=58.3×103 J/mol
- Gas constant, R=8.31 J/(K⋅mol)
- Temperatures: T1=300 K and T2=305 K
- T2−T1=305 K−300 K=5 K
- T1⋅T2=300 K⋅305 K=91500 K2
logk2k1=58.3×1032.303×8.31⋅591500
- First, calculate 2.303×8.31:
2.303×8.31≈19.18993
- Now calculate 58.3×10319.18993:
58.3×10319.18993≈3031.82
- Finally, calculate logk2k1:
logk2k1=3031.82⋅591500≈0.165
- To find k2k1, we take the antilogarithm:
k2k1=100.165≈1.468
- The ratio of the rate constants at 305 K and 300 K is approximately:
k2k1≈1.5
Topper's Solved these Questions
Similar Questions
Rate of reaction at "400K" with " catalyst is equal to rate of reaction at "500K" without catalyst.What will be the activation energy of reaction if the catalyst lower the activation energy by "20KJ/mol
View SolutionThe rate constant is given by the Arrhenius equation as :
k=Ae−Ea/RT
Calcualte the ratio of the catalysed and uncatalysed rate constants at 25∘ C If the energy of activation of a analysed reaction is 162 kJ mol−1 and for uncatalysed reaction, the value is 350 kJ mol−1.
View Solution
Knowledge Check
The rate constant of a reaction at temperature 200 K is 10 times less than the rate constant at 400 K. What is the activation energy (Ea) of the reaction ? (R = gas constant)
A1842.4 RB921.2 RC460.6 RD230.3 RThe rate constant of a reaction at temperature 200K is 10 times than the rate constant at 400K . What is the activation energy (Ea) of the reaction (R=gas constant) ?
A1842.4 RB921.2 RC660.6 RD230.3 RThe rate constant of a reaction at temperature 200 K is 10 times less than the rate constant at 400 K. What is the activation energy (Ea) of the reaction ? (R = gas constant)
A1842.4 RB921.2 RC460.6 RD230.3 R
The activation energy of a reaction is 58.3 kJ mol−1. The ratio of rate constants at 305 K and 300 K is about (R= 8.3 J K−1mol−1)
View SolutionIf the half-lives of the first order reaction at 350 K and 300 K are 2 and 20 seconds respectively, the activation energy of the reaction in kJ mol−1 is :
View SolutionThe rate constant of a reaction at temperature 200 K is 10 times less than the rate constant at 400 K. What is the activation energy (Ea) of the reaction ? (R = gas constant)
View SolutionThe rate of a reaction doubles when its temperature changes form 300K to 310K. Activation energy of such a reaction will be:
(R=8.314JK−1mol−1andlog2=0.301)
View SolutionA colliison between reactant molecules must occur with a certain minimum energy before it is effective in yielding Product molecules. This minimum energy is called activation energy Ea Large the value of activation energy, smaller the value of rate constant k. Larger is the value of activation energy, greater is the effect of temperature rise on rate constant k.
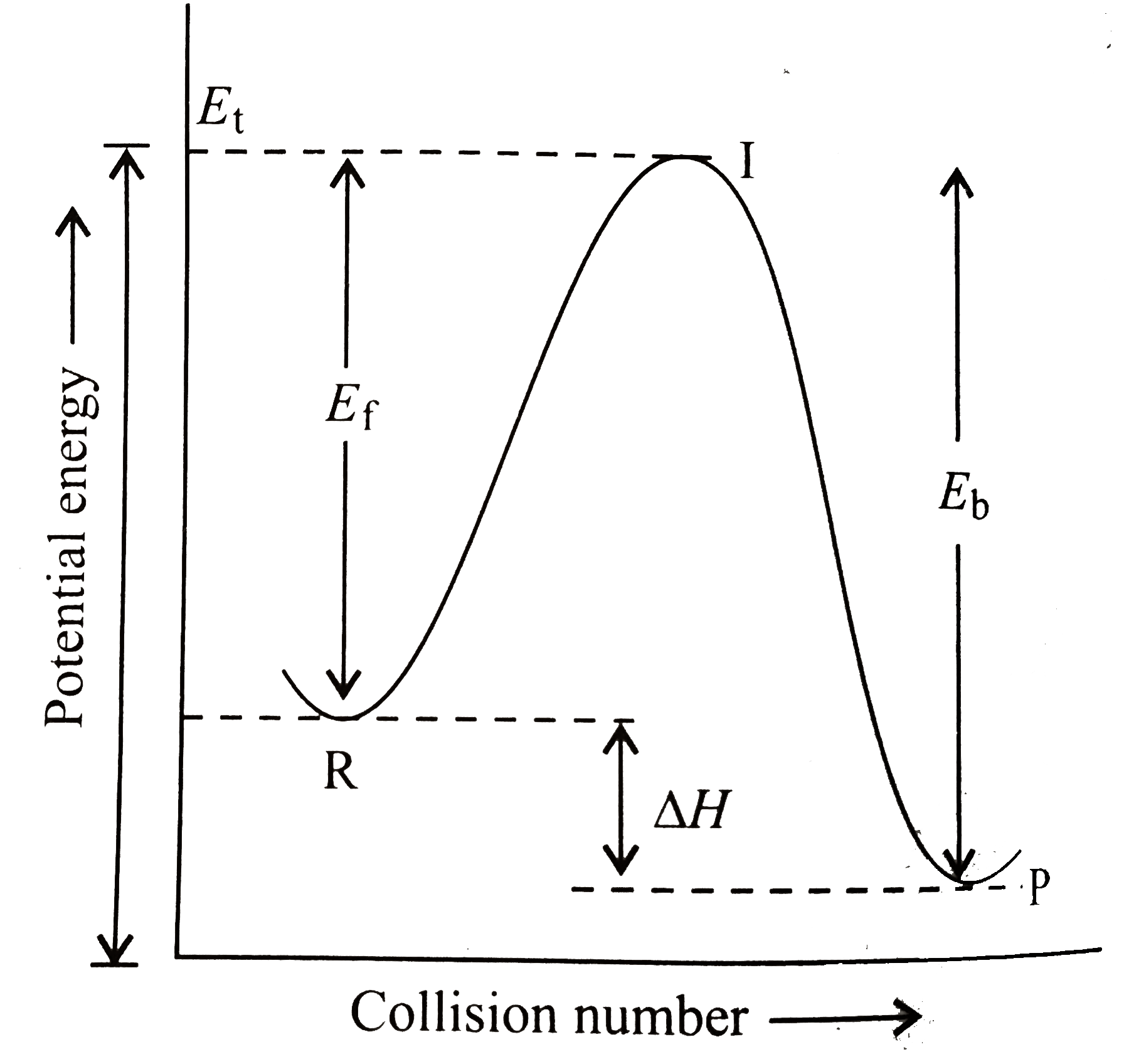
Ef= Activation energy of forward reaction
Eb= Activation energy of backward reaction
ΔH=Ef−Eb
Ef= threshold energy
The activation eneries for forward and backward reactions in a chemical reaction are 30.5 and 45.4kJmol−1 respectively. The reaction is
View Solution
