Find the molar mass of an ideal gas at 100mm Hg pressure at 235∘C temprature having a density of 0.46 g/mL (use R = 0.0821 L atm / mol /K )
To find the molar mass of the ideal gas, we can use the ideal gas equation and the relationship between density, pressure, and temperature. Let's break it down step by step.
Step 1: Write the Ideal Gas Equation
The ideal gas law is given by:
PV=nRT
where:
- P = pressure of the gas
- V = volume of the gas
- n = number of moles of the gas
- R = ideal gas constant
- T = temperature in Kelvin
Step 2: Relate Moles to Mass
The number of moles n can be expressed as:
n=mM
where:
- m = mass of the gas
- M = molar mass of the gas
Substituting this into the ideal gas equation gives:
PV=mMRT
Step 3: Rearranging for Molar Mass
Rearranging the equation to solve for molar mass M:
M=mRTPV
Step 4: Express Density in Terms of Mass and Volume
Density d is defined as:
d=mV
Thus, we can express mass as:
m=dV
Step 5: Substitute Density into the Molar Mass Equation
Substituting m=dV into the molar mass equation:
M=dV⋅RTPV
Step 6: Simplifying the Equation
The volume V cancels out:
M=dRTP
Step 7: Convert Given Values
1. Density: Given as 0.46g/mL which can be converted to 0.46g/cm3 (since 1mL=1cm3).
2. Temperature: Convert 235∘C to Kelvin:
T=235+273=508K
3. Pressure: Convert 100mmHg to atmospheres:
P=100mmHg760mmHg/atm=100760atm≈0.1316atm
Step 8: Substitute Values into the Molar Mass Equation
Now substitute d=0.46g/cm3, R=0.0821L atm / mol / K, T=508K, and P=0.1316atm:
M=0.46g/cm3×0.0821L atm / mol / K×508K0.1316atm
Step 9: Calculate Molar Mass
Calculating the numerator:
0.46×0.0821×508≈18.99
Now divide by the pressure:
M=18.990.1316≈144.1g/mol
Final Answer
The molar mass of the ideal gas is approximately 144.1g/mol.
The ideal gas law is given by:
PV=nRT
where:
- P = pressure of the gas
- V = volume of the gas
- n = number of moles of the gas
- R = ideal gas constant
- T = temperature in Kelvin
The number of moles n can be expressed as:
n=mM
where:
- m = mass of the gas
- M = molar mass of the gas
PV=mMRT
Rearranging the equation to solve for molar mass M:
M=mRTPV
Density d is defined as:
d=mV
Thus, we can express mass as:
m=dV
Substituting m=dV into the molar mass equation:
M=dV⋅RTPV
The volume V cancels out:
M=dRTP
1. Density: Given as 0.46g/mL which can be converted to 0.46g/cm3 (since 1mL=1cm3).
2. Temperature: Convert 235∘C to Kelvin:
T=235+273=508K
3. Pressure: Convert 100mmHg to atmospheres:
P=100mmHg760mmHg/atm=100760atm≈0.1316atm
Now substitute d=0.46g/cm3, R=0.0821L atm / mol / K, T=508K, and P=0.1316atm:
M=0.46g/cm3×0.0821L atm / mol / K×508K0.1316atm
Calculating the numerator:
0.46×0.0821×508≈18.99
Now divide by the pressure:
M=18.990.1316≈144.1g/mol
The molar mass of the ideal gas is approximately 144.1g/mol.
Topper's Solved these Questions
Similar Questions
Vapour density of an ideal gas which has a density 2.16 g/L at 1.12 atm pressure & 273 K temperature (R=0.0821)
View Solution(i) Prove that osmotic pressure is a colligative property.
(ii) Calculate the molar of urea solution if it exerts an osmotic pressure of 2.45 atmosphere at 300K. (R = 0.0821 L atm. mol−1K−1).
View Solution
Knowledge Check
If two moles of an ideal gas at 500 K occupies a volume of 41 litres, the pressure of the gas is (R=0.082LatmK−1mol−1)
A2 atmB3 atmC4 atmD5 atmUnder what conditions will a pure sample of an ideal gas not only exhibit a pressure of 1atm but also a concentration of 1mollitre−1
[R=0.082 iltre atm mol−1K−1]AAt STPBWhen V =22.4 LCWhen T =12 KDImpossible under any conditionsFor the given isotherm for one mole of an ideal gas, which follows Boyle's law, what will be the value of temperature (R = 0.0821 (L atm/mol/K))
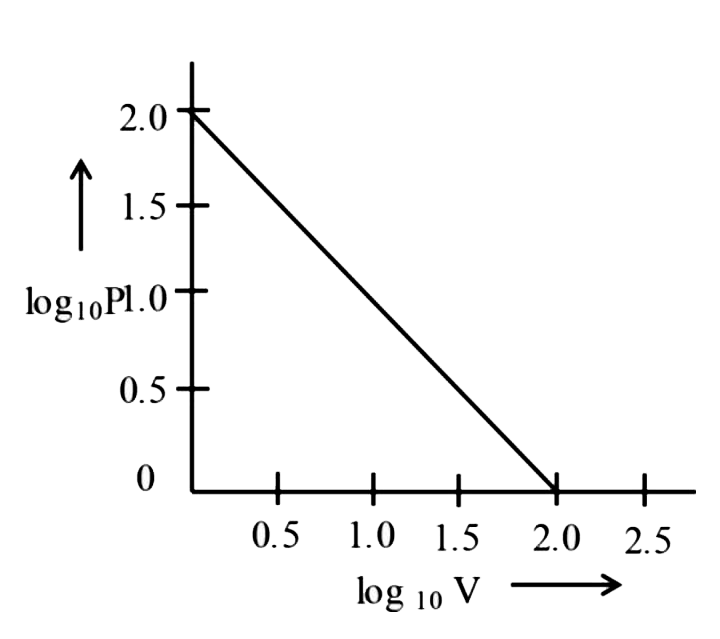 A8.2×10−4 KB1220 KC947 KD18 K
A8.2×10−4 KB1220 KC947 KD18 K
At 298 K, 100cm3 of a solution containing 3.002 g of an unidentified solute exhibits an osmotic pressure of 2.55 atmospheres. What is the molar mass of solute? (R = 0.0821 L atm. mol−1K−1)
View SolutionAn evacuated vessel weighs 50 g when empty, 144 g when filled with a liquid of density 0.47 g mL−1 and 50.5 g when filled with an ideal gas at 760 mm Hg at 300 K. The molar mass of the ideal gas is (Given R=0.082 L atm K−1mol−1)
View SolutionWhat is the molar mass of a gas that has a density of 5.66gL−1 at 35∘C and 745mm Hg?
View SolutionFor the given isotherm (P in atm and V in L) for one mole of an ideal gas, which follows Boyle's law, what will be the approx value of temperature ? (R=0.0821Latm/molK)
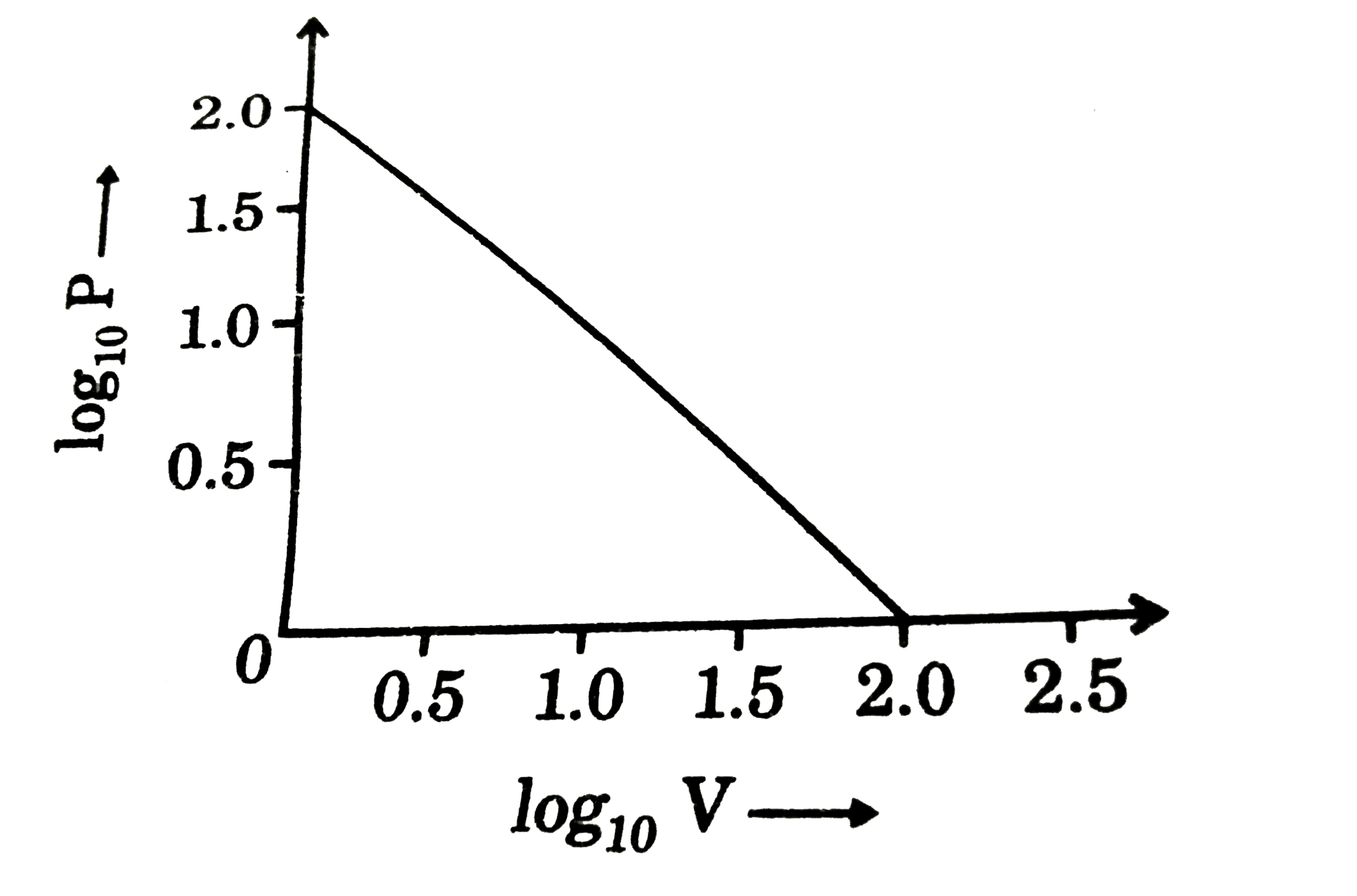
View SolutionA 2.00 litre evacuated container has a mass of 1050.0g. When the container is filled with an unknown gas at 800mm Hg pressure and 25.0∘C the mass is 1052.4g. What is the molar mass of the gas (in gmol−1)?
View Solution
