For complete combusion of methanol
CH3OH(I)+32O2→CO2(g)+2H2O(I)
the amount of heat produced as measured by bomb calorimeter is 726 kJ mol−1 at 27∘C. The enthalpy of combustion for the reaction is −x KJ mol−1 , where x is __________. (Nearest integer)
(Given : R=8.3JK−1mol−1)
To find the enthalpy of combustion for the reaction of methanol, we will use the relationship between internal energy change (ΔU) and enthalpy change (ΔH). The equation we will use is:
ΔH=ΔU+ΔNgRT
Where:
- ΔH is the enthalpy change,
- ΔU is the internal energy change,
- ΔN_g is the change in the number of moles of gas,
- R is the universal gas constant (8.314 J/(mol·K)),
- T is the temperature in Kelvin.
Step 1: Identify ΔU
The heat produced during the complete combustion of methanol is given as -726 kJ/mol. Therefore, we can write:
ΔU=−726 kJ/mol=−726,000 J/mol
Step 2: Calculate ΔN_g
Next, we need to calculate ΔN_g, which is the change in the number of moles of gas during the reaction.
From the balanced equation:
Reactants: 32 O2Products: CO2+2 H2O
The total moles of gas in the products is 1 (from CO₂) and the total moles of gas in the reactants is 32 (from O₂). Therefore:
ΔNg=Moles of gaseous products−Moles of gaseous reactants=1−32=−12
Step 3: Convert R to kJ
Now, we need to convert R from J/(mol·K) to kJ/(mol·K):
R=8.314 J/(mol⋅K)=0.008314 kJ/(mol⋅K)
Step 4: Convert Temperature to Kelvin
The temperature is given as 27°C, which we convert to Kelvin:
T=27+273=300 K
Step 5: Calculate ΔH
Now we can substitute these values into the equation for ΔH:
ΔH=ΔU+ΔNgRT
Substituting the values:
ΔH=−726,000 J/mol+(−12)×0.008314 kJ/(mol⋅K)×300 K
Calculating the second term:
ΔNgRT=−12×0.008314×300=−1.245 kJ/mol
Now substituting back:
ΔH=−726,000 J/mol−1.245 kJ/mol
Converting -726,000 J to kJ:
ΔH=−726 kJ/mol−1.245 kJ/mol=−727.245 kJ/mol
Step 6: Round to the Nearest Integer
The final value of x, which is the enthalpy of combustion, is:
x≈727 kJ/mol
Final Answer
The value of x is 727.
---
- ΔH is the enthalpy change,
- ΔU is the internal energy change,
- ΔN_g is the change in the number of moles of gas,
- R is the universal gas constant (8.314 J/(mol·K)),
- T is the temperature in Kelvin.
The heat produced during the complete combustion of methanol is given as -726 kJ/mol. Therefore, we can write:
Next, we need to calculate ΔN_g, which is the change in the number of moles of gas during the reaction.
Reactants: 32 O2Products: CO2+2 H2O
Now, we need to convert R from J/(mol·K) to kJ/(mol·K):
The temperature is given as 27°C, which we convert to Kelvin:
Now we can substitute these values into the equation for ΔH:
The final value of x, which is the enthalpy of combustion, is:
The value of x is 727.
Similar Questions
For the complete combustion of ethanol, C2H5OH(l)+3O2(g)→2CO2(g)+3H2O(l) the amount of heat produced as measured in bomb calorimeter is 1364.47KJmol−1 at 25∘C . Assuming ideality, the enthalpy of combustion, ΔHC , for the reaction will be
[R=8.314JK−1mol−1]
View SolutionFor the complete combustion of ethanol, C2H5OH(l)+3O2(g)→2CO2(g)+3H2O(l) the amount of heat produced as measured in bomb calorimeter is 1364.47KJmol−1 at 25∘C . Assuming ideality, the enthalpy of combustion, ΔHC , for the reaction will be
[R=8.314JK−1mol−1]
View Solution
Knowledge Check
For complete combustion of ethanol,
C2H5OH(l)+3O2(g)→2CO2(g)+3H2O(l), the amount of heat produced as measured in bomb calorimeter, is 1364.47 kJ mol−1 at 25∘C. Assuming ideality the enthalpy of combustion, ΔcH for the reaction will be (R = 8.314 J mol−1)A−1350.50kJmol−1B−1366.95kJmol−1C−1361.95kJmol−1D−1460.50kJmol−1For complete combustion of ethaol,
C2H5OH(l)+3O2(g)→2CO2(g)+3H2O(L)
the amount of heat produced as measured in bomb calorimeter, is 1364.47 kJ mol−1 at 25∘C. Assuming ideality the enthalpy of combustion, ΔcH, for the reaction will be (R=8.314kJmol−1)A−1366.95kJ mol−1B−1361.95kJ mol−1C−1460.95kJ mol−1D−1350.50kJ mol−1For complete combustion of ethaol,
C2H5OH(l)+3O2(g)→2CO2(g)+3H2O(L)
the amount of heat produced as measured in bomb calorimeter, is 1364.47 kJ mol−1 at 25∘C. Assuming ideality the enthalpy of combustion, ΔcH, for the reaction will be (R=8.314kJmol−1)A−1366.95kJmol−1B−1361.95kJmol−1C−1460.50kJmol−1D−1350.50kJmol−1
For the complete combustion of ethanol, C2H5OH(l)+3O2(g)→2CO2(g)+3H2O(l) the amount of heat produced as measured in bomb calorimeter is 1364.47KJmol−1 at 25∘C . Assuming ideality, the enthalpy of combustion, ΔHC , for the reaction will be
[R=8.314JK−1mol−1]
View SolutionEstimate the enthalpy of combustion of methane in KJ .mol−1
CH4(g)+2O2(g)→CO2(g)+2H2O(g)
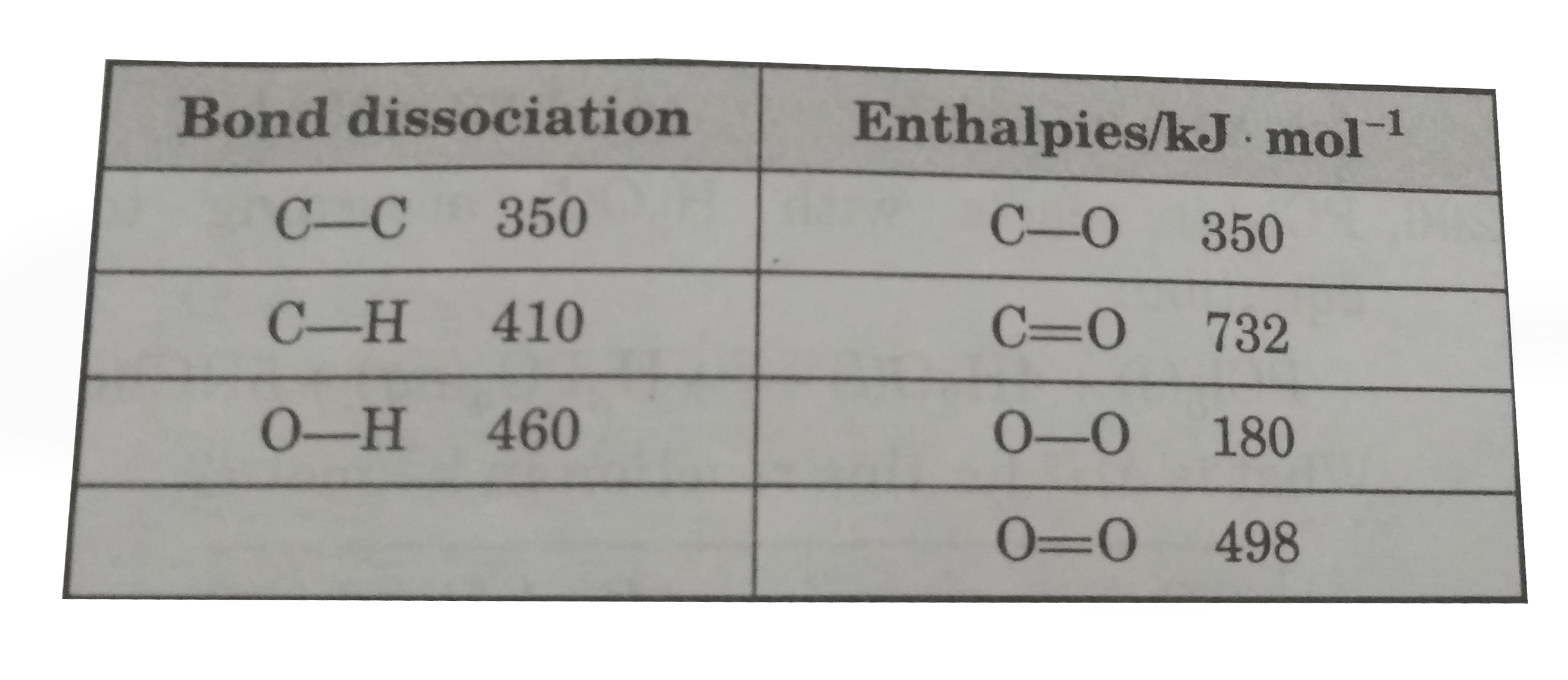
View SolutionThe combustion of benzene (l) gives CO2(g) and H2O(l). Given that heat of combustion of benzene at constant volume is –3263.9kJmol–1 at 25∘C, heat of combustion (in kJmol–1) of benzene at constant pressure will be
(R = 8.314 JK–1 mol–1)
View SolutionThe combustion of benzene (l) gives CO2(g) and H2O(l).Given that heat of combustion of benzene at constant volume is −3263.9kJmol−1 at 25∘C, heat of combustion (in kJmol−1) of benzene at constant pressure will be (R=8.314JK−1mol−1).
View SolutionThe combustion of benzene (l) gives CO2(g) and H2O(l). Given that heat of combustion of benzene at constant volume is –3263.9kJmol–1 at 25∘C, heat of combustion (in kJmol–1) of benzene at constant pressure will be
(R = 8.314 JK–1 mol–1)
View Solution
