Elevation in boiling point of non volatile solute A and B are same. Mass percent of sol A is 2% and mass percent of sol B is 8%. What is the ratio of their molar mass?
To solve the problem, we need to find the ratio of the molar masses of two non-volatile solutes A and B, given that their elevation in boiling point is the same and their mass percentages in the solution are different.
1. Understanding the Problem:
We know that the elevation in boiling point (ΔT_B) for both solutes A and B is the same. The formulas for elevation in boiling point is given by:
ΔTB=KB⋅m⋅i
where KB is the ebullioscopic constant, m is the molality, and i is the van 't Hoff factor. Since both solutes are non-volatile and non-electrolytes, i=1.
2. Setting Up the Equation:
Since ΔT_B for both solutions is the same, we can equate the two expressions:
mA=mB
3. Calculating Molality:
The molality m is defined as:
m=mass of solute (in kg)mass of solvent (in kg)
For solute A (2% solution):
- Mass of solute A = 2 g
- Mass of solvent = 100 g - 2 g = 98 g = 0.098 kg
Therefore, the molality of A:
mA=2g98g⋅10.001kg=20.098
For solute B (8% solution):
- Mass of solute B = 8 g
- Mass of solvent = 100 g - 8 g = 92 g = 0.092 kg
Therefore, the molality of B:
mB=8g92g⋅10.001kg=80.092
4. Equating the Molalities:
Since mA=mB:
2MA⋅0.098=8MB⋅0.092
Where MA and MB are the molar masses of solutes A and B respectively.
5. Cross Multiplying:
Cross-multiplying gives us:
2⋅0.092⋅MB=8⋅0.098⋅MA
6. Rearranging for the Ratio:
Rearranging the equation to find the ratio MAMB:
MAMB=2⋅0.0928⋅0.098
7. Calculating the Ratio:
Now, calculate the ratio:
MAMB=0.1840.784=0.234
Final Answer:
The ratio of the molar masses of solute A to solute B is approximately:
MAMB≈0.234
We know that the elevation in boiling point (ΔT_B) for both solutes A and B is the same. The formulas for elevation in boiling point is given by:
ΔTB=KB⋅m⋅i
where KB is the ebullioscopic constant, m is the molality, and i is the van 't Hoff factor. Since both solutes are non-volatile and non-electrolytes, i=1.
Since ΔT_B for both solutions is the same, we can equate the two expressions:
mA=mB
The molality m is defined as:
m=mass of solute (in kg)mass of solvent (in kg)
For solute A (2% solution):
- Mass of solute A = 2 g
- Mass of solvent = 100 g - 2 g = 98 g = 0.098 kg
mA=2g98g⋅10.001kg=20.098
- Mass of solute B = 8 g
- Mass of solvent = 100 g - 8 g = 92 g = 0.092 kg
mB=8g92g⋅10.001kg=80.092
Since mA=mB:
2MA⋅0.098=8MB⋅0.092
Where MA and MB are the molar masses of solutes A and B respectively.
Cross-multiplying gives us:
2⋅0.092⋅MB=8⋅0.098⋅MA
Rearranging the equation to find the ratio MAMB:
MAMB=2⋅0.0928⋅0.098
Now, calculate the ratio:
MAMB=0.1840.784=0.234
The ratio of the molar masses of solute A to solute B is approximately:
MAMB≈0.234
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
What is the mass percent of oxygen in ethanol ?
A0.5214B0.1313C0.16D0.3473 - Question 2 - Select One
Molecular mass of non-volatile solute can be determined by :
ACryoscopic methodBVictor-Meyer's methodCGraham's methodDDuma's method - Question 3 - Select One
Figure explains elevation in boiling point when a non-volatile solute is added to a solvent. Variation of vapour pressure with temperaure and elevation in boiling point is marked.

Ratio of ΔTb/Kb of 6%AB2 and 9%A2B(AB2 and A2B both are non-electrolytes) is 1 mol/kg in both cases. Hence, atomic masses of A and B are respectively.A60,90B40,40C40,10D10,40
An aqueous solution of 2% non-volatile solute exerts a pressure of 1.004 bar at the normal boiling point of the solvent. What is the molar mass of the solute ?
View SolutionIs the mass percent of a solution same as its strength ? Justify your answer.
View SolutionAn aqueous solution of 2 per cent (wt./wt) non-volatile solute exerts a pressure of 1.004 bar at the boiling point of the solvent. What is the molecular mass of the solute?
View SolutionWhat is the mass percent of carbon in carbon dioxide ?
View SolutionFigure explains elevation in boiling point when a non-volatile solute is added to a solvent. Variation of vapour pressure with temperaure and elevation in boiling point is marked.
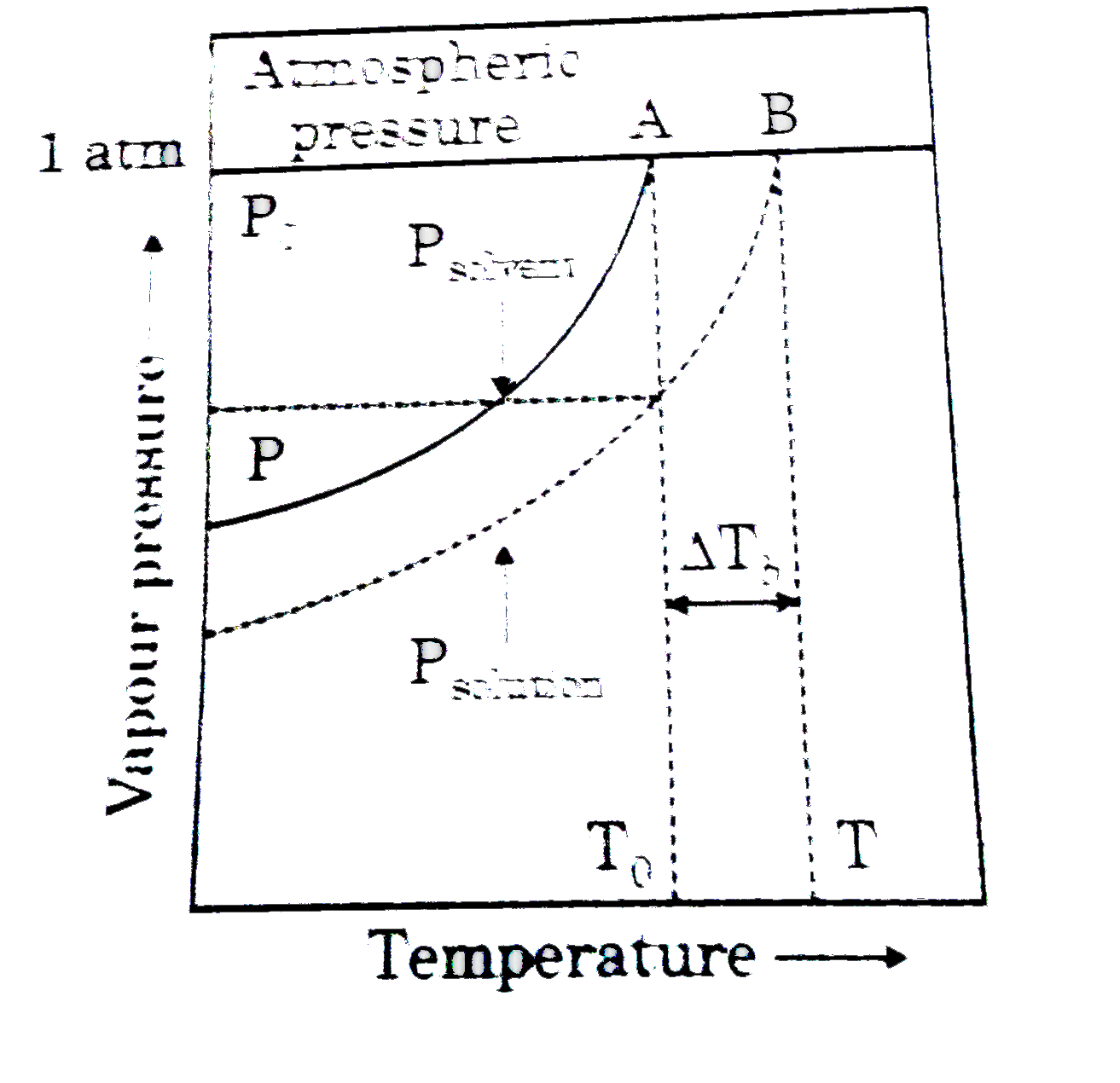
Given that ΔTb is the elevation in boiling point of the solvent in a solution of molarity m then limm→0(ΔTbm) is equal to:View Solution

