Two rods are connected in series in series between 450 C and 0. Their thermal conductivities, area of cross section and lengths are in ratio 1/2, 1/9 and 1/2. Find temperature of common junction 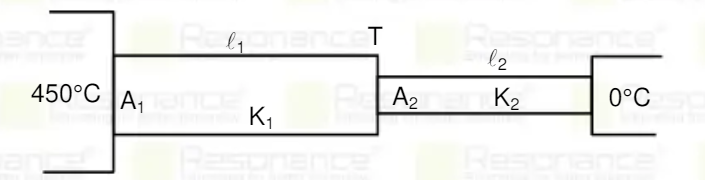
a.15 C
b.30 C
c.45 C
d.60 C
Step by step video & image solution for Two rods are connected in series in series between 450 C and 0. Their thermal conductivities, area of cross section and lengths are in ratio 1/2, 1/9 and 1/2. Find temperature of common junction by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Two metal rods 1 and 2 of same lengths have same temperature difference between their ends. Their thermal conductivities are K1 and K2 and cross sectional areas A1 and A2 respectively. If the rate of heat conduction in 1 is four times that in 2, then
View SolutionThree rods of equal length and equal area of cross section are connected end to end in series. The open end of the first rod (from left) is at a temperature of 300∘C and that of the last rod is at 20∘C in steady state. If the thermal conductivities of rods from left to right are in the ratio of 1: 3: 4, then find the temperatures of both the junctions.
View Solution
Knowledge Check
The end of two rods of different materials with their thermal conductivities, area of cross-section and lengths all in the ratio 1:2 are maintained at the same temperature difference. If the rate of flow of heat in the first rod is 4cal/s. Then, in the second rod rate of heat flow in cal/s will be
A1B2C8D16Three bars each of area of cross section A and length L are connected in series as shown in the figure. Thermal conductivities of their materials are K, 2K and 1.5K. If the temperatures of free end of first and the last bar are 200∘C and 18∘C. The value of θ1 and θ2 are (in steady state):
A120∘C,80∘CB116∘C,80∘CC116∘C,74∘CD120∘C,74∘CTwo rods of same length and cross section are joined along the length. Thermal conductivities of first and second rod are K1 and K2. The temperature of the free ends of the first and seconds rods are maintained at θ1 and θ2 respectively. The temperature of the common junction is
Aθ1+θ22BK2K2K1+K2(θ1+θ2)CK1θ1+K2θ2K1+K2DK2θ1+K1θ2K1+K2
Three bars of equal lengths x and equal area of cross-section A are connected in series. Their thermal conductivities are in the ratio 2:4:3. If the open ends of the first and the last bars are at temperatures 200∘C and 18∘C, respectively in the steady state, calculate the temperatures of both the junctions.
View SolutionThree bars of equal lenghts and equal area of cross-section are connected in series. Their thermal conductivities are in the ratio of 2:4:3. If the open ends of the first and the last bars are at temperature 200∘C and 180∘C respectivity in the steady state, calculate the temperature of both the junctions.
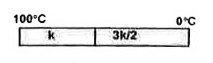
View SolutionThree rods each of same length and cross - section are joined in series. The thermal conductivity of the materials are K, 2K and 3K respectively. If one end is kept at 200∘C and the other at 100∘C. What would be the temperature of the junctions in the steady state? Assume that no heat is lost due to radiation from the sides of the rods.
View SolutionThree rods A, B and C of the same length and same cross-sectional area are joined as shown. Their thermal conductivities are in the ratio 1:2:3/2. If the open ends of A and C are at 200∘C and 18∘C, respectively, then the temperature at the junction of A at steady state is
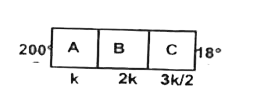
View SolutionTwo rods of similar area of cross section and length are joined as shown in the figure. The ratio of their thermal conductivity is 2:3. The free ends are maintained at 100∘C and 0∘C respectivley. THE temperature at junction in steady state is
View Solution
