Two rods of identical lengths and cross sectional area are connected in series. If σ1 and σ2 is the thermal conductivity of material of two rods then equivalent conductivity of combination is equal to
a. 2σ1σ2σ1+σ2
b. σ1σ2σ1+σ2
c. 2σ1σ2σ1−σ2
d. 2σ1σ2σ1−σ2
To solve the problem of finding the equivalent thermal conductivity of two rods connected in series, we can follow these steps:
1. Understanding the Setup:
We have two rods of identical lengths L and cross-sectional areas A. The thermal conductivities of the two rods are σ1 and σ2.
2. Resistance in Terms of Thermal Conductivity:
The thermal resistance R of a rod can be expressed as:
R=Lσ⋅A
where σ is the thermal conductivity.
3. Calculating Individual Resistances:
For the first rod with conductivity σ1:
R1=Lσ1⋅A
For the second rod with conductivity σ2:
R2=Lσ2⋅A
4. Total Resistance in Series:
Since the rods are connected in series, the total resistance Rtotal is:
Rtotal=R1+R2=Lσ1⋅A+Lσ2⋅A
5. Combining the Resistances:
We can factor out LA:
Rtotal=LA(1σ1+1σ2)
6. Finding the Equivalent Thermal Conductivity:
The equivalent thermal conductivity σeffective can be defined in terms of the total resistance:
Rtotal=Lσeffective⋅A
Setting the two expressions for Rtotal equal gives:
Lσeffective⋅A=LA(1σ1+1σ2)
7. Simplifying the Equation:
Cancel LA from both sides:
1σeffective=1σ1+1σ2
8. Finding the Equivalent Conductivity:
Taking the reciprocal gives:
σeffective=σ1⋅σ2σ1+σ2
Final Answer:
The equivalent thermal conductivity of the combination of the two rods is:
σeffective=σ1⋅σ2σ1+σ2
We have two rods of identical lengths L and cross-sectional areas A. The thermal conductivities of the two rods are σ1 and σ2.
The thermal resistance R of a rod can be expressed as:
R=Lσ⋅A
where σ is the thermal conductivity.
For the first rod with conductivity σ1:
R1=Lσ1⋅A
For the second rod with conductivity σ2:
R2=Lσ2⋅A
Since the rods are connected in series, the total resistance Rtotal is:
Rtotal=R1+R2=Lσ1⋅A+Lσ2⋅A
We can factor out LA:
Rtotal=LA(1σ1+1σ2)
The equivalent thermal conductivity σeffective can be defined in terms of the total resistance:
Rtotal=Lσeffective⋅A
Setting the two expressions for Rtotal equal gives:
Lσeffective⋅A=LA(1σ1+1σ2)
Cancel LA from both sides:
1σeffective=1σ1+1σ2
Taking the reciprocal gives:
σeffective=σ1⋅σ2σ1+σ2
The equivalent thermal conductivity of the combination of the two rods is:
σeffective=σ1⋅σ2σ1+σ2
Topper's Solved these Questions
Similar Questions
Two metal plates of same area and thickness l1 and l2 are arranged in series If the thermal conductivities of the materials of the two plates are K1 and K2 The thermal conductivity of the combination is .
View SolutionTwo rods of equal length and diameter have thermal conductivity 3 and 4 units respectively. If they are joined in series, the thermal conductivity of the combination in the given units would be
View Solution
Knowledge Check
- Question 1 - Select One
Two metal wires of identical dimesnios are connected in series. If σ1 and σ2 are the conducties of the metal wires respectively, the effective conductivity of the combination is
Aσ1σ2σ1+σ2B2σ1σ2σ1+σ2Cσ1+σ22σ1σ2Dσ1+σ2σ1σ2 - Question 2 - Select One
Two metal wires of identical dimesnios are connected in series. If σ1 and σ2 are the conducties of the metal wires respectively, the effective conductivity of the combination is
Aσ1σ2σ1+σ2B2σ1σ2σ1+σ2Cσ1+σ22σ1+σ2Dσ1+σ2σ1+σ2 - Question 3 - Select One
The metal rods of same length and same area of cross-section are connected in series. If their condcutivites are 120Wm−1and240Wm−1K−1 the effective conductivity of the combination is
A150Wm−1 K−1B160Wm−1 K−1C180Wm−1 K−1D80Wm−1 K−1
A slab consists of two parallel layers of two different materials of same thickness having thermal conductivities K1andK2. The equivalent conductivity of the combination is
View Solution Statement I: Two solid cylindrical rods of identical size and different thermal conductivity K1 and K2 are connected in series. Then the equivalent thermal conductivity of two rods system is less than that value of thermal conductivity of either rod.
Statement I: Two solid cylindrical rods of identical size and different thermal conductivity K1 and K2 are connected in series. Then the equivalent thermal conductivity of two rods system is less than that value of thermal conductivity of either rod.
Statement II: For two cylindrical rods of identical size and different thermal conductivity K1 and K2 connected in series, the equivalent thermal conductivity K is given by 2K=1K1+1K2View SolutionTwo rods of the same length and diameter having thermal conductivities K1 and K2 are joined in parallel. The equivalent thermal conductivity of combination is
View SolutionTwo rods of the same length and diameter having thermal conductivities K1 and K2 are joined in parallel. The equivalent thermal conductivity of combination is
View SolutionTwo conducting rods A and B of same length and cross-sectional area are connected (i) In series (ii) In parallel as shown. In both combination a temperature difference of 100°C is maintained. If thermal conductivity of A is 3 K and that of B is K then the ratio of heat current flowing in parallel combination to that flowing in series combination is
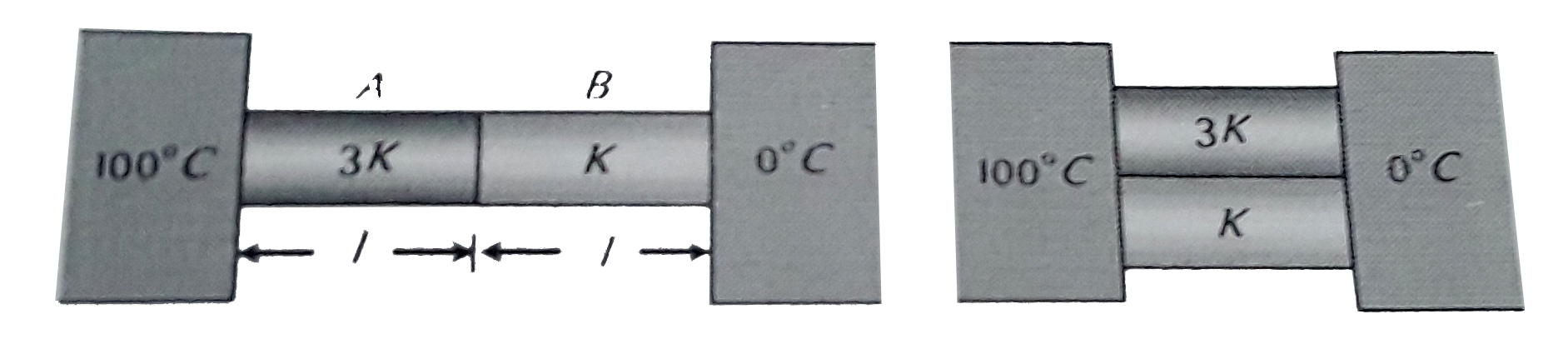 View Solution
View Solution

