Question

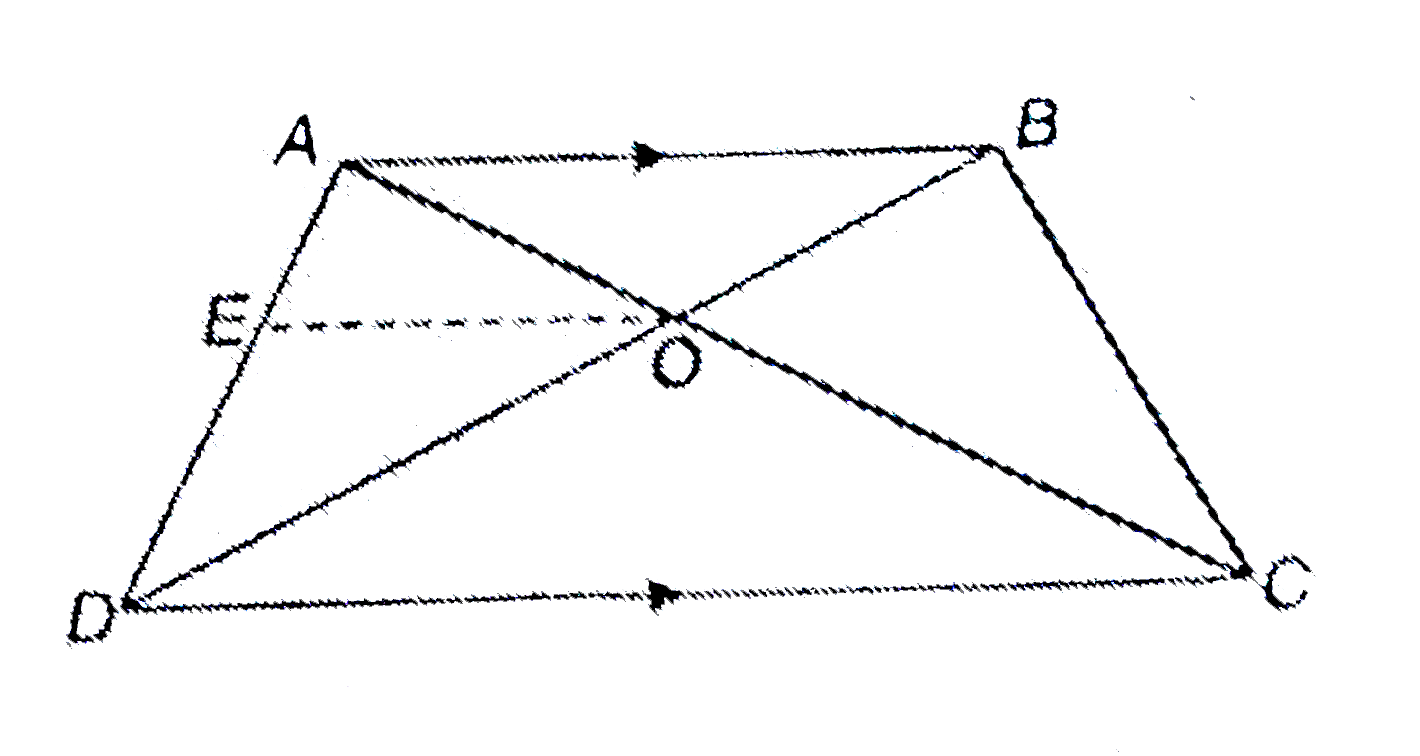
ABCD is a trapezium with AD ∥ BC and AD = 4cm. If the diagonals AC and BD intersect each other at O such that AO/OC = DO/OB =1/2, then BC =

Options

A. 6 cm
B. 7 cm
C. 8 cm
D. 9 cm

Explanation

To solve the problem, we will follow these steps:

1. Draw the trapezium ABCD:
We have a trapezium ABCD where AD is parallel to BC and AD = 4 cm.

2. Identify the point of intersection of the diagonals:
The diagonals AC and BD intersect at point O. We are given that AO/OC = DO/OB = 1/2.

3. Set up the ratios:
Since AO/OC = 1/2, we can denote AO as x and OC as 2x. Similarly, since DO/OB = 1/2, we can denote DO as y and OB as 2y.

4. Use the property of similar triangles:
Triangles AOD and BOC are similar because AD is parallel to BC. By the AA (Angle-Angle) similarity criterion, we can establish the following ratio:
\( \frac{AO}{OC} = \frac{AD}{BC} \)

5. Substituting the known values:
We know AO = x, OC = 2x, and AD = 4 cm. Therefore, we can write:
\( \frac{x}{2x} = \frac{4}{BC} \)
Simplifying the left side gives:
\( \frac{1}{2} = \frac{4}{BC} \)

6. Cross-multiply to solve for BC:
Cross-multiplying gives:
\( 1 \cdot BC = 2 \cdot 4 \)
Thus:
\( BC = 8 \text{ cm} \)

7. Conclusion:
The length of BC is 8 cm.