Let μ be the mean and σ be the standard deviation of the distribution
![Let mu be the mean and sigma be the standard deviation of the distribution where sumfi=62. If [x] denotes the greatest interger lex, then [mu^2+sigma^2] is equal to](https://d10lpgp6xz60nq.cloudfront.net/physics_images/JM_23_S2_20230410_MAT_01_Q01.png)
where ∑fi=62. If [x] denotes the greatest interger ≤x, then [μ2+σ2] is equal to
9
8
7
6
Step by step video solution for Let mu be the mean and sigma be the standard deviation of the distribution where sumf_i=62. If [x] denotes the greatest interger lex, then [mu^2+sigma^2] is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
If the standard deviation of the binomial distribution (q+p)16 is 2, then mean of the distribution is
A6B8C10D12 - Question 2 - Select One
If the standard deviation of the binomial distribution (q+p)16 is 2, then mean of the distribution is
A6B8C10D12 - Question 3 - Select One
The function f(x)=3sin2π+sin4πx+x−[x] where [x] denotes the greatest interger less than or equal to x, is
Aa periodic function with period 1Ba periodic function with period 2Ca periodic function with period 12Dnot a periodic function
If m and σ2 are the mean and variance of the random variable X whose distribution is given by
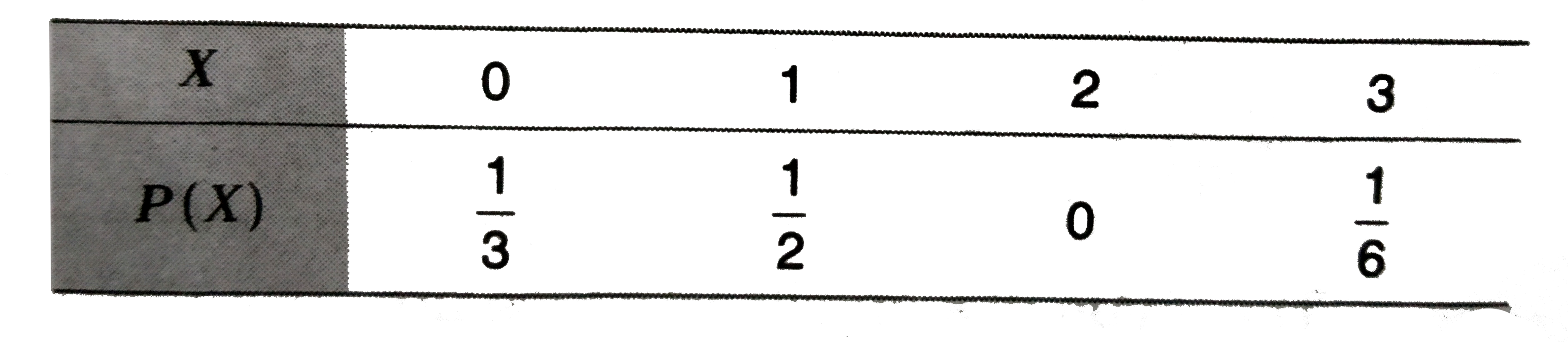
View SolutionLet f:R→A defined byf(x)=[x−2]+[4−x],(where [ ] denotes the greatest integer function).Then
View SolutionIf m and σ2 are the mean and variance of the random variable X, whose distribution is given by
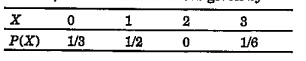
View SolutionLet f(x)=max.{x+|x|,x−[x]} , where [x] denotes the greatest integer less than or equal to x, then ∫2−2f(x) is equal to
View SolutionLet f(x) =[x]2+[x+1]−3, where [.] denotes the greatest integer function. Then
View Solution