Let C be the curve f(x)=ln2x+2lnx and A(a,f(a)),b(b,f(b)) where (a<b) are the points of tangency of two tangents drawn from origin to the curve C
Step by step video & image solution for Let C be the curve f(x)=ln^2x+2lnx and A(a,f(a)), b(b,f(b)) where (a<b) are the points of tangency of two tangents drawn from origin to the curve C by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Let f be continuous on [a, b] and assume the second derivative f" exists on (a, b). Suppose that the graph of f and the line segment joining the point (a,f(a))and(b,f(b)) intersect at a point (x0,f(x0)) where a<x0<b. Show that there exists a point c∈(a,b) such that f(c)=0.
View SolutionVerify Rolle theorem for the function f(x)=log{x2+abx(a+b)}on[a,b], where 0<a<b.
View Solution
Knowledge Check
The range of the function f(x)=x2ln(x) for x∈[1,e] is [a,b], where a+b is equal to
Ae2Be2+1Ce+1D2e2Let f (x) be a polynomial function of degree 3 where a<b<candf(a)=f(b)=f(c). If the graph of f (x) is as shown, which of the following statements are INCORRECT ? (Where c>|a|)
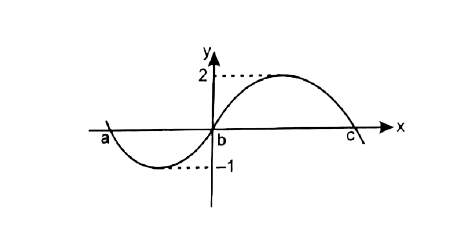A∫caf(x)dx=∫cbf(x)dx+∫bcf(x)dxB∫caf(x)dx<aC∫baf(x)dx<∫bcf(x)dxD1b−a∫baf(x)dx>1c−b∫cbf(x)dx
Verify Rolle theorem for the function f(x)=log{x2+abx(a+b)}on[a,b], where 0<a<b.
View SolutionLet g(x) be the inverse of the function f(x)=lnx2,x>0. If a,b,c∈R ,then g(a+b+c) is equal to
View SolutionThe range of the function f(x)=x2ln(x) for x∈[1,e] is [a,b], where a+b is equal to
View SolutionVerify Rolles theorem for the function f(x)=log{x2+abx(a+b)} on [a, b] , where 0<a<b .
View SolutionIf f(x)=logx(lnx) then f'(x) at x=e is
View SolutionLet f(x)=x2−2x−1∀x∈R,Letf,(−∞,a]→[b,∞), where 'a' is the largest real number for which f(x) is bijective.
The value of (a+b) is equal to
View Solution