Doubtnut Promotions Sticky

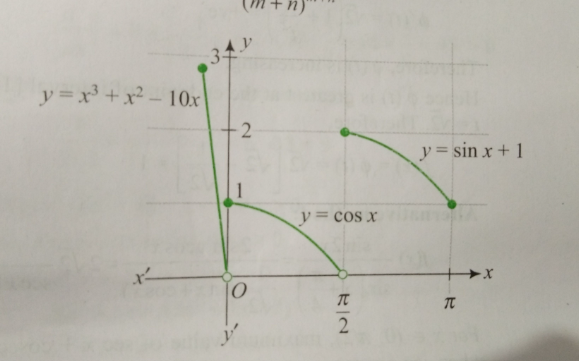
Let the function f ( x ) be defined as follows:
f ( x ) = { x 3 + x 2 - 10 x - 1 ≤ x ≤ 0 cos x 0 ≤ x ≤ π / 2 1 + sin x π / 2 ≤ x ≤ π

A

a local minimum at x= π / 2

B

a global maximum at x= π / 2

C

a absolute maximum at x=-1

D

a absolute maximum at x= π

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

Thus the graph of f ( x ) is
ocr_image

|
Updated on:21/07/2023
Class 12MATHSAPPLICATION OF DERIVATIVES

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    Let the function f(x) be defined as follows:
    f(x)=⎧⎪⎨⎪⎩x3+x2−10x−1≤x≤0cosx0≤x≤π/21+sinxπ/2≤x≤π

    Aa local minimum at x=π/2
    Ba global maximum at x=π/2
    Ca absolute maximum at x=-1
    Da absolute maximum at x=π
  • Question 2 - Select One

    Let f(x)=⎧⎪ ⎪⎨⎪ ⎪⎩x3+x2−10x−1≤x<0cosx0≤x<π21+sinxπ2≤x≤x Then at x=π2, f(x) has:

    Aa local minimum
    Ba local maximum
    Cno extremum
    Dno local maximum
  • Question 3 - Select One or More

    Let f(x) = ⎧⎪⎨⎪⎩x3+x2−10x−1≤x<0sinx0≤x<x/21+cosxπ/2≤x≤π then f(x) has

    Alocal maximum at x=π2
    Blocal minimum at x=π2
    Cabsolute minimum at x=0,π
    Dabsolute maximum at x=π2