For example 32 consider target body B initially at rest, get the expressin for velocities of bodies after the collision $(a) m_{1} = m_{2} (b) m_{2} > > m_{1} (c) m_{2} < < m_{1}$

Text Solution

Verified by Experts

Put $m_{1} = m_{2} and u_{2} = 0$ in equations (x) & (xi)
(b) Put $m_{1} = 0$ in equations (x) &n (xi)
(c ) Put $m_{2} = 0$ in equations (x) & (xi)
$u_{2} = 0$ (given)
From equation (10)
$v_{1} = \frac{(m_{1} - m_{2}) u_{1}}{m_{1} + m_{2}} ... (12)$
From equation (11)
$v_{2} = \frac{2 m_{1} u_{1}}{m_{1} + m_{2}} ... (13)$
(a) Put $m_{1} = m_{2} = m$ (say) in equations (12) & (13)
$v_{1} = \frac{(m - m) u_{1}}{m + m} = 0$
$v_{2} = \frac{2 m u_{1}}{m + m} = u_{1}$
i.e., body A comes to rest and body B starts mobing with the initial velocity of A $100 %$ KE of A is transferred to the body B.
(b) $m_{2} > > m_{1}$
$m_{1}$ can be ignored
Put $m = 0$ in equations (12) & (13)
$v_{1} = \frac{- m_{2}}{0 + m_{2}} u_{1} = - u_{1}$
$v_{2} = \frac{2 \times 0 \times u_{1}}{0 + m_{1}} = 0$
When a light body a collides against a heavy body B at rest. A rebounds with its own velocity and B continous to be at rest e.g., a ball rabounds with same speed (direction of velocity is opposite) on striking a floor.
(c ) (When target body B at rest has negligible mass) $m_{2} < < m_{1}, m_{2}$ can be ignored.
Put $m_{2} = 0$ in equations (12) and (13)
$v_{1} = - \frac{(m_{1} - 0)}{m_{1} + 0} u_{1} = u_{1}$
$v_{2} = \frac{2 m_{1} u_{1}}{m_{1} + 0} = 2 u_{1}$
When a heavy body AS undergoes an elasticv collision witha light body at rest, A keeps on moving with the same velocity of its own and B starts moving with double the initial velosity of A.

Updated on:21/07/2023

A body of mass $m_{1}$ moving at a constant speed undergoes an elastic head on collision with a body of mass $m_{2}$ initially at rest. The ratio of the kinetic energy of mass $m_{1}$ after the collision to that before the collision is -
A ${(\frac{m_{1} - m_{2}}{m_{1} + m_{2}})}^{2}$
B ${(\frac{m_{1} + m_{2}}{m_{1} - m_{2}})}^{2}$
C ${(\frac{2 m_{1}}{m_{1} + m_{2}})}^{2}$
D ${(\frac{2 m_{2}}{m_{1} + m_{2}})}^{2}$
Consider three blocks of masses $m_{1}, m_{2}, m_{3}$ interconnected by strings which are pulled by a common force $F$ on a frictionless horizontal tabel as in the figure. The tension $T_{1}$ and $T_{2}$ are also indicated
.
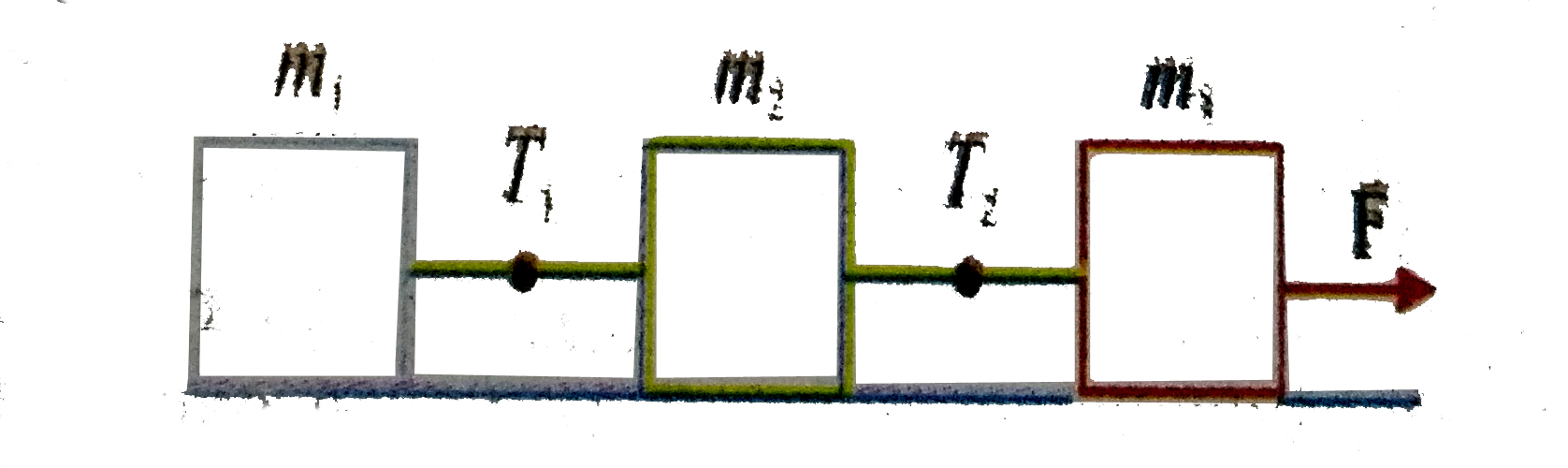a) $T_{2} > T_{1}$ if $m_{2} > m_{1}$
b) $T_{2} = T_{1}$ if $m_{2} = m_{1}$ , c) $T_{2} > T_{1}$ always
d) acceleration of the system $\frac{F}{m_{1} + m_{2} + m_{3}}$ .
A $a, b$
B $b, d$
C $a, d$
D $c, d$
A body of mass m1 collides head on perfectly elastically with a stationary body of mass $m_{2}$ . If velocities of $m_{1}$ before and after the collision are v and –v/3 respectively then the value of $m_{1} / m_{2}$ is-
A1
B2
C0.5
D4