Find the potential energy of a system of four particles each of mass 2m kept at the vertices of a square of side x. Also find the potential at the centr of the square.

Video Solution

Text Solution

Verified by Experts

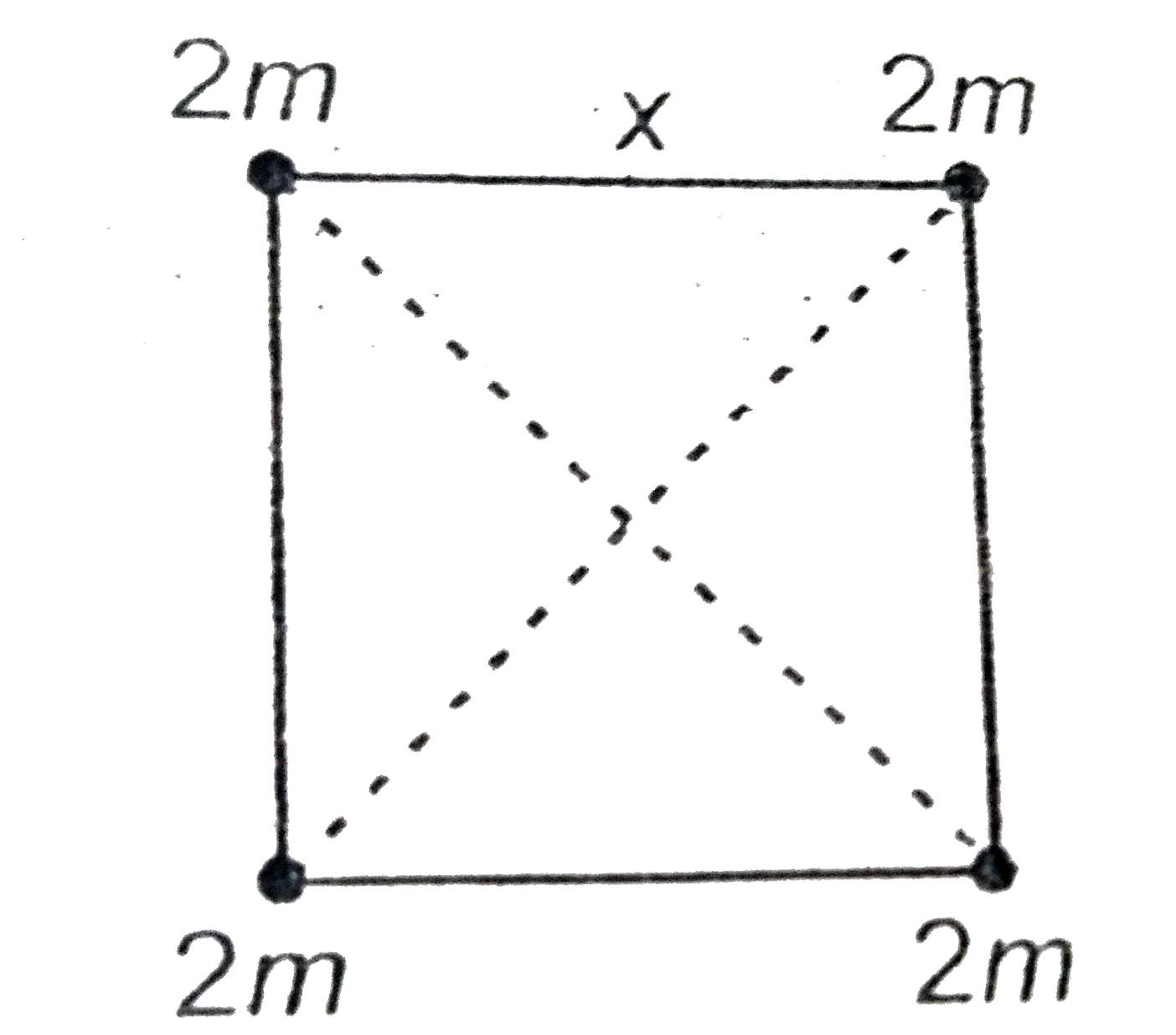
Distance of diagonal pair is $= \sqrt{x^{2} + x^{2}} = \sqrt{2} x$
Now, potential energy of system = potential enegy of pairs of mass at the ends of the sides + potential energy of masses at diagonal ends
$= 4 [\frac{- G {(2 m)}^{2}}{x}] + 2 [\frac{- G {(2 m)}^{2}}{\sqrt{2} x}]$
$= - 16 \frac{G m^{2}}{x} - \frac{8 G m^{2}}{\sqrt{2} x}$
$= - 21.64 \frac{G m^{2}}{x}$
Now, the gravitational potential at the centre will be V(centre) $= 4 ⎡ ⎢ ⎢ ⎣ \frac{- G (2 m)}{(\sqrt{2} \frac{x}{2})} ⎤ ⎥ ⎥ ⎦ = 8 \sqrt{2} \frac{G m}{x}$

|

Updated on:21/07/2023

Class 12 PHYSICS GRAVITATION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Four particles each of mass m are placed at the vertices of a square of side $l$ . the potential at the centre of square is
A $- 2 \sqrt{2} \frac{G m}{l}$
B $- 3 \sqrt{2} \frac{G m}{l}$
C $- 2 \frac{G m}{l}$
D $- 4 \sqrt{2} \frac{G m}{l}$
Question 2 - Select One
Find the potential energy of 4-particles, each of mass 1 kg placed at the four vertices of a square of side length 1 m.
A $+ 4.0 G$
B $7.5 G$
C $- 5.4 G$
D $+ 6.3 G$
Question 3 - Select One
Three particles each of mass $m$ are kept at the vertices of an euilateral triangle of side $L$ . The gravitational field at the centre due to these particle is
A $- \frac{3 G M}{L}$
B $- \frac{\sqrt{3} G M}{L}$
C $- \frac{3 \sqrt{3} G M}{L}$
D $- \frac{G M}{3 \sqrt{3} L}$

AAKASH INSTITUTE-GRAVITATION -ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)

Home
Ask Doubt
Profile