Two ropes of length l and l/2 and mass per unit length μandμ/4 respectively are joined at B and hanged vertically with a heavy mass at its end C. A pulse is generated simultaneously at both ends A and C which travels along the ropes. Find the distance from the top at which pulses will cross each other.
The correct Answer is:5l8
To solve the problem, we need to find the distance from the top at which the pulses from both ends of the ropes cross each other. We will follow these steps:
Step 1: Determine the velocities of the pulses in each rope
The velocity of a wave in a rope is given by the formula:
v=√Tμ
where T is the tension in the rope and μ is the mass per unit length.
1. For the first rope (length l, mass per unit length μ):
- The velocity v1 is given by:
v1=√Tμ
2. For the second rope (length l/2, mass per unit length μ/4):
- The velocity v2 is given by:
v2=√Tμ/4=√4Tμ=2√Tμ=2v1
Step 2: Set up the equations for the distances traveled by the pulses
Let x be the distance from the top of the first rope where the pulses cross each other. The distance traveled by the pulse from the top of the first rope to the crossing point is x, and the distance traveled by the pulse from the bottom of the second rope to the crossing point is l−x.
- Time taken by the pulse from the first rope to reach the crossing point:
t1=xv1
- Time taken by the pulse from the second rope to reach the crossing point:
t2=l−xv2
Step 3: Set the times equal to each other
Since both pulses are generated simultaneously, we can set the times equal to each other:
xv1=l−xv2
Substituting v2=2v1:
xv1=l−x2v1
Step 4: Solve for x
We can simplify the equation by multiplying both sides by 2v1:
2x=l−x
Now, add x to both sides:
3x=l
Thus, we find:
x=l3
Step 5: Conclusion
The distance from the top at which the pulses will cross each other is:
l3
- The velocity v1 is given by:
v1=√Tμ
- The velocity v2 is given by:
v2=√Tμ/4=√4Tμ=2√Tμ=2v1
t1=xv1
t2=l−xv2
Topper's Solved these Questions
- WAVESBOOK - AAKASH INSTITUTECHAPTER - WAVESEXERCISE - Assignment (Section-J) (Subjective Type Questions)
4 Videos
- WAVESBOOK - AAKASH INSTITUTECHAPTER - WAVESEXERCISE - Assignment (Section-J) (Paragraph Type Questions)
4 Videos
- WAVESBOOK - AAKASH INSTITUTECHAPTER - WAVESEXERCISE - Assignment (Section-H) (Mutiple true-false type questions)
3 Videos
- WAVE OPTICSBOOK - AAKASH INSTITUTECHAPTER - WAVE OPTICSEXERCISE - Assignment (Section-J (Aakash Challengers question))
1 Video
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - D)
15 Videos
Similar Questions
A pulse is generated at lower end to a hanging rope of uniform density and length L. The speed of the pulse when it reaches the mid point of rope is
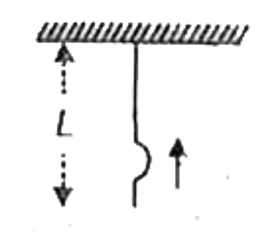
View SolutionA rope of length L and uniform linear density is hanging from the ceiling. A transverse wave pulse, generated close to the free end of the rope, travels upwards through the rope. Select the correct option:
View Solution
Knowledge Check
Two thin rods of same length l but of different uniform mass per unit length μ1 and μ2 respectively are joined together. The system is ortated on smooth horizontal plane as shown in figure. The tension at the joint will be
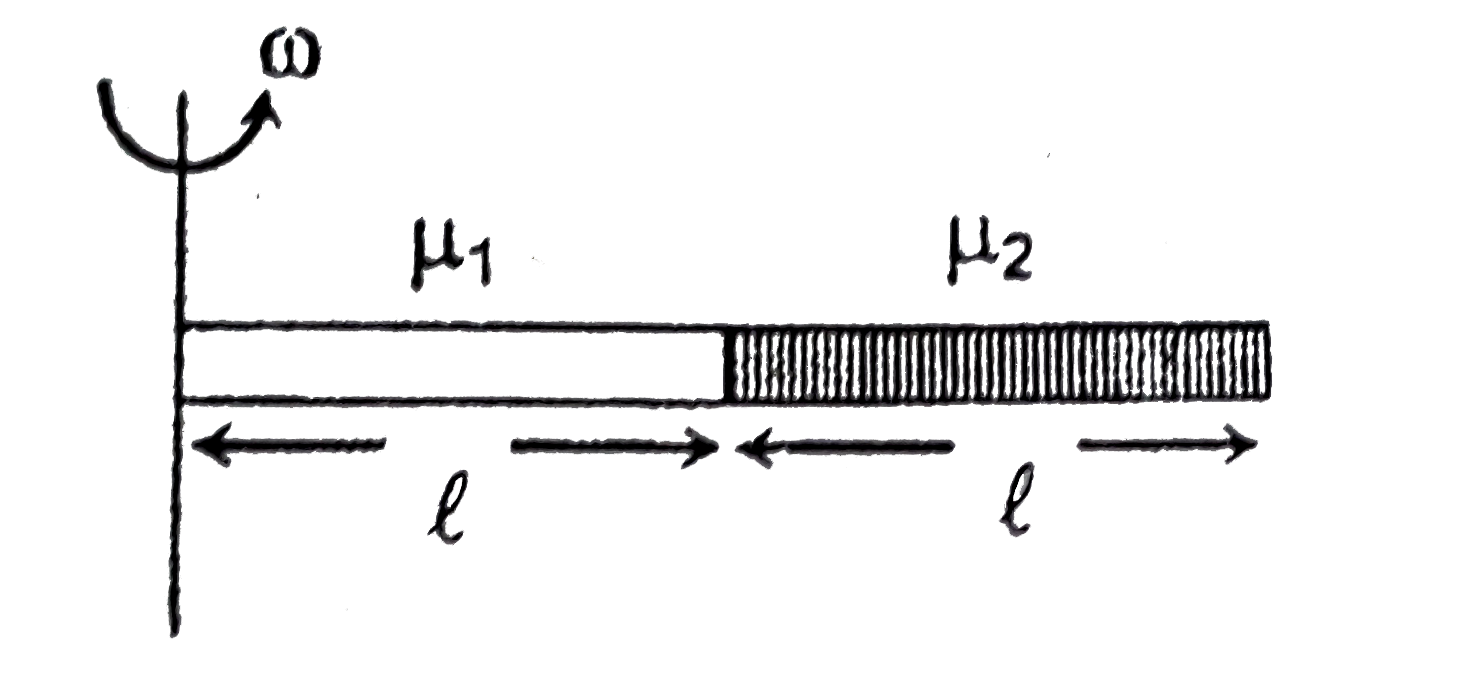 A32μ2l2ω2B32(μ1+μ2)l2ω2C32μ1l2ω2D12μ1l2ω2
A32μ2l2ω2B32(μ1+μ2)l2ω2C32μ1l2ω2D12μ1l2ω2A thick rope of length 2L and linear mass density 9μ is joined at B to a thin rope of length L and linear mass density 4μ. The systems horizontally stretched by the two vertical wall A and C. Assuming B to be a node, find the minimum number of loops in the thick rope.
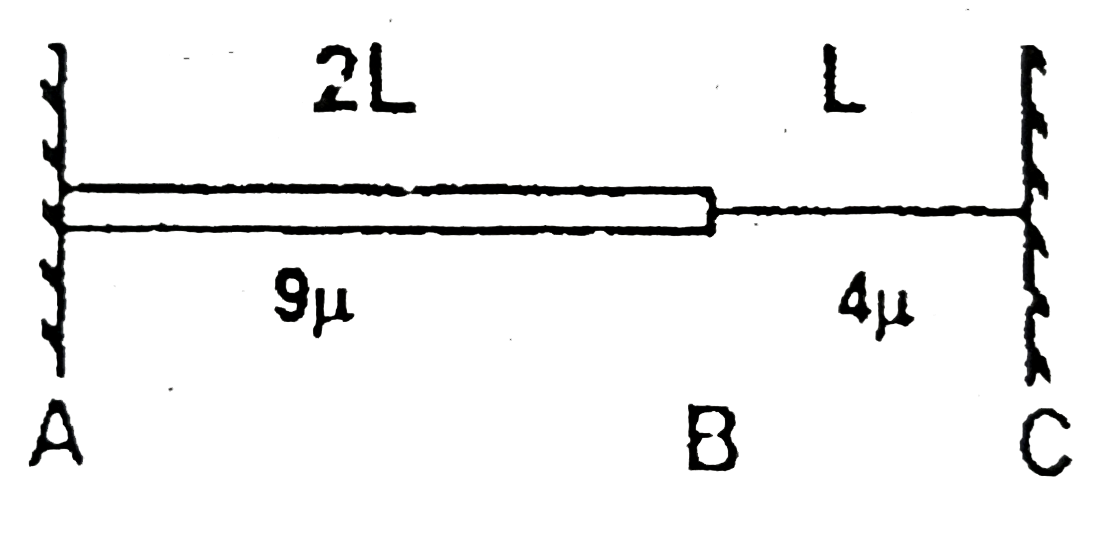 A1B2C3D4
A1B2C3D4A thin bar of length L has a mass per unit length λ, that increases linerarly with distance from one end. If its total mass is M and its mass per unit length at the lighter end is λ0, then the distance of the centre of mass from the lighter end is
AL3+λ0L28MBL3+λ0L24MCL2−λ0L24MD2L3−λ0L26M
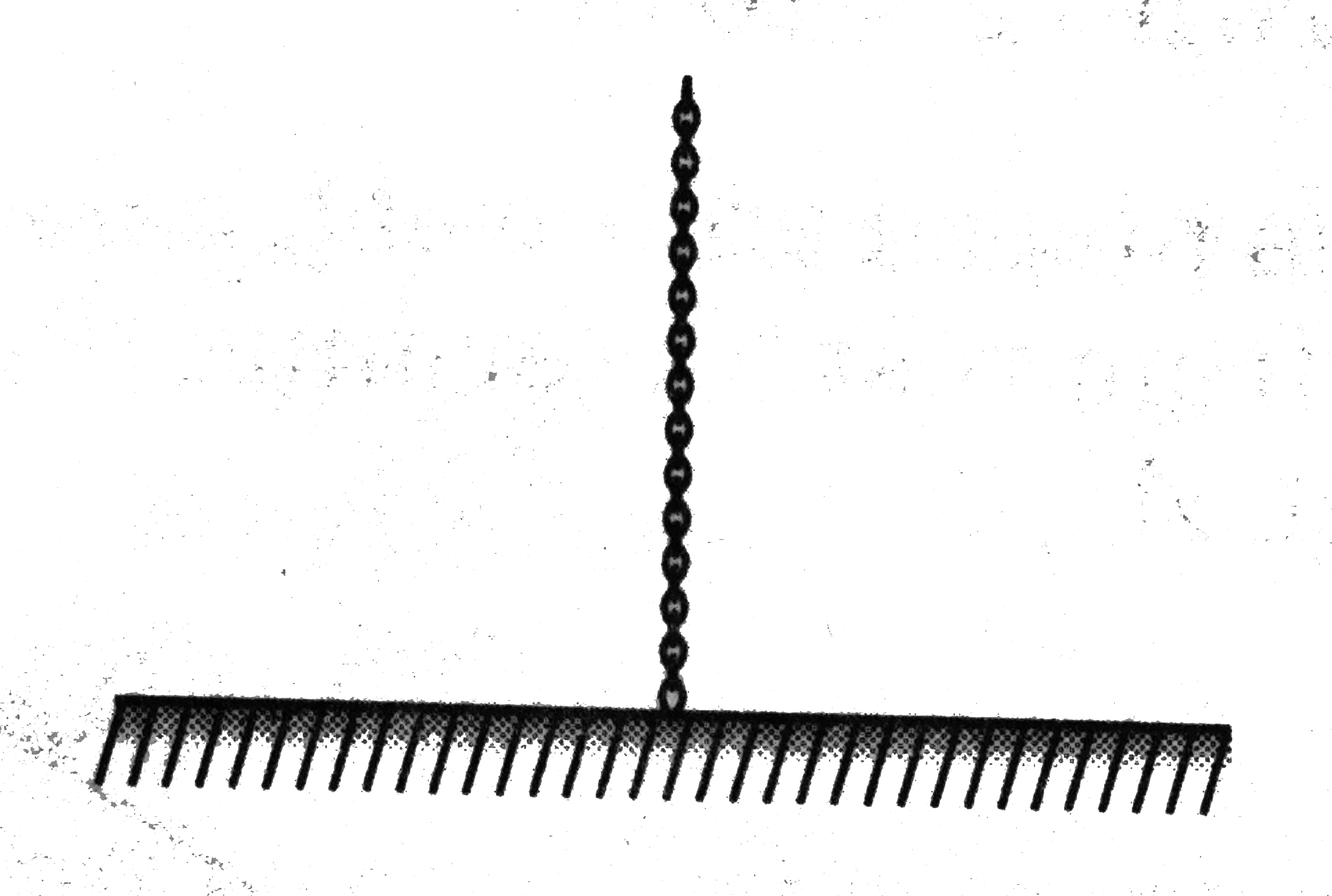
A uniform rope of mass m per unit length, hangs vertically from a support so that the lower end just touches the table top shown in figure. If it is released, show that at the time a length y of the rope has fallen, the force on the table is equivalent to the weight of the length 3y of the rope.
View SolutionA compsits wire fixed at both ends consists of two uniform parts having length l and 4l and mass per unit lengths μand4μ respectively. Then in fundamental mode of vibration, wavelength in heavy wire, keeping junction as node, is
View SolutionA string of length L and mass M hangs freely from a fixed point. Then the velocity of transverse waves along the string at a distance x from the free end is
View SolutionA uniform rope of length 20m and mass 5kg is hanging vertically from a rigid support. A block of mass 4kg is attached to the free end. The wave length of the transverse wave pulse at the lower end of the rope is 0.04 m. The wavelength of the same pulse as it reaches the top is
View SolutionA non-uniform rope of length l hangs from a ceiling.Mass per unit length of rope (μ) changes as μ=μ0ey, where y is the distance along the string from its lowest point.Then graph between square of velocity of wave and y will be best represented as :
View Solution