Let the trajectories cut the crve of given family at an angle α where tnaα=k.
The slope dydx=tanΨ (of the tangent to a member of the family and the slope dyTdx=tanΦ to the isogonal trajectory are connected by the relationship
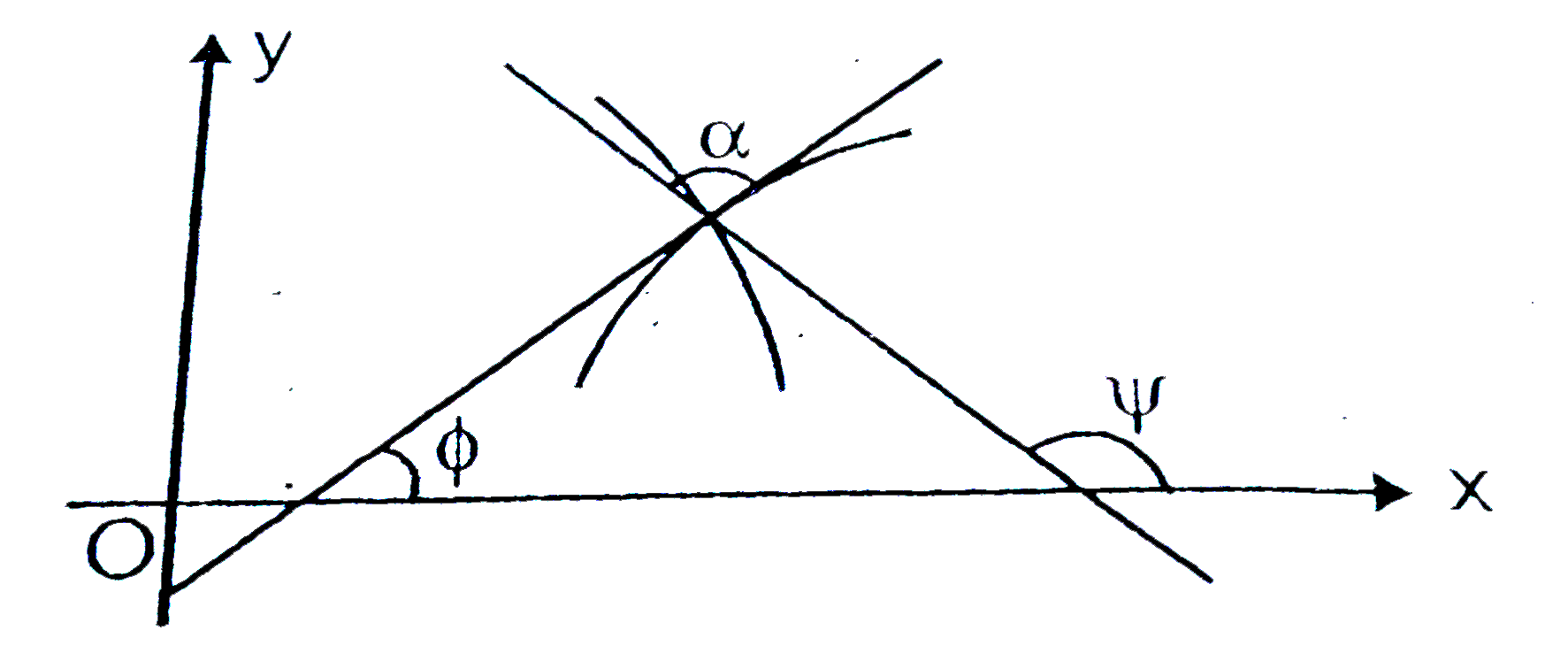
tanϕ=tan(Ψ−α)=tanΨ−tanα1+tanαtanΨ
i.e., dydx=(dyTdx)−kkdyTdx+1
Substituting this expression into equation, (l') and dropping the subscript T, we obtain the differential equation of isogonal trajectories.
The isogonal trajectories of a family of straight lines y = c, that cuts the given family at angle α, the tangent of which is k, is
y = kx
y=ktanαx
y=kcot2x
y = cx
The correct Answer is:A
Step by step video & image solution for Let the trajectories cut the crve of given family at an angle alpha where tna alpha = k. The slope (dy)/(dx) = tan Psi (of the tangent to a member of the family and the slope (dy_(T))/(dx) = tan Phi to the isogonal trajectory are connected by the relationship tan phi = tan (Psi - alpha) = (tan Psi - tan alpha)/(1+ tan alpha tan Psi) i.e., (dy)/(dx) = (((dy_(T))/(dx))-k)/(k(dy_(T))/(dx)+1) Substituting this expression into equation, (l') and dropping the subscript T, we obtain the differential equation of isogonal trajectories. The isogonal trajectories of a family of straight lines y = c, that cuts the given family at angle alpha, the tangent of which is k, is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- DIFFERENTIAL EQUATIONSBOOK - AAKASH INSTITUTECHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Assignment Section - E (Assertion - Reason Type Questions)
10 Videos
- DIFFERENTIAL EQUATIONSBOOK - AAKASH INSTITUTECHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Assignment Section-G (Integer Answer Type Questions)
3 Videos
- DIFFERENTIAL EQUATIONSBOOK - AAKASH INSTITUTECHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Assignment Section - C (Objective Type Questions) (Multiple than one options are correct)
17 Videos
- DETERMINANTS BOOK - AAKASH INSTITUTECHAPTER - DETERMINANTS EXERCISE - SECTION - J
12 Videos
- INTEGRALSBOOK - AAKASH INSTITUTECHAPTER - INTEGRALSEXERCISE - Try yourself
50 Videos
Similar Questions
Knowledge Check
tanα+2tan2α+4tan4α+8tan8α is equal to :
Atan 16 αB0CcotαDnone of these" If α=2π7 ,then the value of tanαtan2α+tan2αtan4α+tan4αtanα is "
A−5B−3C−1D−7(1+tanαtanβ)2+(tanalpha−tanbeta)2= tan2αtan2β (b) sec2αsec2β tan2αcot2β (d) sec2αcos2β
Atan2αtan2βBsec2αsec2βCtan2αcot2βDsec2αcos2β
