The co-ordinates of two fixed points A and B of a triangle ABC are (a,0) and (−a,0) respectively. If variable point C moves such that cotA+cotB=λ, (where λ is a constant) then the locus of point C is:-
(1) yλ=2a (2) y=λa
(3) ya=2λ
(4) None of these.
Step by step video & image solution for The co-ordinates of two fixed points A and B of a triangle ABC are (a,0) and (-a,0) respectively. If variable point C moves such that cot A+ cot B=lamda, (where lamda is a constant) then the locus of point C is:- (1) y lamda =2a (2) y =lamda a (3) y a=2lamda (4) None of these. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
If the lines ax+y+1=0 , x+by+1=0 and x+y+c=0 , ( a , b , c being distinct and different from 1 ) are concurrent, then 1/(1-a)+1/(1-b)+1/(1-c)= (A) 0 (B) 1 (C) 1/(a+b+c) (D) None of these
View SolutionWhich one of the following equations parametrically represents equation to a parametric profile ? (A) x=3cost ; y=4sint (B) x^2-2=-2cost ; y=4cos(t^2/2) (C) sqrt(x)=tant ; sqrt(y)=sect (D) x=sqrt(1-sint) ; y=sin(t/2)+cos(t/2)
View SolutionA variable takes the values of 0 , 1 , 2 ,....., n with frequencies proportional to the binomial coefficients C_0 , C_1 , C_2 ,......, C_n , then mean of the distribution is (1) (n(n+1))/4 (2) n/2 (3) (n(n-1))/2 (4) (n(n+1))/2
View SolutionA straight line passing through the point (2,2) and the axes enclose an area lamda . The intercepts on the axes made by the line are given by the two roots of: (A) x^2-2|lamda|x+|lamda|=0 (B) x^2+|lamda|x+2|lamda|=0 (C) x^2-|lamda|x+|2lamda|=0 (D) None of these
View SolutionThe measure of the angle between the internal and external bisectors of an angle is: (A) 60° (B) 70° (C) 80° (D) 90°
View SolutionIf the difference between mean and m0de is 63, the difference between mean and median is 189 (b) 21 (c) 31.5 (d) 48.5
View SolutionClassify they following curves as open or closed:
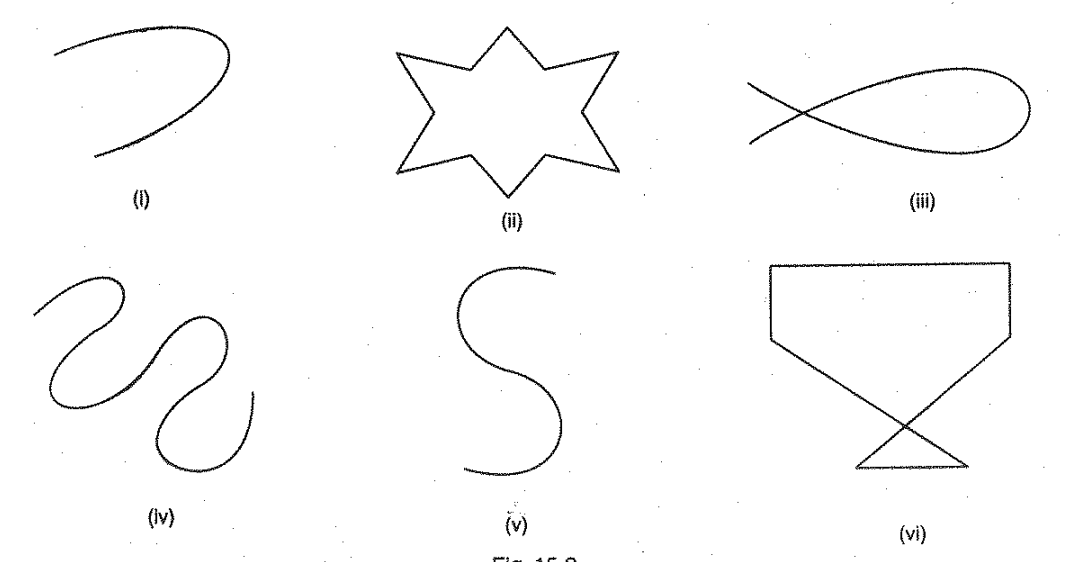
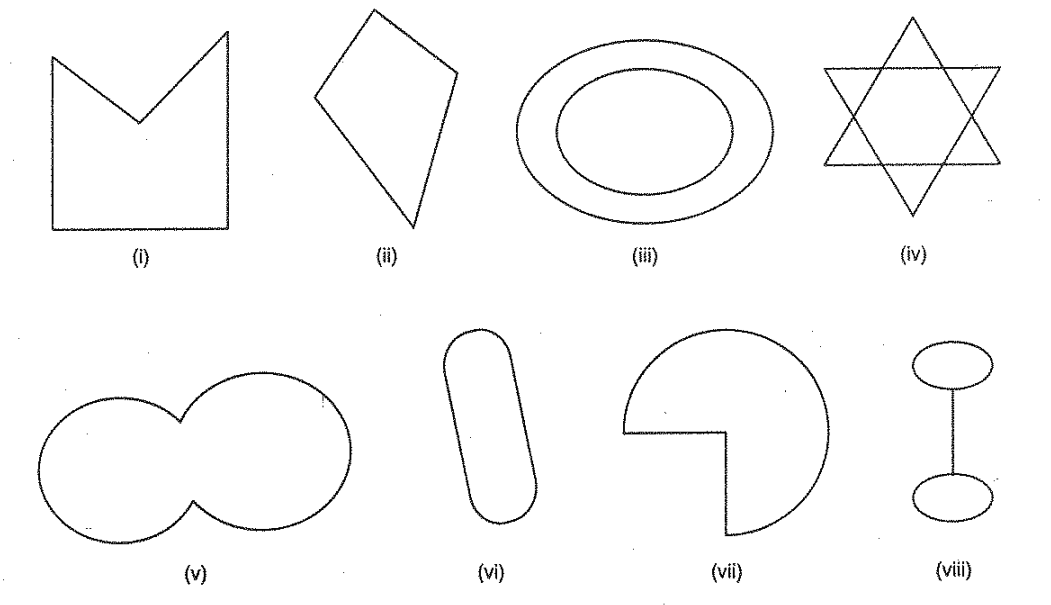
View SolutionFollowing are some figures: Classify each of these figures on the basis of the following:(i) Simple curve (ii) Simple closed curve (iii) Polygon (iv) Convex polygon (v)Concave polygon (vi) Not a curve
View Solution
