The sum of last 3 digits of 3100 is
Video Solution
Text Solution
As we know that 3100=950=(10−1)50
by using binomial expansion we can write
(10−1)50=.50C01050−.50C11049+.....50C48(10)2−.50C49(10)1+.50C50
=50×492×100−50×10+1
=122500−500+1
=122001
So, sum of last 3 digit is =0+0=1=1
option A is correct.
|
Similar Questions
Knowledge Check
The last digit of 340 is
A1B3C7D9If a certain number of two digits is divided by the sum of its digits, the quotient is 6 and the remainder is 3. If the digits are reversed and the resulting number is divided by the sum of the digits, the quotient is 4 and the remainder is 9. The sum of the digits of the number is
A6B9C12D4The last digit of 334n+1, is
A0B4C8D3
The last digit of 9!+39966 is :
View SolutionThe last digit of 9!+39966 is :
View SolutionThe sum of all the 3 digit numbers is
View SolutionA 2 digit number is 3 times the sum of its digits. If 45 is added to the number, its digits are interchanged. The sum of digits of the number is
View SolutionA 2-digit number is 3 times the sum of its digits. If 45 is added to the number, its digits are Interchanged. The sum of digits of the number is
View Solution

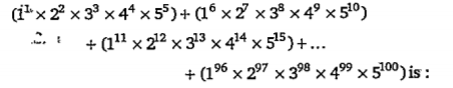 is:
is: