Calculate the concentration of H+ (aq) in 0.2 M solution of HCN. Given that the dissociation constant of HCN in water is 4.9×10−10.
The correct Answer is:9.9×10−6M
To solve the problem of calculating the concentration of H+ in a 0.2 M solution of HCN, we can follow these steps:
Step 1: Write the dissociation equation
HCN dissociates in water as follows:
HCN⇌H++CN−
Step 2: Set up the expression for the dissociation constant (K)
The dissociation constant Ka for the reaction is given by:
Ka=(H+)(CN−)(HCN)
Step 3: Define the concentrations at equilibrium
Let x be the concentration of H+ ions that dissociate from HCN. At equilibrium:
- The concentration of H+ will be x.
- The concentration of CN− will also be x.
- The concentration of undissociated HCN will be 0.2−x.
Step 4: Substitute into the expression for Ka
Given Ka=4.9×10−10:
Ka=x⋅x0.2−x=x20.2−x
Step 5: Neglect x in comparison to 0.2
Since Ka is very small, we can assume x is negligible compared to 0.2 M:
Ka≈x20.2
Step 6: Rearrange to find x2
Substituting the value of Ka:
4.9×10−10=x20.2
x2=4.9×10−10×0.2
Step 7: Calculate x2
Calculating x2:
x2=4.9×10−10×0.2=9.8×10−11
Step 8: Solve for x
Taking the square root to find x:
x=√9.8×10−11≈9.899×10−6
Step 9: Final result
Thus, the concentration of H+ in the solution is approximately:
(H+)≈9.9×10−6M
HCN dissociates in water as follows:
HCN⇌H++CN−
The dissociation constant Ka for the reaction is given by:
Ka=(H+)(CN−)(HCN)
Let x be the concentration of H+ ions that dissociate from HCN. At equilibrium:
- The concentration of H+ will be x.
- The concentration of CN− will also be x.
- The concentration of undissociated HCN will be 0.2−x.
Given Ka=4.9×10−10:
Ka=x⋅x0.2−x=x20.2−x
Since Ka is very small, we can assume x is negligible compared to 0.2 M:
Ka≈x20.2
Substituting the value of Ka:
4.9×10−10=x20.2
x2=4.9×10−10×0.2
Calculating x2:
x2=4.9×10−10×0.2=9.8×10−11
Taking the square root to find x:
x=√9.8×10−11≈9.899×10−6
Thus, the concentration of H+ in the solution is approximately:
(H+)≈9.9×10−6M
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - EQUILIBRIUMEXERCISE - Advanced Problems (For Competitions)19videos
EQUILIBRIUM
- BOOK - PRADEEPCHAPTER - EQUILIBRIUMEXERCISE - Test Your Grip ( I. Multiple choice Questions)19videos
EQUILIBRIUM
- BOOK - PRADEEPCHAPTER - EQUILIBRIUMEXERCISE - Curiosity Question4videos
EQUILIBRIUM
- BOOK - PRADEEPCHAPTER - ENVIRONMENTAL CHEMISTRYEXERCISE - COMPETITION FOCUS (JEE(Main and Advanced)/Medical Entrance (VI.ASSERTION-REASON) Type II6videos
ENVIRONMENTAL CHEMISTRY
- BOOK - PRADEEPCHAPTER - EQUILIBRIUM IN PHYSICAL AND CHEMICAL PROCESSESEXERCISE - Competition Focus (Jee(Main and advanced)/Medical Entrance) VIII. ASSERTION - REASON TYPE QUESTIONS (TYPE - II)10videos
EQUILIBRIUM IN PHYSICAL AND CHEMICAL PROCESSES
Similar Questions
Calculate the H+ ion concentration in 0.10 M acetic acid solution- Given that the dissociation constant of acetic acid in water is 1.8×10−6.
View SolutionCalculate t the H+ ion concentration in 0.10 M acetic acid solution. Given that the dissociation constant of acetic acid in water is 1.8×10−5
View Solution
Knowledge Check
- Question 1 - Select One
The first ionization constant of H2S is 9.1×10−8. Calculate the concentration of HS− ion in its 0.1 M solution. How will this concentration be affected, if the solution is 0.1 M in HCl also? If the second dissociation constant of H2S is 1.2×10−13 , then calculate the concentration of S2− under both conditions. Select these four answers from the choices given below.
A B
B C
C DNone of these
DNone of these - Question 2 - Select One
The first ionization constant of H2S is 9.1×10−8. Calculate the concentration of HS− ion in its 0.1 M solution. How will this concentration be affected, if the solution is 0.1 M in HCl also? If the second dissociation constant of H2S is 1.2×10−13 , then calculate the concentration of S2− under both conditions. Select these four answers from the choices given below.
A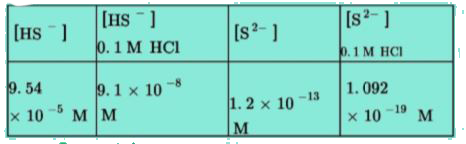 B
B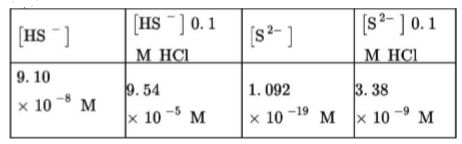 C
C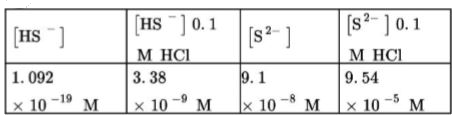 DNone of these
DNone of these
Calculate t the H+ ion concentration in 0.10 M acetic acid solution. Given that the dissociation constant of acetic acid in water is 1.8×10−5
View SolutionCalculate the concentration of H+ ion in 5×10−1M solution of H2SO4
View SolutionCalculate the pH of 0.01 M solution of NH4CN. The dissociation constants Ka for HCN=6.2×10−10andKb for NH3=1.6×10−5.
View SolutionCalculate the pH of 0.10 M solution of NH4CI . The dissociation constant (Kb)ofNH3is1.8×10−5 .
View SolutionDetermine the hydrogen ion concentration in 1.0 M solution of HCN , if its dissociation constant is 4.0×10−10.
View SolutionCalculate the approximate pH of a 0.1M H2S (aq) solution. The first and second dissociation constant of H2S are 1×10−7and1.3×10−14 respectively.
View Solution

