The mid-point of a line segment AB with A(5,6) and B(2,0) divides it in the ratio a.1:2, (b) 2:1, (c) 1:1
Step by step video & image solution for The mid-point of a line segment AB with A(5,6) and B(2,0) divides it in the ratio a.1:2, (b) 2:1, (c) 1:1 by Maths experts to help you in doubts & scoring excellent marks in Class 6 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
The mid-point of the line segment joining (2a, 4) and (-2, 2b) is (1, 2a + 1). The values of a and b are
Aa = 3, b = -1Ba = 2,b = -3Ca = 3, b = -2Da = 2, b = 3The co-ordinates of point which divides the segment joining A (0,4) and B (6,0) in the ratio 1 :2 are
A(38,12)B(12,38)C(2,83)D(83,2)The line segment joining the points A(3,2) and B (5,1) is divided at the point P in the ratio 1:2 and it lies on the line 3x−18y+k=0. Find the value of k.
A19B18C17D16
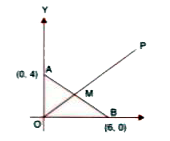
M is the mid-point of the line segment joining the points A(0, 4) and B(6, 0). M also divides the line segment OP in the ratio 1:3. Find :
co-ordinates of M
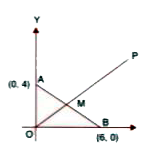
View SolutionM is the mid-point of the line segment joining the points A(0, 4) and B(6, 0). M also divides the line segment OP in the ratio 1:3. Find :
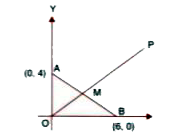
length of BP
View SolutionThe coordinates of the point P dividing the line segment joining the points A(1, 3) and B(4, 6) in the ratio 2 :1 are (2, 4) (b) (3, 5) (c) (4, 2) (d) (5, 3)
View SolutionThe line segment joining the points A(3,2) and B (5,1) is divided at the point P in the ratio 1:2 and it lies on the line 3x−18y+k=0. Find the value of k.
View SolutionA line perpendicular to the in segment joining the points (1,0) and (2,3) divides it in the ratio 1:n . Find the equation of the line.
View Solution