ABCD is a square having length of a side 20 units. Taking the centre of the square as the origin and xandy axes parallel to AB and AD respectively, find the coordinates of A, B, C and D.
To find the coordinates of the vertices A, B, C, and D of the square ABCD with a side length of 20 units and the center at the origin (0, 0), we can follow these steps:
Step 1: Understand the Position of the Square
Since the center of the square is at the origin (0, 0), and the sides of the square are parallel to the axes, we can visualize the square in the Cartesian coordinate system. The square will be symmetric about the origin.
Step 2: Determine Half the Length of the Side
The length of a side of the square is 20 units. Therefore, half of this length is:
202=10 units
Step 3: Identify the Coordinates of the Vertices
Given that the square is centered at the origin and has sides parallel to the axes, we can find the coordinates of the vertices as follows:
- Vertex A: This vertex will be located at the top left corner of the square. Since it is 10 units left and 10 units up from the center, the coordinates of A are:
A(−10,10)
- Vertex B: This vertex will be located at the top right corner of the square. It is 10 units right and 10 units up from the center, so the coordinates of B are:
B(10,10)
- Vertex C: This vertex will be located at the bottom right corner of the square. It is 10 units right and 10 units down from the center, giving the coordinates of C as:
C(10,−10)
- Vertex D: This vertex will be located at the bottom left corner of the square. It is 10 units left and 10 units down from the center, resulting in the coordinates of D as:
D(−10,−10)
Step 4: Summarize the Coordinates
Thus, the coordinates of the vertices of the square ABCD are:
- A: (-10, 10)
- B: (10, 10)
- C: (10, -10)
- D: (-10, -10)
Final Answer
The coordinates of the vertices of the square ABCD are:
- A(-10, 10)
- B(10, 10)
- C(10, -10)
- D(-10, -10)
---
Since the center of the square is at the origin (0, 0), and the sides of the square are parallel to the axes, we can visualize the square in the Cartesian coordinate system. The square will be symmetric about the origin.
The length of a side of the square is 20 units. Therefore, half of this length is:
202=10 units
Given that the square is centered at the origin and has sides parallel to the axes, we can find the coordinates of the vertices as follows:
A(−10,10)
B(10,10)
C(10,−10)
D(−10,−10)
Thus, the coordinates of the vertices of the square ABCD are:
- A: (-10, 10)
- B: (10, 10)
- C: (10, -10)
- D: (-10, -10)
The coordinates of the vertices of the square ABCD are:
- A(-10, 10)
- B(10, 10)
- C(10, -10)
- D(-10, -10)
Similar Questions
Let ABCD be a rectangle such that AB=10 units and BC=8 units. Taking AB and AD as x and y axis respectively, find the coordinates of A, B, C and D.
View SolutionEach side of a square is of length 6 units and the centre of the square Is ( - 1, 2). One of its diagonals is parallel to x+y=0. Find the co-ordinates of the vertices of the square.
View Solution
Knowledge Check
ABCD is a square of side a. The centre of the circle which circumscribes the square on taking AB and AD as axes is
A(a, -a)B(-a, a)C(1/2,a/2)DnoneABCD is a square, the length of whose side is a. Taking AB and AD as the coordinate axes, the equation of the circel passing through the ceteices of the square is
Ax2+y2+ax+ay=0Bx2+y2−ax−ay=0Cx2+y2+1ax+2ay=0Dx2+y2−2ax−2ay=0
Let ABCD be a square of side 2a. Find the coordinates of the vertices of this square when (i) A coincides with the origin and AB and AD are along OX and OY respectively. (ii) The centre of the square is at the origin and coordinate axes are parallel to the sides AB and AD respectively
View SolutionA ,B and C are the points (2,0),(5,0)and(5,3) respectively. Find coordinates of D such that ABCD is a square.
View SolutionEach side of a square is of length 4. The center of of the square is (3,7) and are of its disgonals are parallel to y=x. Then coordinates of the vertices are?
View SolutionFind the length of the side of the given square if area of the square is 625 square units and then find the value of x.
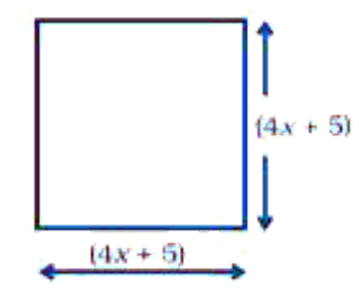
View SolutionIf the perimeter of a square is (8a+16) units. Find the length of the side of the square (in terms of a).
View SolutionABCD is a square . W is the mid point of AB side of square is 16 cm. Find the radius of circle in figure
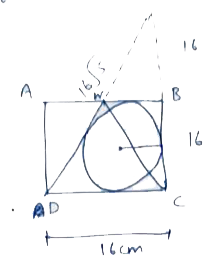
View Solution
