Three vertices of a triangle are A(1,2),B(−3,6)andC(5,4). If D, E and F are the mid-points of the sides opposite to the vertices A, B and C, respectively, show that the area of triangle ABC is four times the area of triangle DEF.
Step by step video & image solution for Three vertices of a triangle are A (1, 2), B (-3, 6) and C(5, 4). If D, E and F are the mid-points of the sides opposite to the vertices A, B and C, respectively, show that the area of triangle ABC is four times the area of triangle DEF. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Thevertices of a triangle ABC are A(−7,8),B(5,2)andC(11,0). If D, E, F are the mid-points of the sides BC,CAandAB respectively, show that ΔABC=4ΔDEF.
View SolutionIf D, E and F are the mid-points of the sides of an equilateral triangle ABC, then the ratio of the area of triangle DEF and DCF is :
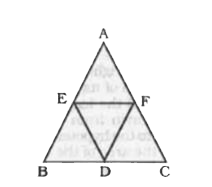
View Solution
Knowledge Check
If D, E and F are the mid-points of sides BC, CA and AB respectively of △ABC, then the ratio of the areas of △DEF to the area of △ABC is :
A1:4B1:2C1:3D2:3The vertices of a triangle are A(0,0) , B(6,0), and C(0,8) . The area of the triangle formed by the mid-point of the sides of the triangle ABC as vertices is
A24 unit2B12unit2C6 unit2D48unit2ABC is a triangle with vertices A(-1,4), B(6,-2) and C(-2,4). D, E and F are the points which divide each AB, BC and CA, respectively in the ratio 3:1 internally. Then, the centroid of the △DEF is
A(3,6)B(1,2)C(4,8)D(-3,6)
ABC is a triangle in which D, E and F are the mid-points of the sides AC, BC and AB respectively. What is the ratio of the area of the shaded to the unshaded region in the triangle?
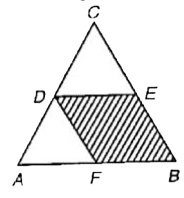
View SolutionIn the adjoining figure D, E and F are the mid-points of the sides BC, AC and AB respectively. △DEF is congruent to triangle :

View SolutionConsider a triangle ABC and let a,bandc denote the lengths of the sides opposite to vertices A,B,andC , respectively. Suppose a=6,b=10, and the area of triangle is 15√3. If ∠ACB is obtuse and if r denotes the radius of the incircle of the triangle, then the value of r2 is
View SolutionABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of ΔABCandΔDEF is :
View SolutionConsider a triangle ABC and let a , b , and c denote the lengths of the sides opposite to vertices A , B and C respectively. suppose a = 6 , b = 10 and the area of the triangle is 15√3. If ∠ACB is obtuse and if r denotes the radius of the in circle of the triangle , then r2 is equal to
View Solution
