Let ABCbe a triangle. Points D,E,F are taken on the sides AB,BC and CA respectively such that Prove that the vectors AE, B and CD form a triangle also find alpha for which the area of the triangle formed by these is least.
Step by step video & image solution for Let ABCbe a triangle. Points D,E,F are taken on the sides AB,BC and CA respectively such that (AD)/(AB)=(BE)/(BC)/=(CF)/(CA)=alpha Prove that the vectors AE, B and CD form a triangle also find alpha for which the area of the triangle formed by these is least. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
In a triangle ABC, D, E, F are the mid-points of the sides BC, CA and AB respectively , the vector →AD is equal to
A→BE+→CFB→BE−→CFC→CF−→BED−→BE−→CFLet →a,→b,→c form sides BC,CA and AB respectively of a triangle ABC then
Aa.b+b.c+c.a=0Ba×b=b×c=c×aCa.b=b.c=c.aDa×b+b×c+c×a=0In a ΔABC,D,E,F are the mid -points of the sides BC, CA and AB respectively, the vector AD is equal to
A−−→BE+−−→CFB−−→BE−−−→CFC−−→CF−−−→BED−−−→BE−−−→CF
If D, E, F are the mid-points of the sides BC, CA and AB respectively of △ABC, then ¯¯¯¯¯¯AD+23¯¯¯¯¯¯BE+13¯¯¯¯¯¯CF=
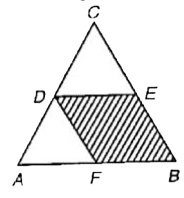
View SolutionABC is a triangle in which D, E and F are the mid-points of the sides AC, BC and AB respectively. What is the ratio of the area of the shaded to the unshaded region in the triangle?
View SolutionLet →a,→b,→c form sides BC,CA and AB respectively of a triangle ABC then
View SolutionABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of ΔABCandΔDEF is :
View SolutionABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of ΔABCandΔDEF is :
View Solution
