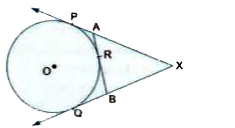
CP and CQ are tangents to a circle with centre O. ARB is another tangent touching the circle at R. If CP = 11 cm, BC = 7cm, Then the length BR is :
CP=CQ=11
AP=AR
BR=BQ
BC=CQ-QB
7=11-QB
QB=4cm
BR=4cm.
Similar Questions
The diameter of a circle with centre at C is 50 cm. CP is a radial segment of the circle. AB is a chord perpendicular to CP and passes through P. CP produced intersects the circle at D. If DP = 18 cm, then what is the length of AB?
View SolutionIn Fig , XP and XQ are two tangents to the circle with centre O , drawn from an external point X . ARB is another tangent, touching the circle at R . Prove that XA + AR = XB + BR.
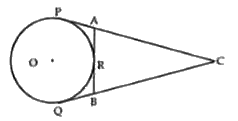
View SolutionIn the side figure, the two tangents drawn from the outer point C to the circle with center O touch the points P and Q, respectively. The tangent CP and CQ drawn on another point R of the circle intersect at points A and B respectively. If, CP=11 Cm. And BC=7 Cm. If so, determine the length of the BR.
View SolutionIn the figure, CP and CQ are tangents from an external point C to a circle with centre O. AB is another tangent which touches the circle at R. If CP=11cm and BR=4 cm. Find BC.
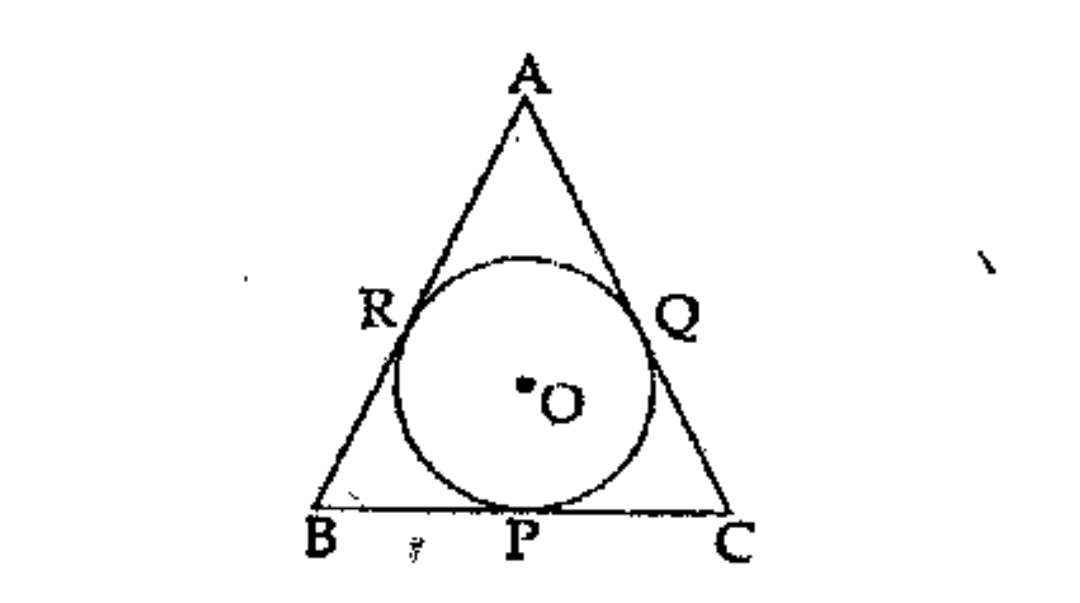
View SolutionCircle with centre O touches the sides of the triangle at P, Q and R, AB=AC,AQ=4 centimetres and CQ=6 centimetres. What is the length of CP?
 .
.
View SolutionIn figure, CP and CQ are tangents from an external point C to a circle with centre O. AB is another tangent which touches the circle at R. If CP = 11 cm and BR = 4 cm. Find the length of BC.
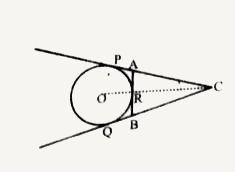
View SolutionCP and CQ are tangents to a circle with center O. ARB is another tangent touching the circle at R. If CP=11cm and BC=7cm find the BR.
View SolutionXP and XQ are tangents to a circle with centre O. ARB is another tangent touching the circle at R. Show that XA+AR=XB+BR.
View Solution
