If the solution set of |x−k|<2 is a subset of the solution set of the inequality 2x−1x+2<1, then the number of possible integral value(s) of 'k' is/are-
वीडियो समाधान
|
उत्तर
Step by step video solution for If the solution set of |x-k| < 2 is a subset of the solution set of the inequality (2x-1)/(x+2)<1, then the number of possible integral value(s) of 'k' is/are- by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023समान प्रश्न
ज्ञान जांच
If
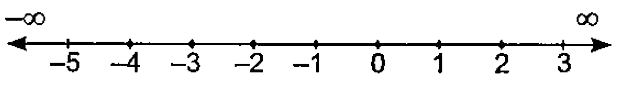 is the solution set of a linear in equation in x, then the solution set of x in set-builder form is:A{x:−4≤x≤2,x∈R}B{x:−5<x<3,x∈R}C{x:−4≤x≤2,x∈N}D{x:−5<x<3,x∈I}
is the solution set of a linear in equation in x, then the solution set of x in set-builder form is:A{x:−4≤x≤2,x∈R}B{x:−5<x<3,x∈R}C{x:−4≤x≤2,x∈N}D{x:−5<x<3,x∈I}If
 is the solution set of a linear inequation in x, then the solution set of x in set-builder form is: A{x:x<4,x∈W}B{x:x≤4,x∈W}C{x:x<4,x∈N}D{x:x≤4,x∈N}
is the solution set of a linear inequation in x, then the solution set of x in set-builder form is: A{x:x<4,x∈W}B{x:x≤4,x∈W}C{x:x<4,x∈N}D{x:x≤4,x∈N}If
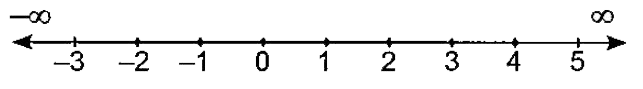 represents the solution set of a linear inequation in x, then the solution set in set-builder form is: A{x:2−<x<3,x∈I}B{x:−2<x<3,x∈W}C{x:−2<x≤3,x∈W}D{x:−2≤x≤3,x∈I}
represents the solution set of a linear inequation in x, then the solution set in set-builder form is: A{x:2−<x<3,x∈I}B{x:−2<x<3,x∈W}C{x:−2<x≤3,x∈W}D{x:−2≤x≤3,x∈I}
The solution set of |4x−3|<27 is
View SolutionFind the solution set of k so that y= is secant to the curve y=x2+k.
View SolutionFind the solution set of 2x−7<8,x∈N. Represent the solution set on the number line.
View SolutionIf
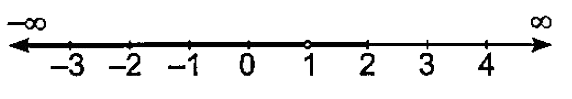 represents the solution set of a linear inequation in x, then the solution set in set-builder form is:
represents the solution set of a linear inequation in x, then the solution set in set-builder form is:
View SolutionThe solution set of |x−1|x>2 is
View Solution
