The given figure consists of and four small semi-circles of A B equal; radii and two big len semi-circles of equal radii (each 42 cm). Find the area and perimeter of Two the shaded region 25. In 19. The total surface area of a hollow cylinder which is open from both sides is 3575 cm
Area of shaded region = 2(Area of bigger semicircle− Area of smaller semi circle + Area of smaller semi circle)
=2( Area of bigger semi circle)
=2(πr22)=πr2=227∗42∗42=5544cm2
Now,
Perimeter of the shaded region =2(Perimeter of bigger semicircle +2⋅Perimeter of smaller semicircle)
=2[πr+2⋅(πr2)]
=4πr=4⋅227⋅42=528cm.
Similar Questions
The given figure consists of and four small semi-circles of A B equal; radii and two big len semi-circles of equal radii (each 42 cm). Find the area and perimeter of Two the shaded region 25. In 19. The total surface area of a hollow cylinder which is open from both sides is 3575 cm
View SolutionThe given figure consists of and four small semi-circles of equal; radii and two big semi-circles of equal radii (each 42 cm). Find the area and perimeter of the shaded region.
View Solution
Knowledge Check
- Question 1 - Select One
Three circle of equal radii touch each other as shown in figure . The radius of each circle is 1 cm. What is the area of shaded region ?
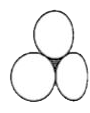 A(2√3−π2)cm2B(3√2−π3)cm2C2√3πcm2Dnone of the above
A(2√3−π2)cm2B(3√2−π3)cm2C2√3πcm2Dnone of the above - Question 2 - Select One
In the given figure there are 3 semicircles , the radii of each smaller circle is equal. If the radius of the larger circle be 22 cm , then the area of the shaded region is :
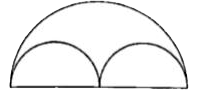 A363π4B363π3C236.5πD363π
A363π4B363π3C236.5πD363π
In the given figure ABCD is a rectangle. It consists of a circle and two semi-circles each of which are of radius 5 cm. Find the area of the shaded region. Give your answer correct to three significant figures.**
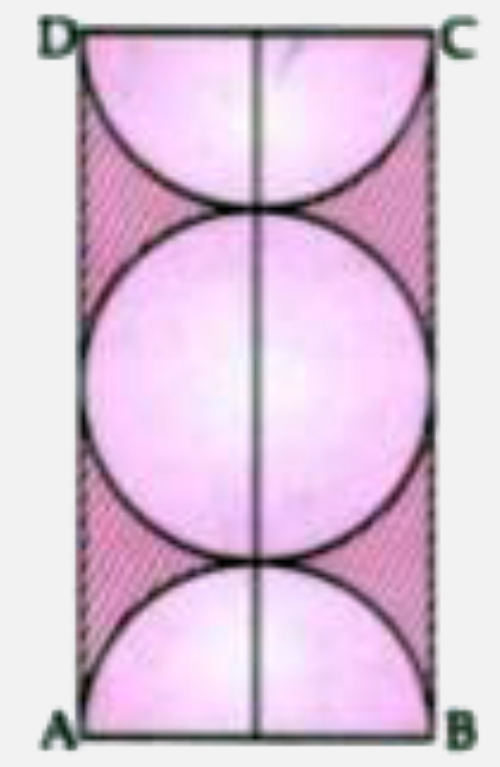 View Solution
View SolutionIn the given figure two small circles touch each other externally at the centre of a big circle and these small circles are touched internally by a big circle of radius 4cm. Find the area of the shaded region
 View Solution
View SolutionIn the give figure, the sectors of two concentric circles of radii 7 cm and 3.5 cm are shown. Find the area of the shaded region.
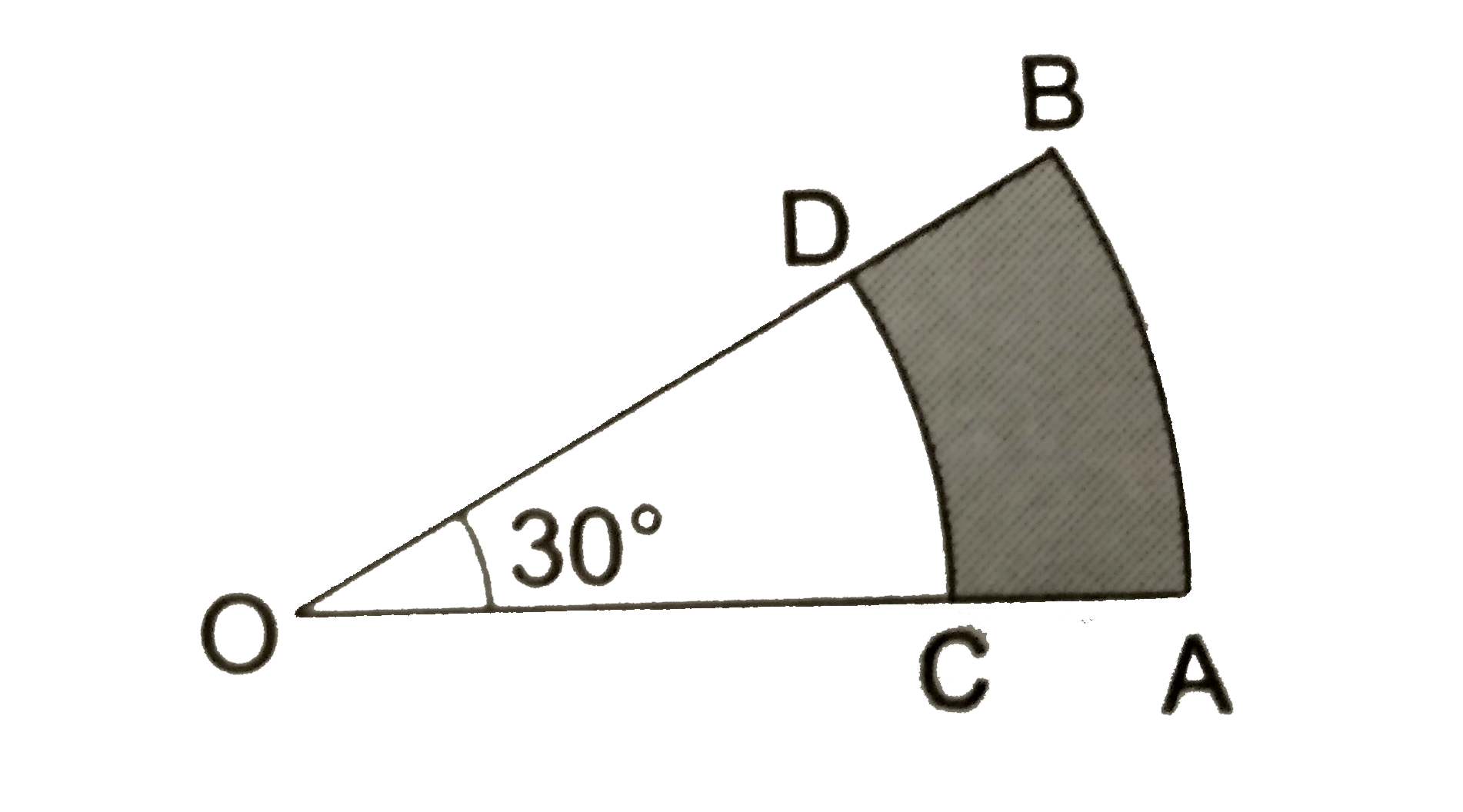 View Solution
View SolutionIn the given figure two small circles touch each other externally at the centre of a big circle and these small circles are touched internally by a big circle of radius 74cm. Find the area of the shaded region
 View Solution
View SolutionIn Figure, there are shown sectors of two concentric circles of radii 7cm and 3.5cm. Find the area of the shaded region.
(π=227)View SolutionIn the figure, ABCD is a square of side 14 cm. Semi-circles are drawn with each side of square as diameter. Find the area of the shaded region.
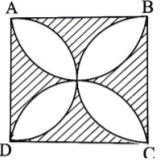 View Solution
View Solution

