The total area of the non-triangle regions in the figure below (the shaded area) is equal to (pq) square units, where p and q are coprime natural numbers then p+q is equal to
Step by step video & image solution for The total area of the non-triangle regions in the figure below (the shaded area) is equal to (p/q) square units, where p and q are coprime natural numbers then p+ q is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
If q is the square of a natural number p, then p is
AThe square root of qBEqual to qCGreater than qDThe square of qLet A=[pqqp] such that det(A)=r where p,q,r all prime number, then trace of A is equal to
A6B5C2D3~(p<→q)∧p is equal to
ApBqCp∧−pD−p∧q
Express 2.3¯¯¯¯45 in the form of p/q where p and q are coprimes.
View Solution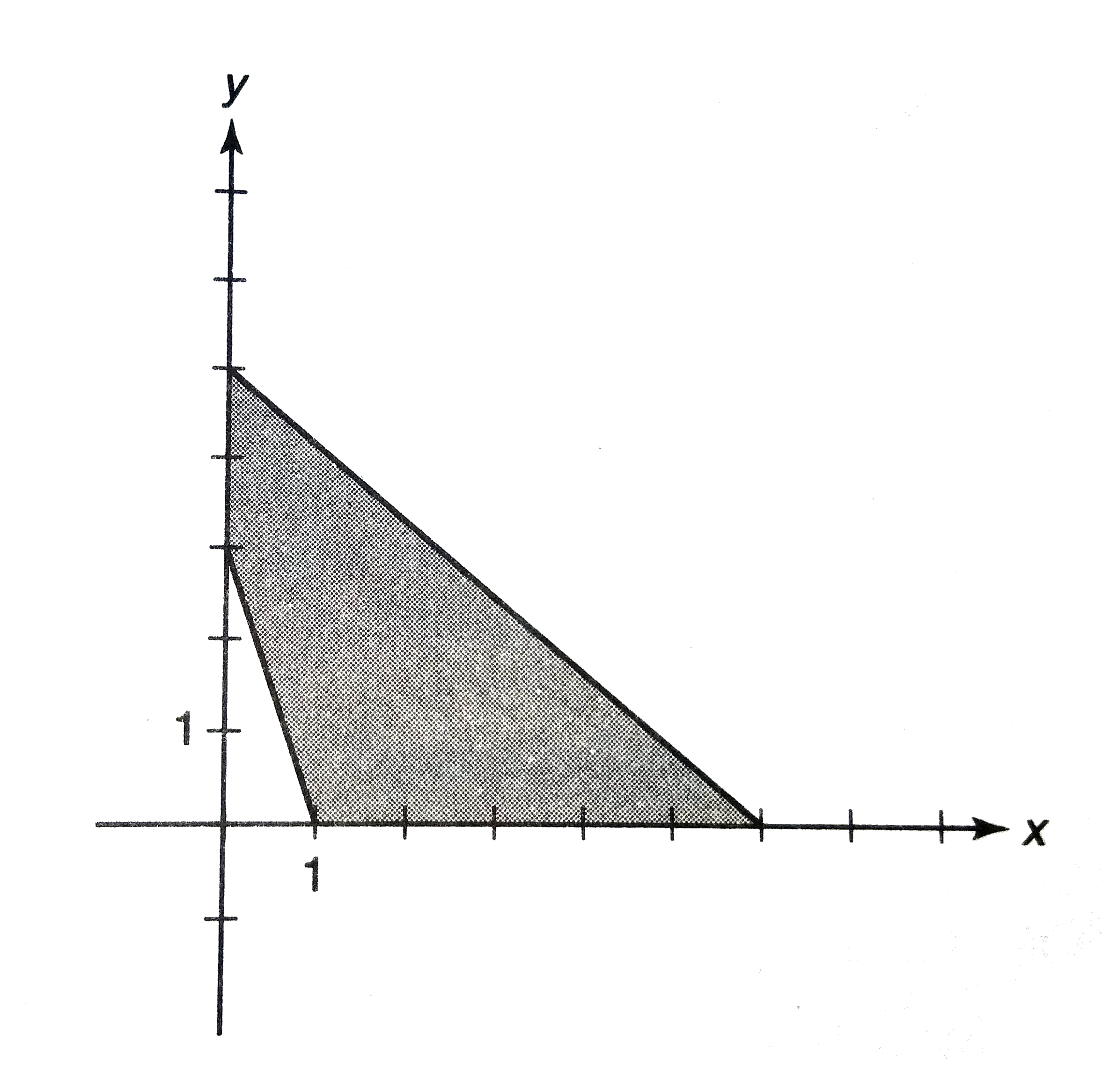
In the figure above, what is the number of square units in the area of the shaded region?
View SolutionLet A=[pqqp] such that det(A)=r where p,q,r all prime number, then trace of A is equal to
View SolutionIf the maximum value of the expression 15sec2θ−tan2θ+4cosec2θ is equal to pq (where p and q are coprime), then the value of (p+q) is
View SolutionIf the maximum value of the expression 15sec2θ−tan2θ+4cosec2θ is equal to pq (where p and q are coprime), then the value of (p+q) is
View Solution
