यदि Sn किसी समांतर श्रेणी के n पदों का योग है तो सिद्ध कीजिए कि S30=3(S20−S10)
Step by step video & image solution for यदि S_(n) किसी समांतर श्रेणी के n पदों का योग है तो सिद्ध कीजिए कि S_(30)=3(S_(20)-S_(10)) by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- समांतर श्रेणीBOOK - BALAJI PUBLICATIONCHAPTER - समांतर श्रेणीEXERCISE - बहुविकल्पीय प्रश्न
11 Videos
- वृत्तों से समबन्धित क्षेत्रफल BOOK - BALAJI PUBLICATIONCHAPTER - वृत्तों से समबन्धित क्षेत्रफल EXERCISE - स्वमूल्यांकन परीक्षण
15 Videos
- सांख्यिकी BOOK - BALAJI PUBLICATIONCHAPTER - सांख्यिकी EXERCISE - स्वमूल्यांकन परीक्षण
18 Videos
Similar Questions
यदि समांतर श्रेणी के n पदों का योग Sn प्रथम पद a तथा सार्वअंतर d=Sn−kSn−1 तब k=
View Solution6 सेमी त्रिज्या वाले वृत्त पर 60∘ पर त्रिज्यखण्ड का क्षेत्रफल व परिमाप ज्ञात कीजिए ।
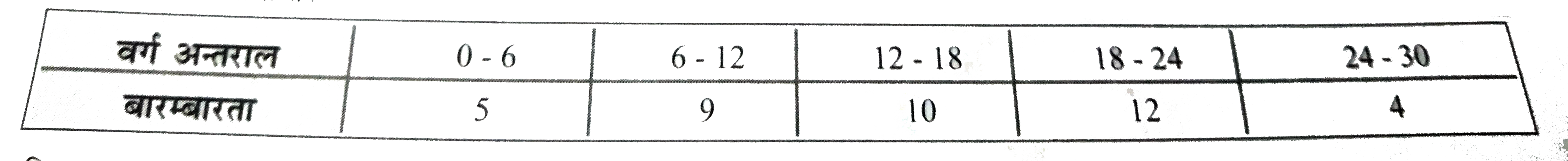
View Solutionइस बंटन का समांतर माध्य ज्ञात कीजिये ।
View SolutionLet Sn=1+2+3++n and Pn=S2S2−1⋅S3S3−1⋅S4S4−1⋅⋅⋅SnSn−1 Where n∈N,(n≥2). Then limx→∞Pn=______
View SolutionConsider the sequence of natural numbers s_(0),s_(1),s_(2),... such that s_(0)=3,s_(1)=3 and s_(n)=3+s_(n-1)s_(n-2) then
View SolutionS_(n)=sum_(n=1)^(n)(n)/(1+n^(2)+n^(4)) then S_(10)S_(20)
View SolutionIf Sn denotes the sum of the first n terms of an A.P., prove that S30=3(S20−S10) .
View Solutionयदि Sn एक A.P. के प्रथम n पदों के योग को निरूपित करता हैं तो सिद्ध कीजिए कि S30=3(S20−S10)
View Solutionमाना Sk=1+2+3+…+kk है। यदि S21+S22+….S210=512A है, तो A बराबर है
View Solutionसमांतर श्रेणी | समांतर श्रेणी का व्यापक पद | समांतर श्रेणी के N पदों का योगफल | उदाहरण
View Solution(1) किसी A.P. के प्रथम 10 पदों का योगफल ज्ञात कीजिए यदि T1=−14 और T5=20.
(ii) किसी A.P. का तीसरा पद 14 और 8वाँ पद 34 है।Sn और S30 ज्ञात कीजिए।
View SolutionIf Sn denotes the sum of n terms of a G.P., prove that: (S10−S20)2=S10(S30−S20)
View Solutionसमांतर श्रेणी के प्रथम N पदों का योग
View SolutionIf Sn denotes sum of the first n times of an A.P, then prove that S30=2[S20−S10.
View SolutionIf Sn denotes the sum of the first n terms of an A.P., prove that S30=3(S20−S10) .
View SolutionRevision|समांतर श्रेणी का n वां पद#!#समांतर श्रेणी के n पदों का योग
View Solution
