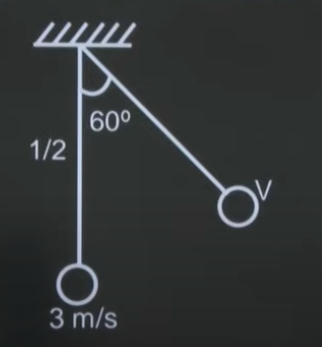
A pendulum bob has a speed 3m/s while passing thorugh its lowest position. What is its speed when it makes an angle of 600 with the vertical? The length of the pendulum is 0.5m Take g=10ms2.
To solve the problem, we will use the principle of conservation of mechanical energy. The total mechanical energy of the pendulum bob remains constant as it swings, assuming there are no non-conservative forces acting on it (like air resistance or friction).
1. Identify Initial and Final States:
- Initial state (lowest position): Speed v1=3m/s
- Final state (at 60∘ with the vertical): Speed v2 (unknown)
2. Determine the Length of the Pendulum:
- Given length l=0.5m
3. Calculate Initial Kinetic Energy (KE):
KE1=12mv21=12m(3)2=92m
4. Calculate Initial Potential Energy (PE):
- At the lowest position, the height h1=0, hence:
PE1=mgh1=mg(0)=0
5. Total Mechanical Energy at the Lowest Position:
E1=KE1+PE1=92m+0=92m
6. Calculate Final Potential Energy (PE) at 60∘:
- The height h2 when the pendulum makes an angle of 60∘ can be calculated using:
h2=l−lcos(60∘)=l(1−cos(60∘))=0.5(1−0.5)=0.5×0.5=0.25m
- Therefore, the final potential energy is:
PE2=mgh2=mg(0.25)
7. Calculate Final Kinetic Energy (KE):
- The total mechanical energy at this position is:
E2=KE2+PE2
- Since energy is conserved, E1=E2:
92m=KE2+mg(0.25)
8. Express KE in terms of v2:
KE2=12mv22
- Substitute into the energy conservation equation:
92m=12mv22+mg(0.25)
9. Cancel m from the equation:
92=12v22+g(0.25)
10. Substitute g=10m/s2:
92=12v22+10(0.25)
92=12v22+2.5
11. Simplify the equation:
92−2.5=12v22
92−52=12v22
42=12v22
2=12v22
12. Multiply both sides by 2:
4=v22
13. Take the square root:
v2=√4=2m/s
Final Answer:
The speed of the pendulum bob when it makes an angle of 60∘ with the vertical is 2m/s.
- Initial state (lowest position): Speed v1=3m/s
- Final state (at 60∘ with the vertical): Speed v2 (unknown)
- Given length l=0.5m
KE1=12mv21=12m(3)2=92m
- At the lowest position, the height h1=0, hence:
PE1=mgh1=mg(0)=0
E1=KE1+PE1=92m+0=92m
- The height h2 when the pendulum makes an angle of 60∘ can be calculated using:
h2=l−lcos(60∘)=l(1−cos(60∘))=0.5(1−0.5)=0.5×0.5=0.25m
- Therefore, the final potential energy is:
PE2=mgh2=mg(0.25)
- The total mechanical energy at this position is:
E2=KE2+PE2
- Since energy is conserved, E1=E2:
92m=KE2+mg(0.25)
KE2=12mv22
- Substitute into the energy conservation equation:
92m=12mv22+mg(0.25)
92=12v22+g(0.25)
92=12v22+10(0.25)
92=12v22+2.5
92−2.5=12v22
92−52=12v22
42=12v22
2=12v22
4=v22
v2=√4=2m/s
The speed of the pendulum bob when it makes an angle of 60∘ with the vertical is 2m/s.
Topper's Solved these Questions
- WORK AND ENERGYBOOK - HC VERMACHAPTER - WORK AND ENERGYEXERCISE - Worked Out Examples
14 Videos
- WORK AND ENERGYBOOK - HC VERMACHAPTER - WORK AND ENERGYEXERCISE - Short Answer
15 Videos
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMACHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Exercises
57 Videos
Similar Questions
The bob of a pendulum is moving with a speed of 5 m/s while passing through mean position . Calculate its speed at the position when it makes an angle of 30∘ with the vertical . The length of pendulum is 0.6 m / Use g=10m/s2
View SolutionA pendulum consists of a 2.0 kg stone swinging on a 4.5 m string of negligible mass. The stone has a speed of 8.0 m/s when it passes its lowest point. (a) What is the speed when the string is at 60∘ to the vertical ? (b) What is the greatest angle with the vertical that the string will reach during the stone's motion ? (c) If the potential energy of the pendulum-Earth system is taken to be zero at the stone's lowest point, what is the total mechanical energy of the system ?
View Solution
Knowledge Check
A pendulum bob has a speed of 3ms−1 at its lowest position. The pendulum is 0.5 m long. The speed of the bob, when string makes an angle of 60∘ to the vertical is (take, g=10ms−1)
A2ms−1B12ms−1C1ms−1D2.5ms−1A simple pendulum of length I and mass (bob) m is suspended vertically. The string makes an angle θ with the vertical. The restoring force acting on the pendulum is
AmgtanθB−mgsinθCmgsinθD−mgcosθA simple pendulum of length l and mass (bob) m is suspended vertically. The string makes an angle θ with the vertical. The restoring force acting on the pendulum is
AmgtanθB−mgsinθCmgsinθD−mgcosθ
A simple pendulum of length 1/2m has initial speed 3m/s when pendulum mass is at lowermost point. What will be the speed pf pendulum mass, when the string of pendulum makes an angle of 600 with vertical.
View SolutionA ball is projected vertically up with speed 20 m/s. Take g=10m/s2
View SolutionThe mass of a simple pendulum bob is 100 gm. The length of the pendulum is 1 m. The bob is drawn aside from the equilibrium position so that the string makes an angle of 60∘ with the vertical and let go. The kinetic energy of the bob while crossing its equilibrium position will be
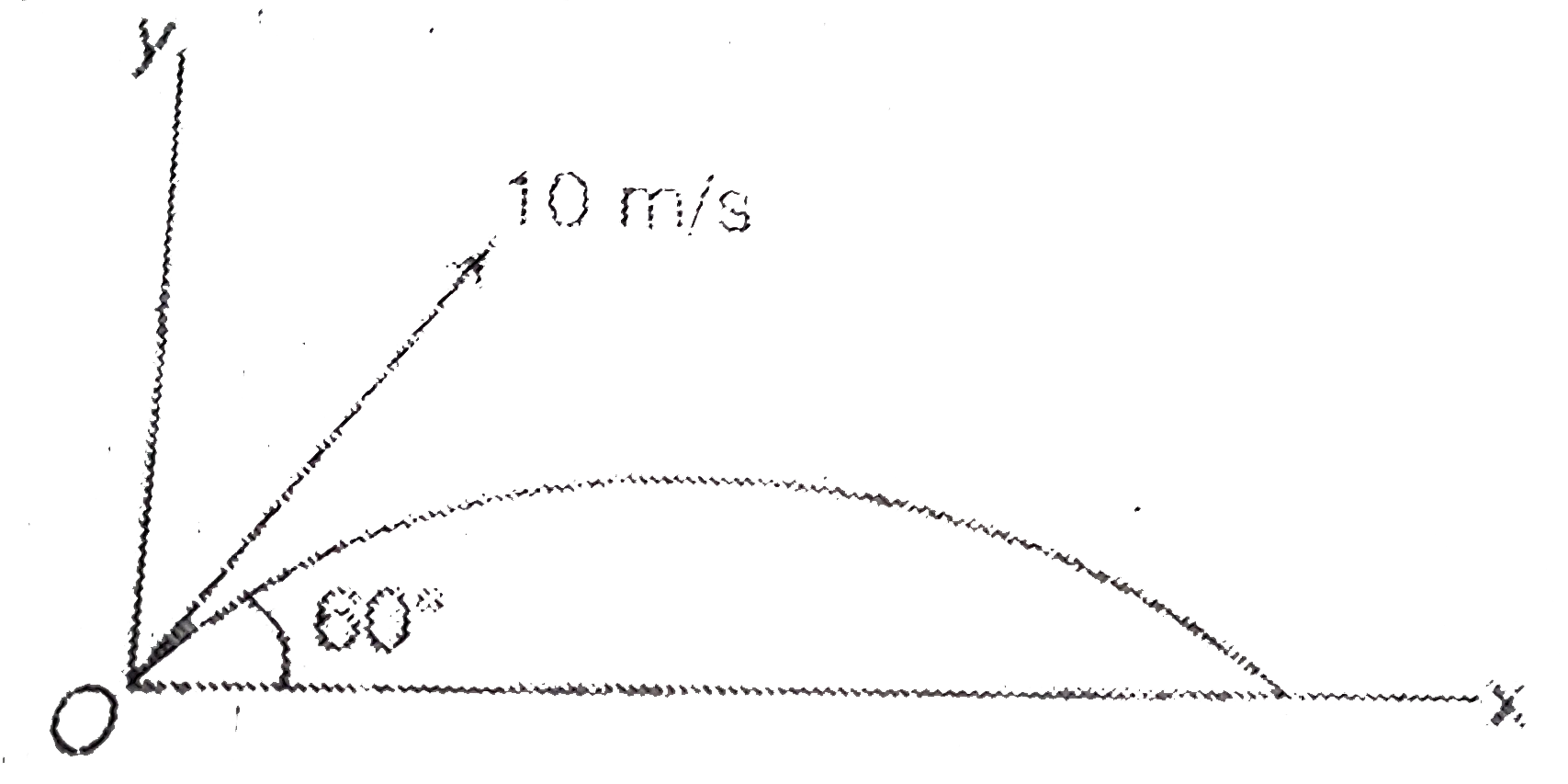
View SolutionA particle is projected at an angle 60∘ with the horizontal with a speed 10m/s. Then, latus rectum is (Take g=10m/s2)
View SolutionThe bob of a pendulum has its rest point 1 m below the support. The bob is pulled aside until the string makes an angle of 60∘ with the vertical line. On release with what speed does the bob swing pass its rest point?
View Solution
