A long string having a cross- sectional area 0.80mm2 and density 12.5gcm−3 is subjected to a tension of 64 N along the X-axis. One end of this string is attached to a vibrator moving in transverse direction at a frequency fo 20Hz. At t = 0. the source is at a maximujm displacement y= 1.0 cm. (a) Write the equation for the wave. (c ) What is the displacement of the particle of the string at x = 50 cm at time t = 0.05 s ? (d) What is the velocity of this particle at this instant ?
To solve the problem step by step, we will break it down into parts (a), (c), and (d) as per the question.
Given Data:
- Cross-sectional area A=0.80mm2=0.80×10−6m2
- Density ρ=12.5g/cm3=12.5×103kg/m3
- Tension T=64N
- Frequency f0=20Hz
- Maximum displacement y=1.0cm=0.01m
Step 1: Calculate the Linear Mass Density (μ)
The linear mass density μ is given by:
μ=ρ⋅A
Substituting the values:
μ=(12.5×103kg/m3)⋅(0.80×10−6m2)=10−5kg/m
Step 2: Calculate the Velocity of the Wave (v)
The velocity of the wave on the string is given by the formula:
v=√Tμ
Substituting the values:
v=√64N10−5kg/m=√6400000=80m/s
Step 3: Write the Wave Equation
The general form of the wave equation is:
y(x,t)=Acos(ωt−kx)
Where:
- A=0.01m (maximum displacement)
- ω=2πf=2π×20=40πrad/s
- The wave number k is given by k=ωv=40π80=π2rad/m
Thus, the wave equation becomes:
y(x,t)=0.01cos(40πt−π2x)
Step 4: Calculate the Displacement at x=50cm and t=0.05s
Convert x to meters:
x=50cm=0.50m
Substituting x and t into the wave equation:
y(0.50,0.05)=0.01cos(40π×0.05−π2×0.50)
Calculating the arguments:
y(0.50,0.05)=0.01cos(2π−π4)=0.01cos(7π4)=0.01⋅1√2≈0.00707m=0.707cm
Step 5: Calculate the Velocity of the Particle at this Instant
To find the velocity, we differentiate the wave equation with respect to time:
vy=∂y∂t=−Aωsin(ωt−kx)
Substituting the values:
vy=−0.01⋅40πsin(40π×0.05−π2×0.50)
Calculating:
vy=−0.01⋅40πsin(2π−π4)=−0.01⋅40π⋅sin(7π4)=−0.01⋅40π⋅(−1√2)
Thus:
vy≈0.01⋅40π⋅1√2≈0.01⋅40⋅3.14⋅0.707≈0.89m/s
Final Answers:
(a) The wave equation is:
y(x,t)=0.01cos(40πt−π2x)
(c) The displacement of the particle at x=50cm and t=0.05s is approximately 0.707cm.
(d) The velocity of this particle at this instant is approximately 0.89m/s.
- Cross-sectional area A=0.80mm2=0.80×10−6m2
- Density ρ=12.5g/cm3=12.5×103kg/m3
- Tension T=64N
- Frequency f0=20Hz
- Maximum displacement y=1.0cm=0.01m
The linear mass density μ is given by:
μ=ρ⋅A
Substituting the values:
μ=(12.5×103kg/m3)⋅(0.80×10−6m2)=10−5kg/m
The velocity of the wave on the string is given by the formula:
v=√Tμ
Substituting the values:
v=√64N10−5kg/m=√6400000=80m/s
The general form of the wave equation is:
y(x,t)=Acos(ωt−kx)
Where:
- A=0.01m (maximum displacement)
- ω=2πf=2π×20=40πrad/s
- The wave number k is given by k=ωv=40π80=π2rad/m
y(x,t)=0.01cos(40πt−π2x)
Convert x to meters:
x=50cm=0.50m
Substituting x and t into the wave equation:
y(0.50,0.05)=0.01cos(40π×0.05−π2×0.50)
Calculating the arguments:
y(0.50,0.05)=0.01cos(2π−π4)=0.01cos(7π4)=0.01⋅1√2≈0.00707m=0.707cm
To find the velocity, we differentiate the wave equation with respect to time:
vy=∂y∂t=−Aωsin(ωt−kx)
Substituting the values:
vy=−0.01⋅40πsin(40π×0.05−π2×0.50)
Calculating:
vy=−0.01⋅40πsin(2π−π4)=−0.01⋅40π⋅sin(7π4)=−0.01⋅40π⋅(−1√2)
Thus:
vy≈0.01⋅40π⋅1√2≈0.01⋅40⋅3.14⋅0.707≈0.89m/s
(a) The wave equation is:
y(x,t)=0.01cos(40πt−π2x)
(c) The displacement of the particle at x=50cm and t=0.05s is approximately 0.707cm.
(d) The velocity of this particle at this instant is approximately 0.89m/s.
Topper's Solved these Questions
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMACHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Short Answer
7 Videos
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMACHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Objective 1
22 Videos
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMACHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Exercises
57 Videos
- THE FORCESBOOK - HC VERMACHAPTER - THE FORCESEXERCISE - Exercises
12 Videos
- WORK AND ENERGYBOOK - HC VERMACHAPTER - WORK AND ENERGYEXERCISE - Exercises
64 Videos
Similar Questions
A uniform string of length 6.5m is subjected to a tension of 40N. Mass of the string is 162.5g. One end of the string is fixed and the other end is tied to a source (s). which produces a transverse oscillation. The displacement of the end of the string tied to the source can be expressed as y=(3mm)sin(40πt), where ‘t’ is time. Find the displacement of point P of the string at a distance of 3.75m from the fixed end, at time t=0.3s.

View SolutionA String on a cello vibrates in its first normal mode with a frequency of 220Hz. The vibrating segment is 70.0cm long and has a mass of 1.20g . (a) Find the tension in the string . (b) Determine the frequency of vibration when the string vibrates in three segments .
View Solution
Knowledge Check
A transverse sinusoidal wave of wavelength 20 cm is moving along a string towards increasing x. the transverse displacement of the string particle at x=0 as a function of time is shown in figure.
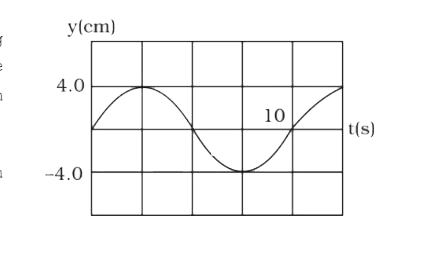
Q. The transverse velocity of the particle at x=0 at t=5.0 s is ?A−2cm/sB+2cm/sC+1.5cm/sD−2.5cm/sThe displacement of a vibrating string versus position along the string is shown in the figure. The periodic wave have a speed of 10.0cm/s. A and B are two points on the string.
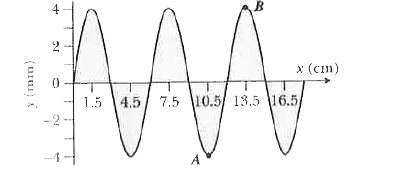
What is the wavelength of the wave?A3.0 cmB6.0 cmC9.0 cmD12 cmThe displacement of a vibrating string versus position along the string is shown in the figure. The periodic wave have a speed of 10.0cm/s. A and B are two points on the string.
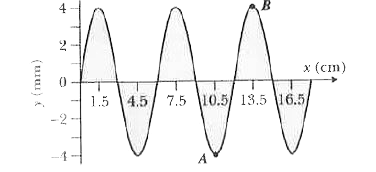
What is the frequency of the wave?A0.60HzB0.90HzC1.7HzD1.3Hz
A string 1 m long with mass 0.1 g cm−1 is under a tension of 400 N.Find is fundamental frequency of vibration.
View SolutionThe equation for a wave travelling in x-direction 0n a string is
y=(3.0cm)sin[(3.14cm−1x−(314s−1t]
(a) Find the maximum velocity of a particle of the string.
(b) Find the acceleration of a particle at x=6.0cm at time t=0.11s
View SolutionThe equation for a wave travelling in x-direction on a string is y =(3.0cm)sin[(3.14 cm^(-1) x - (314s^(-1))t] (a) Find the maximum velocity of a particle of the string. (b) Find the acceleration of a particle at x =6.0 cm at time t = 0.11 s.
View SolutionThe displacement of a vibrating string versus position along the string is shown in the figure. The periodic wave have a speed of 10.0cm/s. A and B are two points on the string.
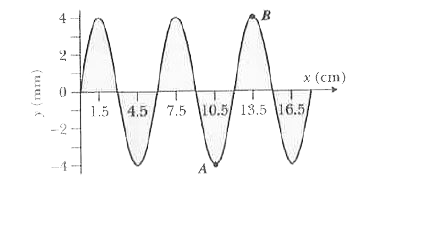
What is the amplitude of the wave?
View SolutionA string of length 0.5m and linear density 10−4 kg/m is stretched under a tension of 100 N. If the frequency of the vibration of the string is 4000 Hz, how many loops are formed on the string?
View Solution
