In a vertical circle the minimum or critical velocity at highest point of path will be
√2rg
√rg
rω
zero
The correct Answer is:B
To find the minimum or critical velocity at the highest point of an object moving in a vertical circle, we can follow these steps:
1. Understanding Forces at the Highest Point:
At the highest point of the circular path, two forces act on the object: the gravitational force (mg, acting downward) and the tension in the string (T, also acting downward if the string is taut).
2. Applying Centripetal Force Equation:
The centripetal force required to keep the object moving in a circle is provided by the net force acting towards the center of the circle. Therefore, we can write the equation:
T+mg=mv2r
where:
- m is the mass of the object,
- v is the velocity at the highest point,
- r is the radius of the circular path.
3. Finding Minimum Velocity:
To find the minimum velocity at the highest point, we need to consider the case where the tension T is zero. This is because the minimum velocity occurs when the object is just about to lose contact with the circular path. Thus, we set T=0:
0+mg=mv2r
4. Simplifying the Equation:
By simplifying the equation, we can cancel m from both sides (assuming m≠0):
mg=mv2r
This simplifies to:
g=v2r
5. Solving for Velocity:
Rearranging the equation to solve for v:
v2=rg
Taking the square root of both sides gives us the critical velocity:
v=√rg
Final Answer:
The minimum or critical velocity at the highest point of the path is:
v=√rg
---
At the highest point of the circular path, two forces act on the object: the gravitational force (mg, acting downward) and the tension in the string (T, also acting downward if the string is taut).
The centripetal force required to keep the object moving in a circle is provided by the net force acting towards the center of the circle. Therefore, we can write the equation:
T+mg=mv2r
where:
- m is the mass of the object,
- v is the velocity at the highest point,
- r is the radius of the circular path.
To find the minimum velocity at the highest point, we need to consider the case where the tension T is zero. This is because the minimum velocity occurs when the object is just about to lose contact with the circular path. Thus, we set T=0:
0+mg=mv2r
By simplifying the equation, we can cancel m from both sides (assuming m≠0):
mg=mv2r
This simplifies to:
g=v2r
Rearranging the equation to solve for v:
v2=rg
Taking the square root of both sides gives us the critical velocity:
v=√rg
The minimum or critical velocity at the highest point of the path is:
v=√rg
Topper's Solved these Questions
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - PRACTICE SET 03EXERCISE - PAPER 2 (MATHEMATICS)1videos
PRACTICE SET 03
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - PRACTICE SET 02EXERCISE - Paper 1 (Physics)50videos
PRACTICE SET 02
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - PRACTICE SET 04EXERCISE - PAPER 1 (PHYSICS & CHEMISTRY)50videos
PRACTICE SET 04
Similar Questions
A stone of mass 5kg, tied to one end of a rope of length 0.8m, is whirled in vertical circle. Find the minimum velocity at the highest point and at the midway point [g=9.8m/s2]
View SolutionA stone of mass 100 g attached to a string of length 50 cm is whirled in a vertical circle by giving it a velocity of 7 m/s at the lowest point. Find the velocity at the highest point .
View Solution
Knowledge Check
- Question 1 - Select One
A particle of mass m attached to an inextensible light string is moving in a vertical circle of radius r. The critical velocity at the highest point is v0 to complete the vertical circle. The tension in the string when it becomes horizontal is
A3mv20rB9mv20rC3mgDboth (A) and (C) are correct - Question 2 - Select One
A fighter plane is moving in a vertical circle of radius 'r'. Its minimum velocity at the highest point of the circle will be
A√3grB√2grC√grD√gr/2 - Question 3 - Select One
A fighter aircraft is looping in a vertical plane. The minimum velocity at the highest point is (given r = radius of the loop)
A√12grB√2grC√grD√3gr
A bob is attached to a string of length l whose other end is fixed and is given horizontal velocity at the lowest point of the circle so that the bob moves in a vertical plane. Match the velocity given at the lowest point of circle in column I with tension and velocity at the highest point of the circle corresponding to velocity of column I of column II
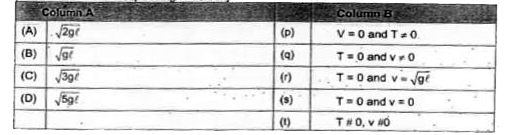 View Solution
View SolutionA body of mass 100 gram is tied at the end of a string of length 1m. It is rotated in a vertical circle at a critical speed of 4 m/s at the highest point . The tension in the string at the huighest point in its path is [ take g=10m/s2]
View SolutionA body is tied up a string of length l and rotated in vertical circle at minimum speed. When it reaches at highest point string breaks and body moves on a parabolic path in presence of gravity according to fig. in the plane of point A, value of horizontal range AC will be
 View Solution
View SolutionIn a circus stuntman rides a motorbike in a circular track of radius R in the vertical plane. The minimum speed at highest point of track will be
View SolutionAn, aeroplane , flying in the sky, suddenly starts revolving in a vertical circle of radius 4 km. At the highest point of the circle , the pilot experiences weightlessness. Its velocity at the highest point will be
View Solution

