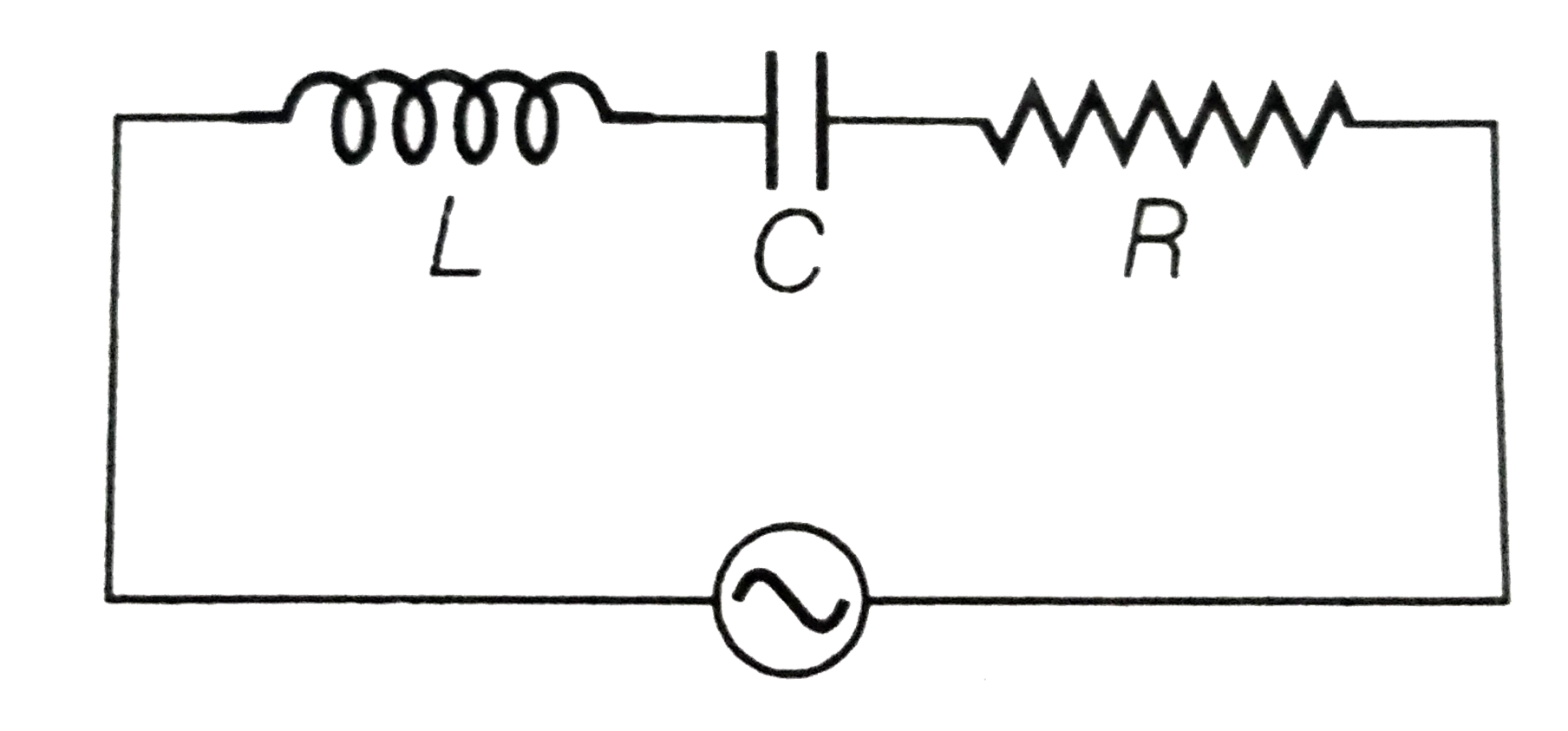
A 100V, aC source of frequency of 500Hz is connected to an L-C-LR circuit with L=8.1mH,C=12.5μF,R=10Ω all connected in series as shown in figure. What is the quality factor of circuit?
2.02
2.5434
20.54
200.54
The correct Answer is:B
Q-factor =1LR√LC=ωrLR
Here L=8.1mH,
C=105μF,R=10Ω and
f=500Hz
∴Q=ωrLR=2πfLR
=2×π×500×8.1×10−310=8.1π10
=2.5434
Topper's Solved these Questions
Similar Questions
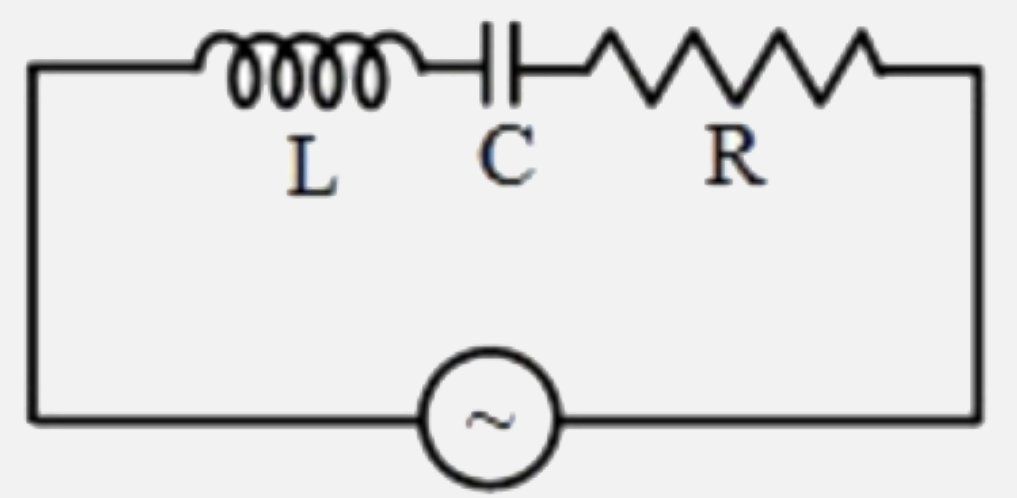
A 100 V, AC source of frequency 500 Hz is connected to an LCR circuit with L=8.1mH, C=12.5μF,R=10Ω all connected in series as shown in the figure. What is the quality factor of the circuit?
 View Solution
View SolutionA 100 V AC source of frequency 500 Hz is connected to LCR circuit with L=8.1mH, C=12.5μF and R=100Ω, all connected in series. Find the potential (in volt) across the resistance.
View Solution
Knowledge Check
- Question 1 - Select One
A 100 V AC source of frequency 500 Hz is connected to a LCR circuit with L= 8.1 mH, C=12.5μF and R=10Ω, all connected in series. The potential difference across the resistance is
A100 VB200 VC300 VD400 V - Question 2 - Select One
A 100V a.c. source of frequency 50Hz is connected to a LCR circuit with L=8.1 millihenry, C=12.5μF and R=10 ohm, all connected in series. What is the potential difference across the resistance?
A100VB200VC300VD450V - Question 3 - Select One
A 100 volt AC source of angular frequency 500 rad/s is connected to a LCR circuit with L = 0.8 H, C=5muF and R = 10Omega, all connected in series. The potential difference across the resistance is
A100√2voltB100voltC50voltD50√3
In series L-C-R circuit the quality factor is
View SolutionIn series L-C-R circuit the quality factor is
View SolutionA 100 volt AC source of angular frequency 500 rad/s is connected to a LCR circuit with L = 0.8 H, C=5muF and R = 10Omega, all connected in series. The potential difference across the resistance is
View SolutionThe resonant frequency of a series LCR circuit with L=2.0H,C=32μF and R=10Ω is
View SolutionFor an alternating current of frequency 500π Hz in L-C-R series circuit with L = 1H, C = 1μF,R=100Ω, impedance is-
View Solution

