The statement p∨~p is
A
lautology
B
contradiction
C
neigther a lautology nor a contradiction
D
None of the above
Video Solution
Text Solution
The correct Answer is:A
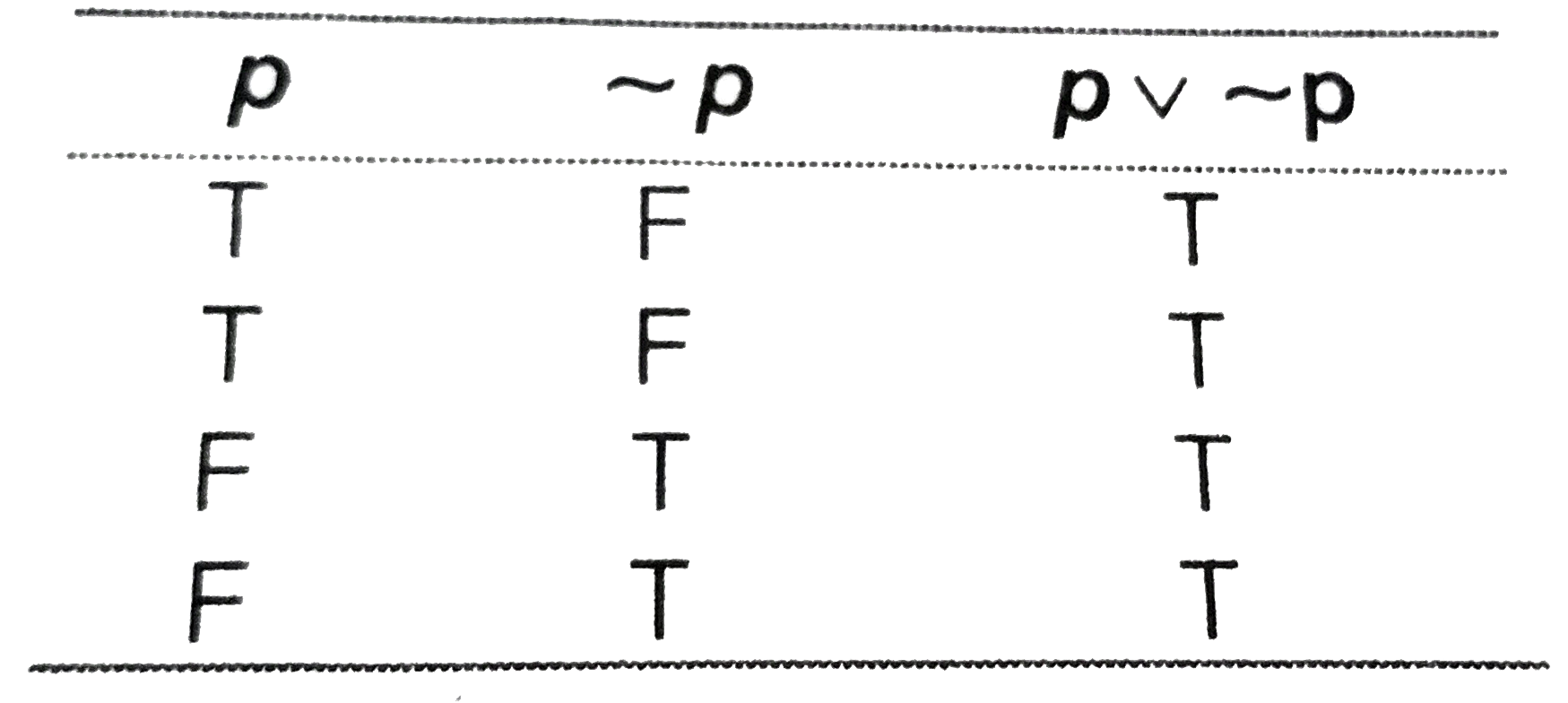
It is clear from the above table that given statement is a tautology.
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The negation of the statement(~p∨~q) is
Ap→qBp∨qC~p∧~qDp∧q - Question 2 - Select One
For two statements p and q, the statement ~(p∨(~q)) is equivalent to
A~p∨qB~p∧qC~p∨~qD~p∧~q - Question 3 - Select One
The statement (p⇒p)∧(~p⇒p) is a-
ATautology and contradictionBNeither tautology nor contradicationCContradictionDTautology
The negative of the statement ~p∨(p∧q) is
View SolutionThe negation of the statement (~p∨~q)∨(p∧~q) is
View SolutionThe dual of the statement (p∧q)∨~q=p∨~q is
View SolutionWhich of the following is true?
(i) If p is a statement then ~p is not a statement
(ii) If p is a statement then ~p is also a statement
(iii) Negation of p : 0 is a positive number is, 0 is a negative numberView SolutionWhich of the following is true?
(i) If p is a statement then ~p is not a statement
(ii) If p is a statement then ~p is also a statement
(iii) Negation of ''p:x is a positive real number'' is , ''x is a negative real number''View Solution

