The area bounded by the curve x=2−y−y2 and Y-axis is
A
92 sq unit
B
23 sq unit
C
84 sq units
D
53 sq units
Video Solution
Text Solution
The correct Answer is:A
At x = 0, 2−y−y2=0
⇒y2+y−2=0
⇒y2+2y−y−2=0
⇒y(y+2)−(y+2)=0
⇒(y+2)(y−1)=0
implies y=-2,1
Therefore, the curve intersects y-axis at (0, 1) and (0, -2)
The required area =∫1−2xdy
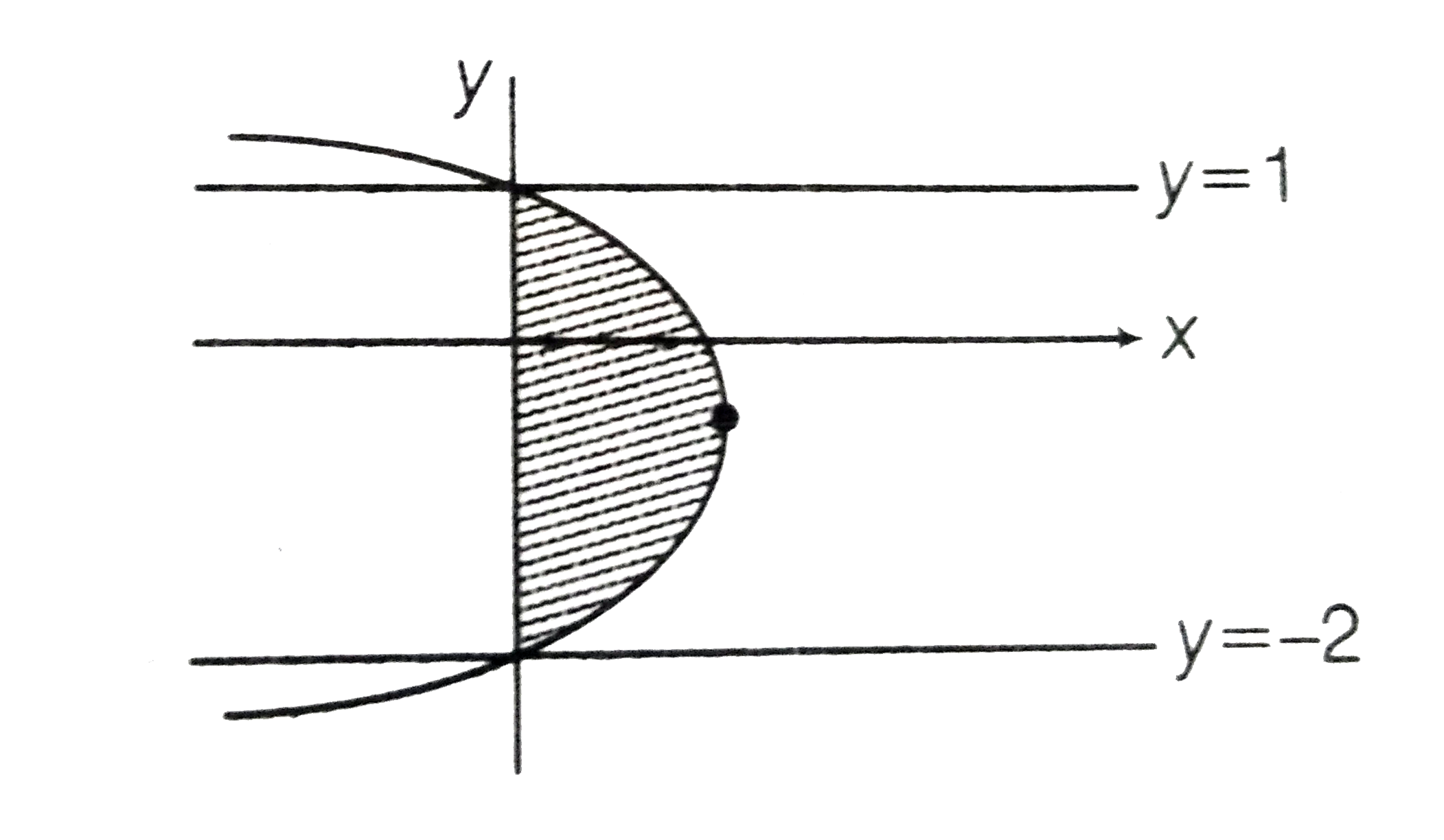
=∫1−2(2−y−y2)dy=[2y−y22−y33]1−2=92 sq units
|
Topper's Solved these Questions
- APPLICATIONS OF DEFINITE INTEGRALS BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DEFINITE INTEGRALS EXERCISE - Exercise 2
34 Videos
- APPLICATIONS OF DEFINITE INTEGRALS BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DEFINITE INTEGRALS EXERCISE - MHT CET Corner
6 Videos
- APPLICATIONS OF DERIVATIVESBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - MHT CET CORNER
21 Videos
Similar Questions
Knowledge Check
The area bounded by the curve x=4−y2 and the Y-axis is
A16 sq unitsB32 sq unitsC323 sq unitsD163 sq unitsThe area bounded by the curve y=x(x2−1) and x-axis is
A0B12C1D14Area bounded by the curve y=x2+x−6 and the X-axis is
A1276B2256C1256DNone of these
The area bounded by the curve y2=4ax, Y-axis between y=-a and y= a is
View SolutionThe area bounded by the curves y2=x3and|y|=2x.
View Solutionthe area included between the curve xy2=a2(a−x) and y -axis is -
View SolutionThe area bounded by the curve |x|+|y|=1 and axis of x, is given by
View SolutionThe area of the region bounded by the curves x=y2−2 and x=y is
View Solution
