The minimum value of $z = 10 x + 25 y$ subject to

A

$0 \leq x \leq 3, 0 \leq y \leq 3, x + y \geq 5$ is

B

80

C

95

D

105

Text Solution

Verified by Experts

The correct Answer is:A

Given Z=10x+25y
and subject to $0 \leq x \leq 3, 0 \leq y \leq 3, x + y \geq 5$
On taking given constraints as equation, we get the following graph

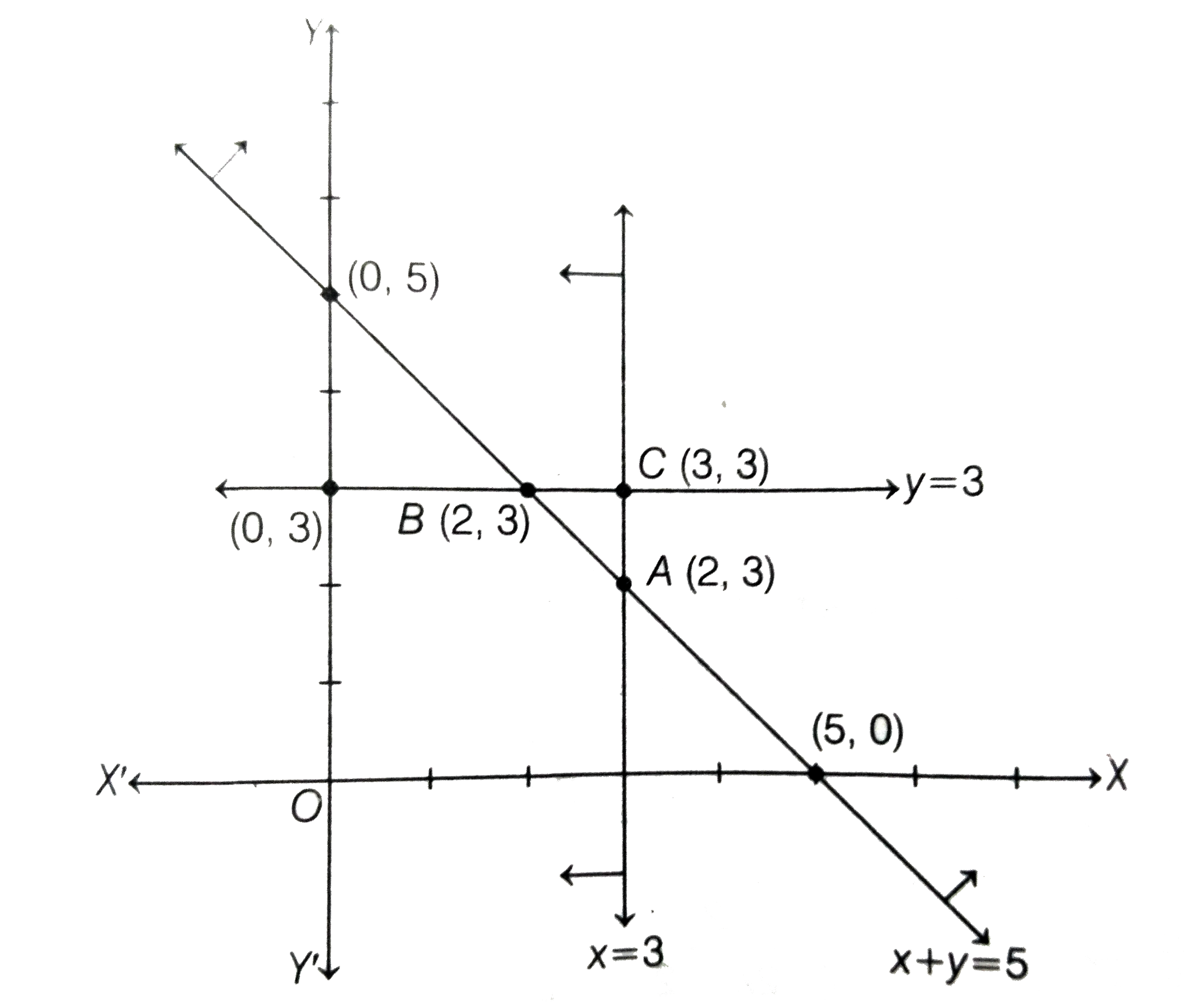
here, intersecting point of lines x=3 and x+y=5 is A(3,2), intersecting point of lines y=3 and x+y=5 is B(2,3) and intersecting point of lines, x=3 and y=3 is C(3,3). Here, ABCA is the feasible region whose corner points are A(3,2), B(2,3) and C(3,3)
$(Corner points, Z=10x+25y), (A (3, 2), 10 \times 3 + 25 \times 2 = 80 (minimum)), (B (2, 3), 10 \times 2 + 25 \times 3 = 95), (C, 3), 10 \times 3 + 25 \times 3 = 105) :}$

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The minimum value of Z = 10x + By subject to $4 x + y \geq 4, x + 3 y \geq 6, x + y \geq 3, x \geq 0, y \geq 0$ is
A60
B27
C $\frac{74}{3}$
D32
Question 2 - Select One
The point at which the minimum value of $z = 8 x + 12 y$ subject to the constraints $2 x + y \geq 8, x + 2 y \geq 10 x \geq 0, y \geq 0$ is obtained is
A(8,0)
B(9, 1)
C(2, 4)
D(10, 0)
Question 3 - Select One
The minimum value of $z = 20 x + 9 y$ subject to $2 x + y \geq 36, 6 x + y \geq 60, x \geq 0, y \geq 0$ is
A330
B336
C360
D333

MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS-SOLVED PAPER 2019-MCQS

Home
Ask Doubt
Profile