For L.P.P, maximize z=4x1+2x2 subject to
A
3x1+2x2≥9,x1−x2≤3,x1≥0,x2≥0 has . . .
B
infinite number of optimal solutions
C
unbounded solution
D
no solution
Text Solution
The correct Answer is:B
We have, maximise z=4x1+2x2
subject to constracts, 3x1+2x2≥9,x1−x2≤3,x1≥0,x2≥0
On taking given constraints as equation, we get the following graphs
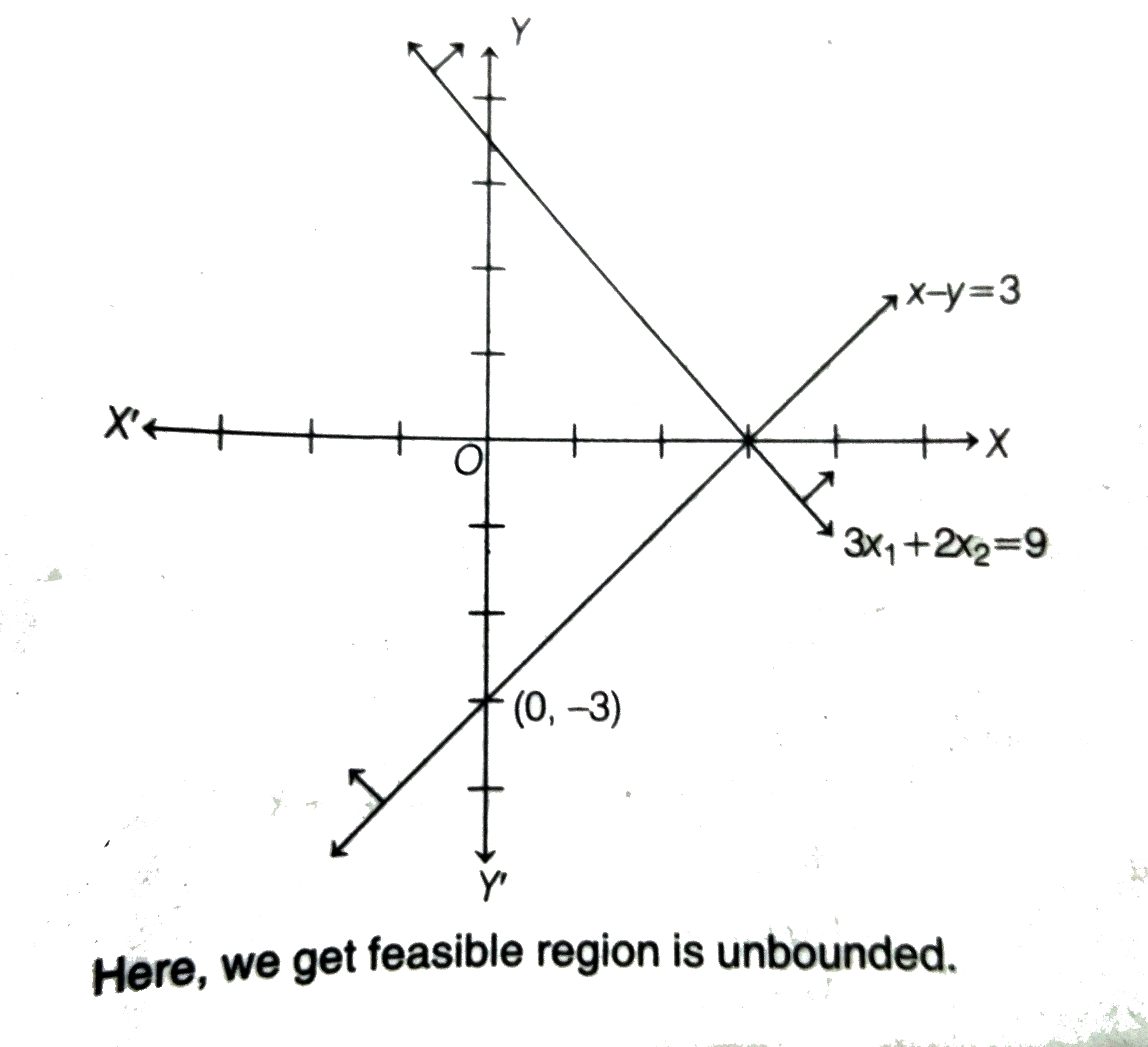
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
For the LPP , maximize z = x + 4y subject to the constraints x+2y≤2,x+2y≥8,x,y≥0
Azmax=4Bzmax=8Czmax=16Dhas no feasible solution - Question 2 - Select One
For the L.P.P. :
Maximize Z=x+y
subject to x+y≤1,2x+2y≥6,x≥0,y≥0,
the number of feasible solutions isA1B2C0Dinfinite - Question 3 - Select One
For the L.P.P. :
Maximize Z=5x+3y
subject to 3x+5y≤15,5x+2y≤10,x≥0,y≥0,
the number of feasible solutions (points) isA1B3C4Dinfinitely many
Solve the L.P.P graphically:
Maximize Z=x+2y
subject to constraints ,
x+2y≥100
2x−y≤0
2x+y≤200
x,y≥0View SolutionSolve the following linear programming problem (L.P.P) graphically Minimize: Z=2x+y subject to constraints 2x+3y≤12;x+y≤5;x≥2;x≥0;y≥0
View SolutionThe L.P.P. :
Maximize Z=4x−10y
subject to 2x−y≤2,x−4y≥4,x≥0,y≥0 hasView SolutionOptimum solution of the L.P.P. :
Maximize z=3x+5y
subject to 3x+2y≤18,x≤4,y≤6,x≥0,y≥0 isView SolutionGiven A≡(3,2),B≡(4,0),C≡(1,1)andD≡(0,4).
Then, the optimal solution of the L.P.P.
Maximize Z=38x+19y
subject to 2x+3y≤12,2x+y≤8,x≥0,y≥0
takes place at the pointsView Solution

