A horse is tethered to one corner of a square plot of side 42 m by a 30 m long rope, then
(i) Find the area it can graze
(ii) Find the area that will be left ungrazed.
The correct Answer is:(i)
To solve the problem step by step, we will follow these calculations:
Step 1: Understand the Problem
A horse is tethered to one corner of a square plot with a side length of 42 m using a rope that is 30 m long. We need to find:
(i) The area the horse can graze.
(ii) The area that will be left ungrazed.
Step 2: Calculate the Area the Horse Can Graze
The horse can graze in a quarter-circle area because it is tethered at one corner of the square. The radius of this quarter-circle is the length of the rope, which is 30 m.
Area of a quarter-circle is given by the formula:
Area=14πr2
Where r=30 m.
Substituting the value of r:
Area=14×227×(30)2
Calculating (30)2=900:
Area=14×227×900
=22×9004×7
=1980028
=707.14 m2
Step 3: Calculate the Total Area of the Square Plot
The area of the square plot is calculated using the formula:
Area of square=side2
Where the side length is 42 m:
Area of square=422=1764 m2
Step 4: Calculate the Ungrazed Area
To find the area that will be left ungrazed, we subtract the grazed area from the total area of the square:
Ungrazed Area=Total Area−Grazed Area
Substituting the values we calculated:
Ungrazed Area=1764−707.14
=1056.86 m2
Final Answers
(i) The area the horse can graze is 707.14 m².
(ii) The area that will be left ungrazed is 1056.86 m².
---
A horse is tethered to one corner of a square plot with a side length of 42 m using a rope that is 30 m long. We need to find:
(i) The area the horse can graze.
(ii) The area that will be left ungrazed.
The horse can graze in a quarter-circle area because it is tethered at one corner of the square. The radius of this quarter-circle is the length of the rope, which is 30 m.
Area=14πr2
Where r=30 m.
Area=14×227×(30)2
Calculating (30)2=900:
Area=14×227×900
=22×9004×7
=1980028
=707.14 m2
The area of the square plot is calculated using the formula:
Area of square=side2
Where the side length is 42 m:
Area of square=422=1764 m2
To find the area that will be left ungrazed, we subtract the grazed area from the total area of the square:
Ungrazed Area=Total Area−Grazed Area
Substituting the values we calculated:
Ungrazed Area=1764−707.14
=1056.86 m2
(i) The area the horse can graze is 707.14 m².
(ii) The area that will be left ungrazed is 1056.86 m².
Topper's Solved these Questions
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MENSURATIONEXERCISE - Assignment 8.58videos
MENSURATION
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MENSURATIONEXERCISE - Assignment 8.311videos
MENSURATION
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - 4 Marks Questions18videos
LINEAR EQUATIONS IN TWO VARIABLES
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MODEL QUESTION PAPEREXERCISE - SOLVE THE FOLLOWING SUBQUESTIONS: (ANY ONE)2videos
MODEL QUESTION PAPER
Similar Questions
Knowledge Check
- Question 1 - Select One
Four horses are tethered at four corners of a square plot of 42 m so that they just cannot reach one another . The area left ungrazed is :
A378m2B438m2C786m2Dnone of these - Question 2 - Select One
Four horses are tethered at four corners of a square plot of 42 m so that they just cannot reach one another . The area left un grazed is :
A378m2B438m2C786m2Dnone of these
A horse is tethered to a corner of a rectangular field 80 m by 50 m by a rope of length 7 m . Find the area over which it can graze . Also find the area beyond his reach .
View SolutionA horse is tethered to one corner of a rectangular field, 60 m by 40 m, by a rope 14 m long. On how much area can the horse graze?
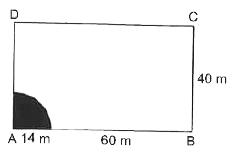 View Solution
View SolutionFour horses are tethered at four corners of a square plot of side 63 metres so that they just reach one another. Find the area left ungrazed inside the plot.
View SolutionA horse is tied to a peg at one corner of a square shaped grass field of side 15 m by means of a 5 m long rope (see the given figure). Find
The increase in the grazing area if the rope were 10 m long instead of 5 m.
[Use π=3.14 ]View SolutionA horse is tied to a peg at one corner of a square shaped grass field of side 15 m by means of a 5 m long rope (see the given figure). Find

The area of that part of the field in which the horse can graze.
[Use π=3.14 ]View SolutionFour horses are tethered at four corners of a square plot of side 63 m, so that they just cannot reach one another. The area left ungrazed is
View Solution

